5. zur Theorie

1
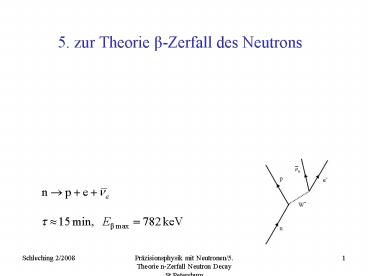
5. zur Theorie ß-Zerfall des Neutrons
2
V-A weak interaction
3
1. universality and 2. CVC
1. Universality GF/v2 Gµ Gt e and
g-charge universality is postulated in Standard
Model, is required in Grand Unification. 2.
Conservation of weak hadronic Vector Current
CVC hadronic vector coupling 1 i.e.
hadronic vector current Vµ weak g(p ?µ n)
is conserved, like hadronic el.-magn. current
Vµ el.-m. e(p ?µ p) is conserved. is
required in electro-weak Standard Model
4
CVC strong isospin conservation
5
3. PCAC

• ? gA/gV 1.27
• axial vector current Aµ is not conserved ?µ Aµ
  ? 0
• old version (40 yrs)
• pion decay ?-? µ- ?µ' is axial decay,
• has ?µAµ f? m?2, with small m?
• ? 3. Partial Conservation of Axial-vector Current
• applied to neutron decay, this gives
• GoldbergerTreiman relation mN gA f? g?N
• good to 10

6
chiral symmetry

• "new" version (20 yrs)
• if gA/gV 1, then axial hadronic current is
  conserved ?µ Aµ 0,
• the underlying (global) symmetry is the
• chiral symmetry of the strong interaction
• N' exp(-i ?½t ?5) N leaves Lagrangean L
  invariant
• Chiral symmetry is left-right symmetric SU(2)L
  SU(2)R.
• "L" and "R" can be defined only for massless
  particles,
• but nucleons are massive, and as gA/gV ? 1
• i.e. chiral symmetry is not a good symmetry.
• however gA/gV is nearly 1 There is a chiral
  symmetry, but it is
• spontaneously broken SU(2)L SU(2)R ? SU(2)iso
  transition
• (probably identical with quark-gluon phase
  transition).

7
example s-model

• massless fermions, coupling g to
• pions ? (pseudoscalar, isotriplet)
• and to s (scalar, isosinglet)
• plus quartic terms in ?, s
• spontaneous symmetry breaking of chiral symmetry
• fermion mass generation mN f g
• pions ? Goldstones with m? 0
• make ?'s massive by explicit symmetry breaking
  term in L
• then follows automatically ?µAµ f m?2,
  i.e. f f? ,
• and mN gA f? g?N
• GoldbergerTreiman relation

?
8
predictions for gA/gV

• gA enters many other processes
• p-N scattering (Adler-Weisberger relation)
• hyperon decay (current algebra relations)
• parton model (Björken, Ellis-Jaffe sum rules)
• Models
• spin-flavor content of constituent quarks
  gA/gV5/3
• constituent quarks in "bag"-potential
• gA/gV5/3radial integral5/30.651.09
• QCD calculations on the lattice
• (lattice constant a)

?exp. gA/gV
9
4. Weak magnetism

• Postulated before advent of Standard Model
• Isovector of hadronic weak current t, t-
• isovector portion of hadronic el.-magn. current
  t0
• isospin triplet (t, t0, t-) of conserved
  currents.

10
measurement of weak magnetism

• either from ß-decay asymmetry spectrum (
  1-effect)
• Problem statistics, undetected background
• or from ß-decay difference spectrum (background
  free)
• Problem statistics, detector function
• Today 1s-effect

11
2. Short history of CKM matrixa) 60ies
Suppression of strangeness-changing decays

• 1963 3 quark flavors known up u
• down d
• strange s
• Observation Strangeness changing decays of K,
  ?, (?S1)
• are suppressed by a factor 20 (w.r.t. ?S0)

weak decay examples quark description rate
14O (0? 0) u ? d e ?e' Gµ2 cos2?C
n ? p e ? p-? p0 e ? d ? u e- ?e' Gµ2 cos2?C
K-? p0 e ? ? ? p e ? s ? u e- ?e' Gµ2 sin2?C
µ ? e ? ? - Gµ2
12
b) Cabbibo angle ?C

• decay rates found such that sin2?C cos2?C 1,
  with
• sin2?C 0.05, cos2?C 0.95 (120),
• sin ?C 0.22, cos ?C 0.97,
• ?C 130 0.22,
• Cabbibo
• quark mixing is 'zero-sum game',
• is pure rotation in flavor space,
• quark mixing matrix is unitary

13
c) 70ies more flavors
1970, GIM "a 4th flavor charm c, would
naturally explain the observed absence of
neutral currents in ?S0" 1972, KM "a 3rd
generation bottom b, top t, would naturally
incorporate violation of T-invariance via a
complex phase f" with si sin?i, ci
cos?i, (i1,2,3, for 1?2, 1?3, 2?3 generation
mixing)
14
d) ever since filling of the CKM matrix