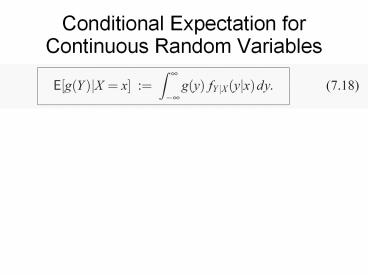
Conditional Expectation for Continuous Random Variables

1
Conditional Expectation for Continuous Random
Variables
2
(No Transcript)
3
(No Transcript)
4
(No Transcript)
5
(No Transcript)
6
(No Transcript)
7
This gives us the Law of Total Probability for
Expectation
8
(No Transcript)
9
(No Transcript)
10
(No Transcript)
11
(No Transcript)
12
(No Transcript)
13
Example 7.18
14
7.4 The Bivariate Normal
We begin be recalling the univariate normal. Let
? denote the standard N(0,1) density
Then the N(m,s2) density can be written as
15
For the bivariate normal, we start in a similar
way. For
If ? 0, then
is the product of two univariate standard normals.
16
(No Transcript)
17
(No Transcript)
18
We show that the standard bivariate density
integrates to one. First, for all ? lt 1,
19
We show that the standard bivariate density
integrates to one. First, for all ? lt 1,
20
We show that the standard bivariate density
integrates to one. First, for all ? lt 1,
21
(No Transcript)
22
(No Transcript)
23
(No Transcript)
24
(No Transcript)
25
From
we see that X and Y are independent if and only
if they are uncorrelated (? 0) since this is
the condition for the density to factor.
26
Reminder
(7.23)
27
(No Transcript)
28
(No Transcript)
29
(No Transcript)
30
(No Transcript)
31
(No Transcript)
32
(No Transcript)
33
(No Transcript)