13.1 Fourier transforms:

1
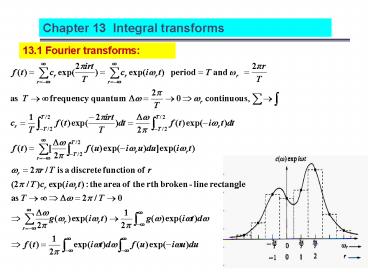
Chapter 13 Integral transforms
13.1 Fourier transforms
2
Chapter 13 Integral transforms
The Fourier transform of f(t)
Inverse Fourier transform of f(t)
Ex Find the Fourier transform of the exponential
decay function and
Sol
3
Chapter 13 Integral transforms
Properties of distribution
4
Chapter 13 Integral transforms

• The uncertainty principle

Gaussian distribution probability density
function
(1) is symmetric about the point
the standard deviation describes the width of a
curve (2) at falls to
of the peak value, these
points are points of inflection
5
Chapter 13 Integral transforms
Ex Find the Fourier transform of the normalized
Gaussian distribution.
Sol the Gaussian distribution is centered on
t0, and has a root mean square
deviation
1

• is a Gaussian distribution centered on
  zero and with a root
• mean square deviation
  is a constant.

6
Chapter 13 Integral transforms
Applications of Fourier transforms
(1) Fraunhofer diffractionWhen the cross-section
of the object is small compared with the distance
at which the light is observed the pattern is
known as a Fraunhofer diffraction pattern.
7
Chapter 13 Integral transforms
Ex Evaluate for an aperture consisting of
two long slits each of width 2b whose centers are
separated by a distance 2a, agtb the slits
illuminated by light of wavelength .
8
Chapter 13 Integral transforms

• The Diracd-function

9
Chapter 13 Integral transforms
Ex Prove that
10
Chapter 13 Integral transforms

• consider an integral
  to obtain

Proof

• Define the derivative of

11
Chapter 13 Integral transforms

• Physical examples for d-function

• an impulse of magnitude
  applied at time
• a point charge at a point
• (3) total charge in volume V
  

• unit step (Heviside) function H(t)

12
Chapter 13 Integral transforms

Proof

• Relation of the d-function to Fourier transforms

13
Chapter 13 Integral transforms

• for large becomes very
• large at t0 and also very narrow
• about t0
• as

14
Chapter 13 Integral transforms

• Properties of Fourier transforms
• denote the Fourier transform of by
  or

15
Chapter 13 Integral transforms
16
Chapter 13 Integral transforms
17
Chapter 13 Integral transforms
Consider an amplitude-modulated radio wave
initial, a message is represent by ,
then add a constant signal
18
Chapter 13 Integral transforms

• Convolution and deconvolution

Note x, y, z are the same physical variable
(length or angle), but each of them appears three
different roles in the analysis.
19
Chapter 13 Integral transforms
Ex Find the convolution of the function
with the
function in the above figure.
Sol
20
Chapter 13 Integral transforms
The Fourier transform of the convolution
21
Chapter 13 Integral transforms
The Fourier transform of the product
is given by
22
Chapter 13 Integral transforms
Ex Find the Fourier transform of the function
representing two wide slits by considering the
Fourier transforms of (i) two d-functions, at
, (ii) a rectangular function of height 1
and width 2b centered on x0
23
Chapter 13 Integral transforms
Deconvolution is the inverse of convolution,
allows us to find a true distribution f(x) given
an observed distribution h(z) and a resolution
unction g(y).
Ex An experimental quantity f(x) is measured
using apparatus with a known resolution function
g(y) to give an observed distribution h(z). How
may f(x) be extracted from the measured
distribution.
the Fourier transform of the measured distribution
extract the true distribution
24
Chapter 13 Integral transforms

• Correlation functions and energy spectra

The cross-correlation of two functions and
is defined by
It provides a quantitative measurement of the
similarity of two functions and as one is
displaced through a distances relative
to the other.
25
Chapter 13 Integral transforms
26
Chapter 13 Integral transforms
Parseval