PowerPoint-Pr - PowerPoint PPT Presentation
Title: PowerPoint-Pr
1
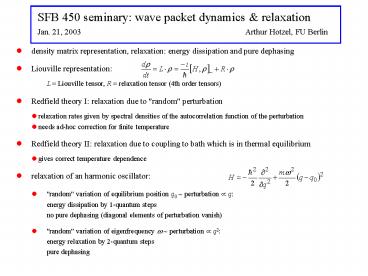
SFB 450 seminary wave packet dynamics
relaxation Jan. 21, 2003 Arthur
Hotzel, FU Berlin
? density matrix representation, relaxation
energy dissipation and pure dephasing
? Redfield theory I relaxation due to "random"
perturbation ? relaxation rates given by
spectral densities of the autocorrelation
function of the perturbation? needs ad-hoc
correction for finite temperature
? Redfield theory II relaxation due to coupling
to bath which is in thermal equilibrium ? gives
correct temperature dependence
? "random" variation of equilibrium position q0
perturbation ? q energy dissipation by
1-quantum steps no pure dephasing (diagonal
elements of perturbation vanish)
? "random" variation of eigenfrequency w
perturbation ? q2 energy relaxation by
2-quantum steps pure dephasing
2
Coupled harmonic oscillators Excited state
intramolecular proton transfer (ESIPT) SFB 450
seminary, Jan. 21, 2003
Arthur Hotzel, FU Berlin
2,5-bis(2-benzoxazolyl)-hydroquinone (BBXHQ)
? proton transfer in the first excited state
(singlet), enol (A) ? keto (B) ? high-frequency
proton oscillation around equilibrium positions
A, B (coordinate q) ? proton site-site distance
modulated by low-frequency scaffold mode
(coordinate Q)
3
Dynamics without dissipation
? consider only first electronically excited enol
and keto singlet states
4
Proton wave functions
5
Scaffold vibrational states
? Effective scaffold potentials are harmonic
potentials with vibrational energy hW 14.6 meV,
reduced mass M 47.8 amu (proton vibrational
energy hw 335 meV).
? Keto and enol scaffold equilibrium positions
are shifted by 0.077 Å with respect to each other.
6
Enol-keto coupling
7
Total Hamiltonian in the enol/keto basis
? Eigenstates of H (considering enol/keto states
a 0, ..., 9, a' 0, ..., 9)
? Initial state Excitation from molecular ground
state with delta pulse scaffold ground state
equilibrium position shifted by 0.077 Å with
respect to electronically excited enol state.
H eigenstates
pure enol states
initial state (enol basis)
pure keto states
initial state (energy basis)
energy amu Å2 ps-2
QÅ
8
Wavefunction dynamics without dissipation
? express initial state in terms of eigenstates
of H
? transfer back into enol/keto basis
9
blue projection onto enol basis red projection
onto keto basis
energy (reduced enol/keto Hamiltonian) amu Å2
ps-2
elapsed time oscillation periods 0.283 ps
QÅ
10
Dissipation
? We consider random perturbation of the form (in
enol/keto basis)
? Random perturbation proportional to scaffold
elongation from equilibrium (Q - Q0) in the enol
and keto states.
11
Relaxation tensor
? Make basis transformation to eigensystem of H
? We assume short correlation time tc of random
correlation
? We take f 200 ps
12
Wavepacket dynamics with dissipation
? diagonalize L eigenvalues Lk, eigenvectors sk
? express initial state r(t 0) in terms of
eigenstates of L
? transfer back into eigenvector basis of H and
then into enol/keto basis
13
blue projection onto enol basis red projection
onto keto basis
energy (reduced enol/keto Hamiltonian) amu Å2
ps-2
elapsed time oscillation periods 0.283 ps
QÅ