Principal axes

1
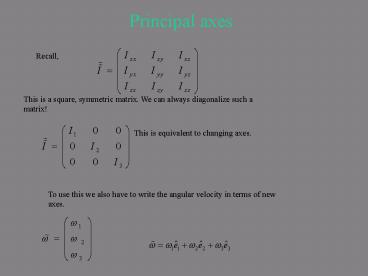
Principal axes
Recall,
This is a square, symmetric matrix. We can always
diagonalize such a matrix!
This is equivalent to changing axes.
To use this we also have to write the angular
velocity in terms of new axes.
2
Quantities expressed with principal axes
What are these angles measured relative to?
3
Rigid body rotational dynamics
Two constants of motions. What are they?
What about in the principal axis frame?
Think about these equations geometrically What
sort of geometric objects are they? That both
equations must be satisfied means what? What are
the axes for the geometric objects?
4
Rigid body rotational dynamics
Two constants of motions. What are they?
What about in the principal axis frame?
Think about these equations geometrically What
sort of geometric objects are they? That both
equations must be satisfied means what? What are
the axes for the geometric objects?
5
Rigid body rotational dynamics Geometric
Two constants of motions. What are they?
What about in the principal axis frame?
Think about these equations geometrically What
sort of geometric objects are they? That both
equations must be satisfied means what? What are
the axes for the geometric objects?
6
Rigid body rotational dynamics Non-geometric
Eulers equations
7
Problem
Imagine a lamina of arbitrary shape rotates
freely under zero torque
is constant., where the 1-2 plane is the plane of
the lamina
Show that the sum
What conditions are required for w3 to be
constant?