Prezentacja programu PowerPoint

1
4. Microsystems in measurements of mechanical
quantities- displacement, velocity and
acceleration

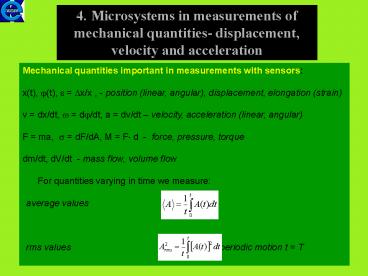
• Mechanical quantities important in measurements
  with sensors
• x(t), ?(t), ? ?x/x , - position (linear,
  angular), displacement, elongation (strain)
• v dx/dt, ? d?/dt, a dv/dt velocity,
  acceleration (linear, angular)
• F ma, ? dF/dA, M F? d - force, pressure,
  torque
• dm/dt, dV/dt - mass flow, volume flow
• For quantities varying in time we measure
• average values
• rms values for periodic motion t T

2

• The unknown parameters can be determined from the
  basic relationships between quantities, e.g. from
  the knowledge of acceleration one obtains
  succesively
• Bearing in mind determination of integration
  constants, it is necessary to do additional
  measurements.
• Recent MST technologies allow to fabricate low
  cost but precise acceleration sensors.
• Accelerometers, regardless of the conversion
  technique, require the existence of a seismic
  mass, which displacement with respect to the
  housing is rgistered. Taking into account the
  conversion technique of a displacement, one deals
  with different kinds of accelerometers
  piezoelectric, piezoresistive, capacitive,
  thermal.

3
Mechanical model of vibration sensor
An equation of motion of mass m with respect to
the reference frame with coordinate y, under the
influence of spring force ky, damping force b
dy/dt and inertial force md2x/dt2 can be
written as
Adjusting the oscillator constants one can
neglect selected terms in the equation, thus
obtaining different sensors

• m large
• b small position sensor
• k small
• 2. m small
• b large velocity sensor
• k small
• m small
• b small accelerometer
• k large

Sensor case moves relative to the Earth along a
coordinate x
In reality the sensor mass should be small enough
to avoid influence on the investigated object. In
this case one can build a sensitive accelerometer
and other vibration parameters can be obtained by
integration of acceleration.
4
Piezoelectric accelerometer
Piezoelectric plates are sandwiched between the
casing and the seismic mass, which exerts on them
a force proportional to acceleration.
1. seismic mass 2. piezoelectric plates (with
magnification) 3. tension control 4. FET
preamplifier 5. cable attachment
In MEMS technology a silicon cantilever with
deposited piezoelectric film, e.g. BaTiO3 is used.
Experimental setup with piezoelectric
accelerometer used for investigation of vibration
parameters.
5
Piezoelectric effect
Section of the quartz crystal in a plane
perpendicular to c axis (z-axis). There exist 3
mechanical axes (perpendicular to crystal
planes) and 3 electrical axes (drawn through
edges). A plate cut from the crystal is also
shown.
Applying a force to the plate along the
electrical axis one generates the charge on the
surfaces, to which a stress is applied
(longitudinal piezoelectric effect). Acting with
a force along mechanical axis y we induce a
charge on the surfaces as before (transversal
effect).
6
Piezoelectric effect, cont.
Quartz crystal structure (the first atomic layer
is shown, in the second layer there are 3 O2-
atoms, the third layer is identical as the first
one, aso.)
Transversal effect
Longitudinal effect
7
Piezoelectric effect, cont.
Application of stress s generates a charge with
density q
kp piezoelectric module (for quartz 2.2 10-12
C/N, for ferroelectrics ca.100 times higher)
For the longitudinal effect (force Fx) one
obtains for surface Ax a charge Q Axq
Axkps kpFx - independent of Ax For
transversal effect (force Fy) one gets Q
-kpFy b/a
8
Piezoelectric effect, cont.
Generated charge on capacitance C gives the
voltage U Q/C Q/(Ck Cm) kpFx/C, Ck,
Cm cap. of crystal and cable, resp. For n
parallel connected plates one gets U nQ/(nCk
Cm) This gives piezoelectric sensitivity Sp
dU/dFx nkp/(nCk Cm) Sensor discharge time
constant is then equal t (Ck Cm)/(Gk Gm)
G conductance This time constant limits
fmin.
9
Capacitive sensor
Capacitance of a flat-plate capacitor C
e0er A/ l A - plate area, l distance
between plates
Sensitivity dC/dl - e0erA/ l2 , changes with l
dC/C - dl/l , high relative sensitivity for
small l (nonlinearity)
10
Differential capacitor
?C C2 C1 e0er A2?l/(l2 ?l2) for ?l ltlt
l ?C e0erA2?l/l2, hence ?C/C 2?l/l
Therefore one obtains increased sensitivity and
linearity. Differential technique decreases the
temperature error and the influence of e drift.
11
Capacitive displacement sensor
Cylindrical capacitive sensor with movable
dielectric shaft in ratiometric configuration W
common electrode S fixed electrode R
variable electrode
The displacement causes moving of the shaft and
is calculated from the ratio of capacitances
CWR/CWS In practice the S electrode needed
carefull screening to avoid the inflence of air
humidity variations on CWS capacitance (change in
configuration of the electric field).
12
Angular position sensor
In the simplest case a rotating capacitor can be
used
12
13
Angular position sensor, cont.
Practical realisation of a differential rotating
capacitor (Zi-Tech Instruments Corp.)
Stators 1 and 2 form with a rotor separate
capacitances. The difference of those
capacitances varies linearly with movement of the
rotor.
14
Capacitive accelerometer
From discussion of the mechanical model of
vibration sensor it follows that for the system
with high spring konstant k we can cosider the
deflection, i.e. also the change in capacitance,
as proportional to the acceleration. In this
case one obtains a capacitive accelerometer.
A capacitive accelerometer with a differential
capacitor fabricated in silicon bulk
micromachining technology.
The movable mass is sandwiched between upper and
base fixed electrodes forming two variable
capacitances.
15
Examples of MEMS accelerometers Analog Devices
ADXL250 (on the left) and Motorola dual-structure
microsystem before encapsulation (on the right)
16
Tilt (inclination) sensor based on capacitive
accelerometer
Construction of integrated Analog Devices
accelerometer (a) scheme of interdigitated
differential capacitor, (b) upper view of the
sensing structure.
The central moving belt forms with static belts
the interdigitated structure (46 capacitors) and
deflects from the central position by inertial
forces.
17
Inclination sensor based on capacitive
accelerometer, cont.
Determination of the tilt angle ? from
measurements of the gravitational acceleration
Tilt angle determination for single axis and
dual axis sensors (g 1)
single axis sensor
dual axis sensor
For single axis sensor the sensitivity decreases
with ?