What Is a Numerical Weather Prediction Model?

1
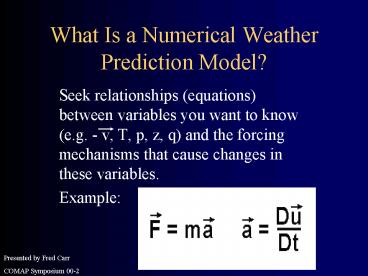
What Is a Numerical Weather Prediction Model?

• Seek relationships (equations) between variables
  you want to know (e.g. - v, T, p, z, q) and the
  forcing mechanisms that cause changes in these
  variables.
• Example

Presented by Fred Carr COMAP Symposium 00-2
2

• In meteorology, we solve for Du/Dt
• (1)

3

• Or
• or
• Example of a prognostic equation

4

• Example of a diagnostic equation
• Just consider vertical component of (1)
• rhs balances perfectly for large-scale flow
• Hydrostatic eq. - used to deduce Z from T

5

• Thus, the essential components of an NWP model
  are
• 1. Physical Processes - RHS of equations (e.g.,
  PGF, friction, adiabatic diabatic heating
  (advection terms are also on rhs unless have
  Lagrangian model)

6

• 2. Numerical Procedures
• approximations used to estimate each RHS term
  (especially imp. for advection terms)
• approximations used to integrate model forward
  in time
• grid used over model domain (resolution)
• boundary conditions
• Note Quality and quantity of observations
  (initial conditions) equally vital to NWP system
• Need to observe prognostic variables

7
Primitive Equation Models

• A. Primitive Equations
• It was recognized early in the history of NWP
  (Charney, 1955) that the primitive equations of
  motion would be best suited for development of
  comprehensive dynamical-physical models of the
  atmosphere. Although the problems of
  initialization and numerical integration of the
  primitive equations are still areas of research,
  stable forecasts from these equations have been
  produced for over 35 years.

8

• We will present the primitive equations in x-y-p
  coordinates and discuss some general properties
  of this system. These equations are equally
  appropriate for global as well as limited-area
  models.
• A set of governing equations that describe
  large-scale atmospheric motions can be derived
  from conservation laws governing momentum, mass,
  energy, and moisture (see Holton, 1979 - Chap.
  2). These are called the primitive

9

• equations, not because they are crude or
  simplistic but because they are fundamental or
  basic. Using the Eulerian framework in x-y-p
  coordinates, they can be written as follows
• (1)
• (2)
• (3)
• (4)
• (5)
• (6)

10

• Eqs. (1) and (2) are the horizontal momentum
  equations for the u- and v- components of the
  wind, respectively. Note that (via scale
  analysis), the curvature terms and the 2??cos?
  Coriolis term have been neglected. Eq. (3) is
  the vertical momentum equation under the
  assumption of hydrostatic balance (diagnoses z).
  The continuity equation (4) expresses the
  conservation of mass (diagnoses w). The First
  Law of Thermodynamics yields an energy equation
  for temperature (5). Eq. (6) is the conservation
  of moisture equation where q is specific
  humidity.

11

• The dependent variables in this set of equations
  are u, v, ?, ?, T, and q which are assumed to be
  continuous functions of the independent variables
  x, y, p, and t. Eqs. (1), (2), (5), and (6) are
  prognostic equations (involve a time derivative)
  and thus require initial conditions. Initial
  conditions are derived from observations or the
  use of some balance relationship (e.g. -
  obtaining u and v from ? by assuming geostrophic
  balance). Eqs. (3) and (4) are diagnostic
  equations and can be computed once the initial
  conditions are provided. Thus, (1) to (6)
  constitute a set of 6 equations in 6 unknowns

12

• and we can say we have a closed system if
• i) we can find expressions for Fx, Fy, H, E,
  and P in terms of the known dependent
  variables
• ii) we have suitable initial conditions over
  the domain
• iii) suitable lateral boundary conditions for
  the dependent variables are formulated (for
  regional models) all models need boundary
  conditions at the top and bottom levels

13

• The first category above encompasses the whole
  subject of adding physics to the primitive
  equations. Fx and Fy are friction terms which
  modify the momentum equations via surface drag
  (skin friction) and horizontal and vertical
  transport of momentum by turbulent eddies of
  various sizes (generally called diffusion in
  large-scale models). The diabatic heating term H
  also consists of several effects which can be
  written
• H HL HC HR HS (7)

14

• where HL is due to latent heat of condensation
  caused by the large-scale dynamic ascent of
  stably-stratified, saturated air (grid-scale
  precipitation), HC is the latent heat rate due to
  convection (cumulus parameterization), HR is the
  radiative heating rate and HS represents sensible
  heat flux from the surface of the earth. One
  of the most difficult problems in NWP is how to
  formulate proper expressions for the net effects
  of HC, HR, and HS (which are generally
  subgrid-scale processes) in terms of the
  large-scale dependent variables (the
  parameterization problem). The precipitation
  rate PPLPC is known once HL and HC are known.

15

• Evaporation E can be due to moisture flux from
  the surface and evaporation of precipitation.
  The effect of mountains also has to be included
  in the model via the lower boundary condition and
  choice of vertical coordinate .
• Once conditions (i) to (iii) are suitably met
  (the fact that they are never perfectly met
  accounts for a large part of the total forecast
  error), the equations (1) to (6) can, in
  principle, be solved in the following
  straightforward manner

16

• 1. Obtain observations of the prognostic
  variables u, v, T, and q over the domain
• 2. Compute ? from (3) and ? from (4)
• 3. Compute Fx, Fy, H, E, P and the other
  terms on the right-hand sides of (1), (2), (5),
  and (6)
• 4. Integrate the four prognostic equations
  forward in time to obtain new values of u, v,
  T, and q
• 5. Repeat steps 2 to 4 until complete the
  forecast

17

• Since there are nearly an infinite number of ways
  to formulate the physics and many numerical
  procedures are available for the solution of the
  equations, no two numerical models are alike.
  Thus each model may have systematic errors or
  biases peculiar to itself. However, some errors,
  such as those arising from insufficient
  resolution, are common to all models. It is
  important for forecasters to become aware of
  these limitations in order to make Intelligent
  Use of Model Guidance.