Sec 1'5 Describe Angle Pair Relationships

1
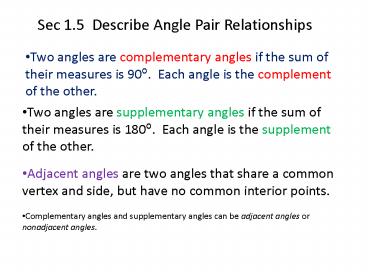
Sec 1.5 Describe Angle Pair Relationships

• Two angles are complementary angles if the sum of
  their measures is 90. Each angle is the
  complement of the other.

• Two angles are supplementary angles if the sum of
  their measures is 180. Each angle is the
  supplement of the other.

• Adjacent angles are two angles that share a
  common vertex and side, but have no common
  interior points.

• Complementary angles and supplementary angles can
  be adjacent angles or nonadjacent angles.

2
Complementary angles
Supplementary angles
3
EXAMPLE 1
Identify complements and supplements
SOLUTION
4
EXAMPLE 2
Find measures of a complement and a supplement

• Given that 1 is a complement of 2 and
  m 1 68,
• find m 2.

SOLUTION
5
for Examples 2 and 3
GUIDED PRACTICE
5. LMN and PQR are complementary angles.
Find the measures of the angles if m LMN
(4x 2) and m PQR (9x 1).
SOLUTION
Complementary angle
(4x 2 ) ( 9x 1 ) 90
Substitute value
13x 1 90
Combine like terms
13x 91
Add 1 to each side
x 7
Divide 13 from each side
6
for Examples 2 and 3
GUIDED PRACTICE
Evaluate the original expression when x 7
7

• Two adjacent angles are a linear pair if their
  noncommon sides are opposite rays.

• The angles in a linear pair are always
  supplementary angles.

• The only difference between a linear pair and
  supplementary angles is that a linear pair are
  always adjacent, or connected.

• Two angles are vertical angles if their sides
  form two pairs of opposite rays.
• These angles have the same vertex and open in
  opposite directions.

8
EXAMPLE 5
Find angle measures in a linear pair
SOLUTION
9
EXAMPLE 5
Find angle measures in a linear pair
Write an equation.
x 5x 180
Combine like terms.
6x 180
Divide each side by 6.
x 30
10
Interpreting a Diagram

• There are some things you can conclude from a
  diagram, and some you cannot.

11
(No Transcript)
12

• Homework
• Advanced Geometry
• Pg. 38-40
• 4-16 even, 17-29 all, 32, 3438 all, 40, 42
• 28 problems