Warm Up Equation for a circle

1
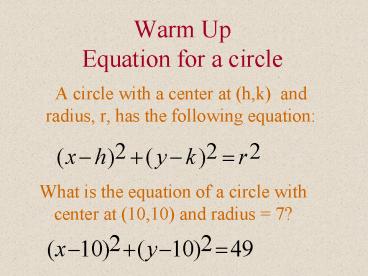
Warm UpEquation for a circle

• A circle with a center at (h,k) and radius, r,
  has the following equation

What is the equation of a circle with center at
(10,10) and radius 7?
2
Inscribed Polygons

• Section 3-5

• Today we will
• Learn what an inscribe polygon is
• Learn a procedure for finding the equation of a
  circle with an
• Inscribed triangle inside

3
Definition

• Chord A segment joining two points on a circle

4
Inscribed Polygons

• Polygons inside a circle that circumscribes it

C

• Sides of triangle that join 2 points on circle
  are chords
• All regular polygons can be inscribed in a circle

A
B

Theorem The perpendicular bisector of a chord of
a circle passes through the center of the
circle. We will use this theorem to find the
center and equation for circles.
5
Procedure for finding the equation of a circle
with a triangle inscribed
Consists of 2 major steps 1) Find the equations
for the perpendicular bisectors of 2 sides of the
triangle (chords of circle). 2) Find the center
of the circle by finding the intersection of
these two perpendicular bisectors.
6
A (10,10)
Finding equations perpendicular bisector (chord
AC first) 1) Find slope of chord
B (8,-7)
C (-6,1)
2) Find slope of perpendicular bisector to
chord Slope negative reciprocal mAC
7
A (10,10)
3) Find midpoint of chord
B (8,-7)
C (-6,1)
4) Use slope and midpoint to find the equation
for the bisector
Equation bisector AC y -1.78x 9.06
8
Repeat process for chord BC (could also choose
AB) 1) Slope BC 2) Slope
Perpendicular Bisector
A (10,10)
B (8,-7)
C (-6,1)
9
3) Midpoint BC 4) Equation Perpendicular Bise
ctor
A (10,10)
B (8,-7)
C (-6,1)
10

• Find the Equation for the Perpendicular Bisector
  for a line with end points
• (2,4) and (8,10)
• Slope -1
• Midpoint (5,7)
• Equation y -x 12

11
To find center of circle 1) Find intersection
of two perpendicular bisectors by setting one
equation equal to the other and solving for x and
y.
x 3.91
Center of circle is (3.91, 2.09)
12
Find the center of the circle for the following
two perpendicular bisectorsy -3x 7y 2x
-3
(2,1)
13
A (10,10)
To find equation of circle use formula
B (8,-7)
C (-6,1)
Where (h, k) is the center of the circle. Center
at (3.91, 2.09), point (x 3.91)2 (y 2.09)2
r2
14
A (10,10)
To find equation of circle use formula
B (8,-7)
C (-6,1)
To find the radius On circle choose any
vertex of triangle. Ex (10,10) and substitute in
for (x, y)
15
A (10,10)
To find equation of circle use formula
B (8,-7)
C (-6,1)
Check by showing that point (-6,1) is on the
circle
(-6 3.91)2 (1 2.09)2 99.6 98.1 1.19
99.6 99.4 99.6 (Close enough)
16
Write the equation for a circle with a center at
(2,4) going through the point (6,8)
(x 2)2 (y 4)2 32
17
Homework
P. 168-169 2-5 http//www.pbs.org/wgbh/nova/arch
imedes/pi.html Read through the description of
how Archimedes approximated pi. Write a paragraph
summary. Launch the interactive tool. Change the
number of sides for the inscribed polygon. Write
value of pi for this. Change the number of sides
for the circumscribed polygon. Write the value of
p for this. Do this 6 times. (3 points)