Geometric Configurations

1
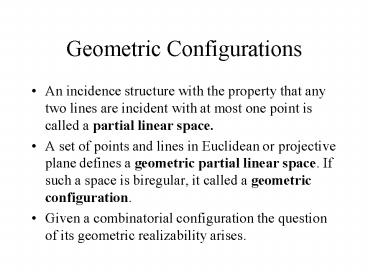
Geometric Configurations

• An incidence structure with the property that any
  two lines are incident with at most one point is
  called a partial linear space.
• A set of points and lines in Euclidean or
  projective plane defines a geometric partial
  linear space. If such a space is biregular, it
  called a geometric configuration.
• Given a combinatorial configuration the question
  of its geometric realizability arises.

2
Astral and Stellar Geometric Configurations

• Combinatorial cyclic configuration contains all
  points (and all lines) in a single orbit. For k gt
  2 we cannot draw such a geometric configuration
  in the Euclidean plane in such a way as to
  display the same (Euclidean) symmetry, i.e.
  isometry. Clearly, each (v2) confiugration can
  be drawn with a single orbit. .
• If there exists a geometric realization with s
  orbits (of equal size), a configuration is called
  s-stellar.
• For a stellar (vk) configuration we have k 2s.
• When s is minimal, s d k/2 e the configuration
  is called astral. For the most interesting cases
  k 3 and k 4 this means two orbits s 2.

3
The Smallest Astral (v3) Configuration

• The smallest astral (103) configuration.

4
The Smallest Astral (v3) Configuration with
Dihedral Symmetry

• The smallest astral (v3) configuration with
  dihedral symmetry has v 12.

5
The Smallest Astral Triangle-Free (v3)
Configuration

• The smallest astral triangle-free (v3)
  configuration has v 18.

6
A 4-stellar configuration

• A stellar (603) configuration that is
  combinatorially equivalent to an astral
  configuration.

7
A 4-stellar configuration
8
15. Existence and Enumeration of Combinatorial
Configurations
9
Lineal Configurations

• In order to emphasise configurations as parital
  linear spaces we call them lineal configurations
  ( digon free configurations).

10
Existence of Lineal Configurations

• Proposition For each lineal (vr,bk)
  configuration (r k) the following is true
• v.r b.k
• b v 1 r(k 1)
• Corollary For symmetric (vk) configurations the
  following lower bound is obtained
• v 1 k(k-1) 1 k k2
• In particular
• For k 3 we have v 7,
• For k 4 we have v 13,
• For k 5 we have v 21.

11
Lower Bounds (Adapted from Grünbaum)
r\k 3 4 5 6 7
3 (73) (123,94) (203,125) (263,136) (353,157)
4 (94,123) (134) (204,165) ?(304,206)? ?(494,,287)?
5 (125,203) (165,204) (215) (305,256) ?(425,307)?
6 (136,263) ?(206,304)? (256,305) (316) X(496,427)X
7 (157,353) ?(287,494)? ?(307,425)? X(427,496)X X(437)X
12
Duality

• Each incidence structure C (P,L,I) gives rise
  to a dual structure Cd (L,P,Id) with the role
  of points and lines reversed and keeping the
  incidence.
• The structures C and Cd share the same Levi graph
  with the roles of black and white vertices
  reversed.

13
Self-Duality and Automorphisms

• If C is isomorphic to its dual Cd , it is said
  that C is selfdual, the corresponding
  isomorphism is called a (combinatorial) duality.
• Duality of order 2 is called (combinatorial)
  polarity.
• An isomorphism mapping C to itself is called an
  automorphism or (combinatorial) collinearity.

14
Automorphisms and Antiautomorphisms

• Automorphisms of the incidence structure C form a
  grup that is called the group of automorphisms
  and is denoted by Aut0C.
• If automorphisms and dualities (antiautomorphisms)
  are considered together as permutations, acting
  on disjoint union P ? L, the extended group of
  automorphism Aut C is obtained.
• Warning If C is disconnected there may be
  mixed automorphisms.

15
Graphs and Configurations

• The Levi graph of a configuration is bipartite
  and carries complete information about
  configuration.
• Assume that C is connected. The extended group of
  automorphisms AutC coincides with the group of
  automorphisms of Levi graph L, while Aut0C
  stabilises both partite sets.

16
Blocking Set

• A set of points B of an incidence structure is
  called a blocking set, if each line L contains
  two points x and y, such that
• x 2 B and (x,L) 2 I,
• y Ï B and (y,L) 2 I.

17
Notation
18
Counting (v3) Configurations
19
Counting Triangle-Free (v3) Configurations