Circuit Parameters

1
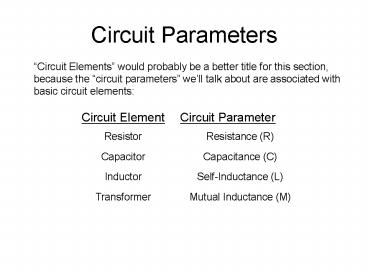
Circuit Parameters
Circuit Elements would probably be a better
title for this section, because the circuit
parameters well talk about are associated with
basic circuit elements
2
Resistive Circuits
The relationship between the Voltage across a
resistor and the current flowing through it is
very simple V IR. If the current is a
function of time i(t) as shown below, the Voltage
is also a function of time
i(t) and v(t) are related by a constant
multiplier, so the current waveform must have the
same shape as the Voltage waveform.
i(t)
This is true for a single resistor, or for a
network of resistors (and no other types of
elements. In a resistive network, all node
Voltage and branch current waveforms have the
same shape as the stimulus waveform.

R
v(t)
-
3
Resistive Circuits
If a resistive networks stimuli are provided by
more than one current or Voltage source, then the
superposition theorem tells us the networks
response will be the sum of the responses to each
source indiviually. Unless all stimulus
waveforms have the same shape, the shape of the
response waveform is not the same as any of the
stimulus waveforms. Heres a simple example.
Well find the response v3(t).
R
R
-
-
v3(t)
e1(t)
-
R
e2(t)
4
Resistive Circuits
To find the response to e1(t), replace e2(t) with
a short circuit
This response was found using mesh analysis, but
any other valid circuit analysis technique would
give the same result. Similarly, replacing e1(t)
with a short circuit results in the following
response
R
R
-
-
v3(t)
e1(t)
-
R
e2(t)
5
Resistive Circuits
The response to both stimuli is, of course, the
superposition of the response to e1(t), and the
response to e2(t).
Suppose e1(t) is a trapezoid waveform, and e2(t)
is a sinusoid. The response v3(t) is clearly
neither a trapezoid nor a sinusoid, it is the
sum of a trapezoid and a sinusoid.
R
R
-
-
v3(t)
e1(t)
-
R
e2(t)
6
Resistive Circuits
The instantaneous power dissipated by a resistor
may be found from the current flowing through it,
or the Voltage across it
If the Voltage or current waveform is periodic,
the average power dissipated by the resistor may
be found using the rms value
i(t)

R
v(t)
-
7
Capacitance
Capacitance is a characteristic of a capacitor,
which is one of the basic circuit elements. You
should recall that a capacitor is two flat,
parallel conductive plates separated by an
insulating dielectric. This means no current can
actually flow through the capacitor because no
electrons can cross the dielectric. However, the
capacitor does store charge in its plates. The
amount of charge stored is proportional to the
Voltage across the capacitor (v(t) for the
capacitor shown here), so if v(t) is a
time-varying
Voltage, the stored charge is also time-varying.
As the stored charge changes, electrons must move
in or out of the capacitor, so it appears that
current is flowing through it. For all practical
purposes, if v(t) is not constant, a current i(t)
actually does flow through the capacitor (even
though no electrons cross the dielectric).
i(t)

C
v(t)
-
8
Capacitance
Current flows through the capacitor only when the
Voltage across the capacitor is changing. The
rate of change of the Voltage v(t) is its
derivative dv/dt, which is zero whenever v(t)
remains constant for a time interval Dt gt 0.
Whenever dv/dt 0, i(t) 0. In fact, the
current i(t) is proportional to the rate of
change of v(t)
In which the constant of proportionality C is
capacitance, the circuit parameter associated
with a capacitor. This is the current-Voltage
relationship for a capacitor. If v(t) is a DC
Voltage, i(t) 0. This means that a capacitor
behaves like an open circuit to a DC Voltage.
i(t)

C
v(t)
-
9
Capacitance
Heres an example The capacitor Voltage
waveform shown below is trapezoidal in shape.
Its slope is constant over each of the four
segments, but each segment has a different slope
10
Capacitance
Heres the trapezoidal waveform, along with its
slope. The shape of the current waveform is the
same as the shape of the slope plot.
11
Capacitance
Weve just considered the case in which v(t) is
the stimulus and i(t) is the response. Its also
possible for the stimulus to be the capacitor
current i(t) and the response v(t). The
capacitors current-Voltage relationship,
Is a very simple first-order differential
equation. By rearranging terms, its possible to
put it in this form
i(t)

Which can be integrated
C
v(t)
-
12
Capacitance
Were often interested only in what happens for t
gt 0, so lets define V0 as the capacitor Voltage
at t 0
V0 accounts for all the charge stored in the
capacitor before t 0. Its the initial
condition of the differential equation. Now, for
t gt 0,
i(t)

C
v(t)
-
13
Capacitance
Unlike a resistor, a capacitor doesnt dissipate
power. It stores energy in the electric field
between the plates, and this energy is available
to be returned to the system at any later time.
Because it doesnt convert electrical energy to
heat the way a resistor does, an ideal capacitor
doesnt get hot. The amount of energy stored in a
capacitor is proportional to the Voltage to which
it is charged
i(t)
In which W represents the stored energy in
Joules, and V represents the Voltage across the
charged capacitor. The charge Q stored on the
capacitor is given by Q CV.

C
v(t)
-
14
Initially Charged Capacitor
We just found that, if a capacitor is charged to
an initial Voltage V0 prior to t 0, its Voltage
for t gt 0 is given by
In this equation, v(t) is the sum of two
Voltages the initial Voltage V0 which accounts
for all charge stored on the capactor up to t
0, and the integral term which accounts for the
charge stored after t 0. The sum of two
Voltages may be modeled as two Voltages in series.
i(t)
C
-
v(t)
V0
-
15
Initially Charged Capacitor
The sum of two Voltages may be modeled as two
Voltages in series, as shown below. The DC
Voltage source represents the initial Voltage,
V0, and the capacitor represents the integral
term in the equation
Modeling an initially charged capacitor this way
allows us to any network which includes
capacitors as if the capacitors are initially
discharged. It does increase the number of
Voltage sources in such a network, but that is
easily dealt with.
i(t)

i(t)
C
v(t)

-
-
v(t)
C
V0
V0
-
-
16
Initially Charged Capacitor
The charge stored on the capacitor is given by Q
CV, so the initial Voltage V0 represents an
initial charge Q0 CV0. The charged capacitor
can also be modeled by a discharged capacitor in
parallel with a current source which instantly
charges it at t 0. The current source has to
transfer charge Q0 CV0 to the capacitor to
charge it to its initial Voltage, and it has to
do it instantaneously.
i(t)
i(t)


C
CV0d(t)
v(t)
v(t)
C
-
V0
-
-
17
Initially Charged Capacitor
If the current sources waveform is an impulse
whose integral equal to the initial charge Q0,
the requirement is met. Thus, the circuit model
on the right is the Norton equivalent of the
model on the left.
i(t)
i(t)


C
CV0d(t)
v(t)
v(t)
C
-
V0
-
-
18
Self Inductance
Inductance is a characteristic of the basic
circuit element called an inductor. There are
two types of inductance mutual inductance
(which well study later) and self inductance.
An inductor is physically a coil of wire,
sometimes wound on a core of magnetic material
such as iron. In the old days, inductors were
called coils (and capacitors were called
condensers) or chokes. The inductors
current-Voltage relationship,
i(t)
Is very similar to that of the capacitor, with
current and Voltage reversed. The constant of
proportionality, L (the inductance in Henrys)
replaces C. Because the roles of current and
Voltage are reversed, the inductor is said to be
the dual of the capacitor.

L
v(t)
-
19
Self Inductance
Notice that the inductors current-Voltage
relationship,
Says that the Voltage across an inductor is zero
if the current flowing through it is constant
(much as the current flowing through a capacitor
is zero if the Voltage across it is constant).
Said another way, the inductor behaves like a
short circuit when a DC current is applied.
i(t)
The waveform of the Voltage across the inductor
has the shape of the slope of the current
waveform.

L
v(t)
-
20
Self Inductance
If we integrate the inductors current Voltage
relationship,
we get
The current waveform has the shape of the
integral of the Voltage waveform.
i(t)

L
v(t)
-
21
Self Inductance
If we integrate the inductors current Voltage
relationship,
we get
This can also be written in two parts
i(t)

The second term represents the initial current,
i(t 0), which well call I0
L
v(t)
-
22
Self Inductance
So the inductor current i(t) is given by
Like the capacitor, an inductor stores energy and
does not dissipate power. Energy is stored in
the inductors magnetic field, and later returned
to the system. The energy stored in the inductor
is given by
i(t)

L
Stanley calls an inductor which has energy stored
in its magnetic field fluxed. Stanley may be
the only person who uses that term. The word
charged is usually used instead.
v(t)
-
23
Initially Fluxed Inductor
The inductor current i(t) is given by
This is the sum of a DC current I0, and a
time-varying current. The time-varying term is
identical to the current flowing in a discharged
(disfluxed?) inductor. The charged inductor can
therefore be modeled using a discharged inductor
and a DC current source
i(t)
i(t)
i(t)


I0
v(t)
L
L
v(t)
-
-
24
Initially Fluxed Inductor
The Thevenin equivalent of that model may also be
used, as shown below. The development of this
model is similar to the development of the
capacitors Norton model.
i(t)
i(t)


L
I0
v(t)
L
v(t)
-
LI0d(t)
-
-
25
Mutual Inductance
Consider two coils, wound on a common iron core.
When a current i1 flows in the first coil (L1),
it establishes a flux. Nearly all of the flux
stays within the core, because its magnetic
reluctance is less than the surrounding air, so
the flux passes through (or links) both L1 and
L2. If the current i1 is time-varying, then so
is the flux, so a Voltage
v1 is induced across the terminals of L1 (self
inductance) and a Voltage v2 is induced across L2
(mutual inductance). If the terminals of L2 are
open circuited, v2 is given by
i2
i1


L2
L1
v2
v1
-
-
M is the mutual inductance of the two coils.
26
Mutual Inductance
The unit of mutual inductance is the Henry, which
is also the unit of self-inductance. If L1 is
open-circuited and a time-varying current i2
flows in L2, the mutual inductance causes a
Voltage v1 to be induced across L2
Notice that the mutual inductance M is the same
in both equations.
i2
i1


L2
L1
v2
v1
-
-
27
Mutual Inductance
Coils may be fluxed-linked like this without
being wound on a common core, just because they
are close enough for a portion of the flux from
one coil to link the other. This can happen by
accident. A pair of coils which are linked by
design form a transformer. In most cases, the
two coils of a transformer are wound on a common
iron core so nearly all of the flux links both
coils. If L2 is open circuited, so a Voltage is
induced across it, the polarity of the induced
Voltage may be reversed by reversing the
direction in
which either L1 or L2 is wound (clockwise or
counterclockwise). If the direction of both
coils is reversed, the polarity is reversed
twice, which is the same as not changing. The
relative direction of the windings in the two
coils may be indicated using the dot convention.
Notice the two dots next to the coils shown here.

L1
L2
28
Mutual Inductance
If the positive reference direction of the
current (that is, the direction of the current
reference arrow) is flowing into the dotted
terminal of either coil, then the induced Voltage
at the dotted terminal of the other coil is
positive with respect to the undotted
terminal. Two magnetically coupled coils with
self-inductances L1 and L2 and mutual inductance
M are shown here. Lets assume that
i1
i2
Then the self-induced Voltage v1 across L1 is
positive, as shown. Also, the dot convention
indicates that v2 is positive, as shown. If a
load is connected across L2 so a current may flow
in L2, the positive reference direction of that
current is as shown.


L1
L2
v1
v2
-
-
M
29
Mutual Inductance
If the positive reference direction of the
current (that is, the direction of the current
reference arrow) is flowing into the dotted
terminal of either coil, then the induced Voltage
at the dotted terminal of the other coil is
positive with respect to the undotted
terminal. Two magnetically coupled coils with
self-inductances L1 and L2 and mutual inductance
M are shown here. Lets assume that
i1
i2
Then the self-induced Voltage v1 across L1 is
positive, as shown, and is given by Also, the
dot convention indicates that v2 is positive, as
shown. If a load is connected across L2 so a
current may flow in L2, the positive reference
direction of that current is as shown.


L1
L2
v1
v2
-
-
M
30
Mutual Inductance
This figure illustrates the effects of the
self-inductance of both windings, as well as the
mutual inductances. Lets assume that an
external circuit is connected to both pairs of
terminals, so current can flow in both windings.
We can treat the circuit containing L1 as one
mesh, and the circuit containing L2 as another.
The Voltage source on the left represents the
Voltage induced across the L1
by the current i2. The reference direction of i2
is out of the dotted terminal, so the polarity of
the mutually-induced Voltage the L2 side is such
that the dotted terminal is negative with respect
to the undotted terminal.
i1
i2


-

-

v1
v2
-
-
-
-
31
Mutual Inductance
The Voltage source on the L2 side represents the
Voltage induced across the L2 winding by the
current i1. The positive reference direction of
i1 is into the dotted terminal, so the polarity
of the mutually-induced Voltage the L2 side is
such that the dotted terminal is positive with
respect to the undotted terminal.
The two mesh equations are
i1
i2


-

-

v1
v2
-
-
-
-
32
Ideal Transformers
Consider two magnetically-coupled coils which are
so tightly coupled that all the flux linking (or
passing through) one coil links both. If the
self-inductances of the two coils are very, very
large (approaching infinity), then the two coils
form an ideal transformer. Of course, ideal
transformers dont exist, but practical
transformers may approach the characteristics of
an ideal transformer. The ideal transformer is a
useful approximation.
It may be stated that
If the two coils are linked so tightly that all
the flux which links either coil links both,
L1
L2
33
Ideal Transformers
It may be stated that
If the two coils are linked so tightly that all
the flux which links either coil links both,
If we define the coefficient of coupling K as
Then, for an ideal transformer the coefficient of
coupling is 1. If K 0, then none of the flux
linking one coil links the other, so the two
coils do not form a transfomer. Two coils which
are designed to act as a transformer should have
a value of K near unity.
L1
L2
34
Ideal Transformers
Because a transformer operates by electromagnetic
induction, it only works if the Voltages and
currents applied to it are time-varying. A
transformer is usually designed and specified for
a particular operating frequency or frequency
range, and operating it outside that range will
not give good results.
A transformer is not usually specified in terms
of its self or mutual inductances. Its usually
specified in terms of its turns ratio. This is
the ratio of the number of turns on one winding
to the number of
turns on the other winding. The transformer
shown here has n times as many turns on the
right-hand winding as on the left-hand winding.
1n


L1
L2
v2
v1
-
-
35
Ideal Transformers
If excitation is applied to one winding and not
the other, the winding to which excitation is
applied is usually called the primary winding,
and the other winding is called the secondary.
If the transformer shown here has a Voltage or
current source connected to the terminals on the
left, then the left-hand winding is the primary.
For a nearly ideal transformer (most practical
transformers are nearly ideal), such as the one
shown here, the relationships between v1, v2, i1
and i2 are as follows
i1
i2
1n


L1
L2
v2
v1
-
-
36
Ideal Transformers
Suppose the left-hand winding is the primary, and
excitation is applied to it by connecting a
Voltage source as shown. The turns ratio is
1n, so v2 nv1. If a resistance R is connected
across the secondary terminals as shown, then the
current flowing in the secondary winding is
And the primary current is
i1
i2
1n


And the ratio of primary Voltage to current is
L1
L2
R
-
v2
v1
-
-
37
Ideal Transformers
This means that a resistor connected to the
secondary side can be reflected to the primary
side, as shown below. In fact, a whole resistive
circuit can be reflected from the secondary to
the primary, just by dividing each resistance by
n2. Resistances can be reflected in the opposite
direction, from the primary to the secondary, by
multiplying each resistance by n2. Reactances and
impedances can be reflected in the same way.

-
R
v1
-
-
38
Ideal Transformers
Transformers are often used to match a source
impedance, such as an amplifier output impedance,
to a load with a different impedance. Because
reactances can be reflected from the secondary
side to the primary side and vice-versa,
inductances and capacitances can be reflected as
well. If a resistance is reflected by
multiplying it by n2, an inductance is reflected
by multiplying it by n2 and a capacitance is
reflected by dividing it by n2. If a resistance
is reflected by dividing it by n2, an inductance
is reflected by dividing it by n2 and a
capacitance is reflected by multiplying it by n2.

-
R
v1
-
-
39
Ideal Transformers
This is illustrated below

-
C
v1
-
-

-
L
v1
-
-
40
RLC Combinations
Lets apply what weve learned to a circuit which
contains two types of elements. An RL circuit is
shown below, which represents (in a very
oversimplified way) a television deflection coil.
The desired waveform of the deflection current
i(t) is shown on the right, and wed like to find
the waveform of the excitation Voltage v(t) which
results in this current waveform. This is easier
than it sounds.
i
i(t)


-
v
t2

Im
-
-
t
t1
41
RLC Combinations
Because this is a series circuit, the same
current I flows in both the resistor and the
inductor. Applying KVL, we get
We can write an expression for the first period
of i(t)
i


i(t)
-
v
t2

Im
-
-
t
t1
42
RLC Combinations
So, in the expression we found for v(t), the
resistor term is
And the inductor term is
i


i(t)
-
v
t2

Im
-
-
t
t1
43
RLC Combinations
Summing the resistor and inductor terms yields
The Voltage waveform is shown below. Its the
same waveform
Stanley gives, but we obtained it here
analytically instead of graphically.
i
v(t)


-
v

-
-
t
t1