Summary of magnetostatics

1
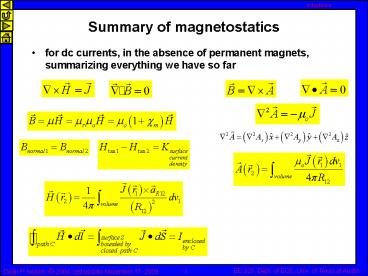
Summary of magnetostatics

• for dc currents, in the absence of permanent
  magnets, summarizing everything we have so far

2
Inductance

• for electric fields we had capacitance, that
  depending only on geometry and materials
  constants (er)
• capacitance was a way to link charge and voltage
• for magnetic fields we know we need current
  flowing
• inductance is a flux linkage concept
• ? is the total flux that links a circuit
• I is the current flowing in the circuit
• units ? is from the magnetic field, measured in
  webers
• L (units) weber / amp henry

3
Inductance

• in circuits, we already know the magnetic energy
  should be
• but the energy using field concepts is
• so the inductance is given by
• volume must include ALL the fields

4
Inductance

• now apply the divergence theorem

5
Example magnetic field from a current carrying
plate

• consider a conducting plate parallel to the x-y
  plane, t thick and w wide, carrying current I
• current density Jo I/(tw)
• a slab carrying current density Jo in the y
  direction produces an H-field in the x
  directions
• youll do this for homework! (its easy using
  Amperes Circuital Law)

6
Example magnetic field from current carrying
parallel plates

• consider two parallel plates, each t thick and w
  wide, one carrying current I, the other carrying
  the return current I, separated by a distance
  d
• now use superposition to get the total field from
  both plates

7
Example magnetic field from current carrying
parallel plates

• consider two parallel plates, each t thick and w
  wide, one carrying current I, the other carrying
  the return current I, separated by a distance
  d
• adding the fields from both plates we get

8
Example inductance of parallel plates

• consider two parallel plates, each t thick and w
  wide, l long, one carrying current I, the other
  carrying the return current I, separated by a
  distance d
• now use the energy method to find L
• here things are uniform with respect to y, so
  that part is really easy the dy integration just
  gives length l

9
Inductance of parallel plates region between the
plates

• lets do the region between the plates first

partial inductance (not self or mutual
inductance... to be defined shortly)
10
Inductance of parallel plates region inside
current carrying plate

• now lets do the region inside a plate

partial inductance (not self or mutual
inductance!)
11
Inductance of parallel plates region inside
current carrying plate

• so the total inductance is the sum of the
  region between the plate, and the regions inside
  the two plates

12
Special case Inductance of perfectly conducting
parallel plates

• if the conductivity of our metals was infinite
  (i.e., a perfect conductor) then all the
  current would have to on the surface
• why?
• because Ohms law would give us infinite current
• because inside a perfect conductor ALL fields
  (electric and magnetic) must be zero
• in this case we dont have to do any energy
  integrals for the inductance inside the
  conductors, so all we have left is the part form
  the gap between two plates
• what was the capacitance?
• interesting note product of inductance per unit
  length and capacitance per unit length

13
Example inductance of coaxial cable

• we found the magnetic field in coax from Amperes
  law

14
Internal inductance of inner conductor

• lets use the energy method, and do just the
  inner wire, of length l
• this has cylindrical symmetry
• recall volume element in cylindrical coords is
  z(rd?)dr
• here things are uniform with respect to z, so
  that part is really easy the dz integration just
  gives l

partial inductance
15
Inductance from field between the inner and outer
conductors

• looks about the same as before, just change H and
  B

partial inductance
16
Inductance from field in the outer conductor

• looks about the same as before, just change H and
  B

partial inductance
17
Inductance from field in the outer conductor

• looks about the same as before, just change H and
  B

partial inductance
18
Inductance of coax with uniform constant current
density

• to get the complete inductance we just need to
  add all the energy contributions
• since we used a problem with uniform current
  density, such as would be found using finite
  conductivity conductors at dc, this is the
  inductance (per unit length) of coax under those
  conditions

19
Special case coax with perfect conductors

• if the conductivity of our metals was infinite
  (i.e., a perfect conductor) then all the
  current would have to on the surface
• why?
• because Ohms law would give us infinite current
• because inside a perfect conductor ALL fields
  (electric and magnetic) must be zero
• in this case we dont have to do any energy
  integrals for the inductance inside the
  conductors, so all we have left is the part form
  the gap between inner and outer conductors
• what was the capacitance?
• interesting note product of inductance per unit
  length and capacitance per unit length

20
What we have for inductance

• note that the vector magnetic potential in the
  expression above is
• defined everywhere in the problem
• is due to ALL currents, everywhere in your world
• but of course, the current density can only be
  non-zero where conductors are present
• so the volume of the integration will only take
  place inside conductors
• but dont forget the vector potential comes from
  all the current elements, everywhere!
• it makes at least some sense to try to divide the
  problem up into
• part due to the current flowing inside the
  conductor you are standing in
• and part due to currents flowing in conductors
  somewhere else
• this leads to the idea of flux linkages
• between one part of a conductor and another part
  of the same conductor
• self inductance
• between two separate conductors (or better
  still, conducting loops)
• mutual inductance
• BUT dont forget you can only observe a
  complete loop, so you cant ever actually measure
  the self and mutual inductances separately

21
Mutual and self inductance

• let A1 be the vector magnetic potential produced
  by the current density J1 flowing in loop 1, A2
  be the vector magnetic potential produced by the
  current density J2 flowing in loop 2
• superposition holds, total vector magnetic
  potential A A1 A2
• the total inductance should be

22
Mutual and self inductance

• let A1 be the vector magnetic potential produced
  by the current density J1 flowing in loop 1, A2
  be the vector magnetic potential produced by the
  current density J2 flowing in loop 2
• the total inductance should be

self inductance of loop 2
self inductance of loop 1
mutual inductance current in 1, field from 2
mutual inductance current in 2, field from 1
23
Mutual and self inductance

• let A1 be the vector magnetic potential produced
  by the current density J1 flowing in loop 1, A2
  be the vector magnetic potential produced by the
  current density J2 flowing in loop 2
• the total inductance should be

self inductance of loop 2
self inductance of loop 1
mutual inductance current in 1, field from 2
mutual inductance current in 2, field from 1

• BUT dont forget you can only observe complete
  loops, so you cant ever actually measure the
  self and mutual inductances separately

24
Inductance formulas

• a good calculator with several geometries
  http//emcsun.ece.umr.edu/new-induct/

25
Summary of electrostatics and magnetostatics

• summarizing everything we have so far in the
  static case
• but what happens if something changes in time???

26
solenoid

• need this?

27
Magnetic fields

• applets
• http//links.math.rpi.edu/applets/appindex/magneti
  cfieldapplet.html
• wire and loop, induced current applet
  http//www.cco.caltech.edu/phys1/java/phys1/Induc
  tance/Inductance.html
• Faradys law applet http//webphysics.davidson.ed
  u/Applets/Faraday/intro.html