Turbulent oscillatory boundary layer flow

1
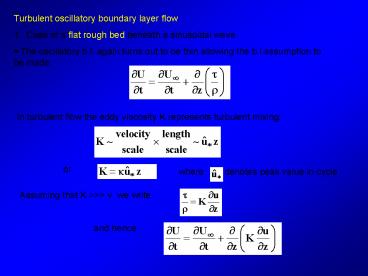
Turbulent oscillatory boundary layer flow 1.
Case of a flat rough bed beneath a sinusoidal
wave ? The oscillatory b.l. again turns out to be
thin allowing the b.l assumption to be made
In turbulent flow the eddy viscosity K represents
turbulent mixing
or
where denotes peak value in cycle
Assuming that K gtgtgt ? we write
and hence
2
We assume in what follows that the eddy viscosity
remains constant in time but increases linearly
with height. This represents the increase in the
turbulent mixing length scale with increasing
height. Boundary conditions We seek a
solution of the b.l. equation of the form
where ? Real Part. It follows that
New term c.f. laminar case
K now z-dependent
3
Writing we have
The solution is in terms of Kelvin functions
(Bessel functions of complex argument written ker
kei )
By the nature of the eddy viscosity assumption
involving the as-yet unknown we need
now to close the system by using the solution
for u itself.
4
With
the shear stress is given by and the peak bed
shear stress then becomes
This transcendental equation for may be
solved iteratively. The solution of J.D. Smith
(The Sea, 1977) is shown here Note. The
functions ker kei are tabulated (Abramowitz and
Stegun, 1964) and are available functions in
Matlab.
5
Once is known, the solution can be
evaluated example from Smith (1977) with U0100
cm/s, z010-3cm, T10s
time
Boundary layer thickness based on dimensional
arguments Overshoot region Logarithmic
near-bed region
The essential features of the earlier laminar
oscillatory b.l. solution are still present. The
turbulent b.l. thickness ?w is typically ?(10cm)
in the sea with a log-layer of maximum thickness
?w or ?(1cm) .
6
The phase lead of bed shear stress over
free-stream velocity is found by expressing ?0 in
the form
Phase lead
The phase lead turns out to be typically in the
range 15º-25º depending upon the value of
which, in turn, may be
expressed in terms of This phase lead is
substantially less than that found in laminar
flow (45º). This is due in part to the fact that
the existence of z0gt0 inhibits the full phase
lead to develop in rough turbulent flow.
7
2. Next consider case of height and time-varying
eddy viscosity The intensity of turbulence
varies through the wave cycle. This may be
represented using a time-varying eddy viscosity
(e.g. Trowbridge Madsen (1984) and Davies
(1986) for waves alone Malarkey Davies (1998)
for WC). Davies (1986) assumed that for a
sinusoidal free-stream flow given by
the eddy viscosity follows the free-stream
flow. The constant parameter ? determines the
amount of time variation (0 ? ? ? 1). As before
the governing equation is written
We seek an approximate solution of the
form Subject to b.c.s
8
Method of solution by normal modes Substitute
(2) and (4) into (3) and then match the
coefficients of the respective cosine and sine
terms. This yields the following system of
equations
To obtain approximate solutions, the Fourier
series can be truncated (N2 or N3 .).
9
The simplest case N2 then yields
The first two of these ordinary differential
equations can be solved for ?1 and ?1 using a
method of normal modes. In this simple case in
which the equations decouple The vertical
structure of the solutions for ?1 and ?1 mirror
the earlier ker kei solutions of Smith (1977).
10
The case N3, which is solved in the same manner,
is more complex since the coefficients of the
different frequency components now interact. It
is also physically more interesting.
? 0, 0.25, 0.5, 0.75 and 1
Typical example U0100cm/s, T10s,
z00.01cm Note the substantial effect of
time-variation in the eddy viscosity.
11
As in the earlier time-invariant case (?0) the
solution must again be closed. The
relationship obtained here for K0 depends upon ?.
12
The shear stress also depends upon ?
The bed shear stress shows the
increasing importance of the third harmonic (3?t)
as ? increases. The bed shear stress leads the
free-stream velocity for all ? values.

13
Phase lead of peak bed shear stress over peak
free-stream velocity
Wave friction factor fw obtained
from the approximate analytical solution in
comparison with some classical results. Note the
pronounced dependence on ?
14

• Consider now the case of a time-varying eddy
  viscosity K(z,t) in the case of oscillatory flow
  above a rippled bed. We here represent this 2D
  phenomenon within a 1DV framework (Davies
  Villaret, 1997, 1999).
• As a result of eddy shedding at flow
  reversal
• ? K(z,t) is more strongly time-varying than
  above a flat rough bed
• ? peaks in K(z,t) occur close to times of flow
  reversal
• ? K(z,t) is remains constant with height z in
  the bottom part of the flow
• ? K(z,t) is dominated by coherent, periodic
  motions and not by turbulence
• Again, assuming a free-stream flow given by
• the eddy viscosity may be written
• Here, in comparison with experimental data (e.g.
  Ranasoma, 1992) the coefficient ? in the time
  varying term turns out to be gt1.

15

• The solution to the problem may be obtained
  analytically
• either by using the earlier method of normal
  modes
• or by seeking a perturbation solution Davies
  Villaret, 1997, 1999
• In the case of purely symmetrical waves, both the
  shear stress t and horizontal velocity U contain
  only odd harmonics and can be expressed (using
  complex variables)

Note. Both of these quantities have been
horizontally averaged over one ripple
wavelength. In the light of the data of Ranasoma
and others, Davies Villaret (1997) truncated
these series at the 3rd harmonic for U, and at
the 5th harmonic for t (consistent with the
truncation of K(z,t) at the 2nd harmonic).
16
Substituting the truncated expressions for U and
t into
and matching the coefficients of ei?t, e3i?t and
e5i?t we may obtain coupled equations for U1 and
U3 as follows
complex conjugate
B.C.s
Davies and Villaret (1997) treated the solution
as a perturbation of the classical Stokes shear
wave solution and obtained
? vertical length scale characterising w.b.l.
17
Turbulent regions I. smooth II. Rough
III. Very rough (incl. rippled)
Laboratory data of Ranasoma (1992) obtained in
the laboratory over a fixed steeply rippled bed
(see also Ranasoma Sleath, 1992) Wave
measurements made using LDA confirmed the
dominance of coherent (eddy shedding) events over
normal turbulence.
Rippled bed data of Ranasoma (1992)
18
Comparisons between rippled bed 1DV model and
Ranasomas horizontally-averaged data Test 2a
with U0 20.3 cm/s T2.41 s ?10cm ?1.8cm
Velocity shear at z0.4cm above crest and its
representation up to 3rd harmonic Eddy
viscosity Free-stream flow
Momentum transfer Data and its representation up
to 5th harmonic Data and model results