Electric Current

1
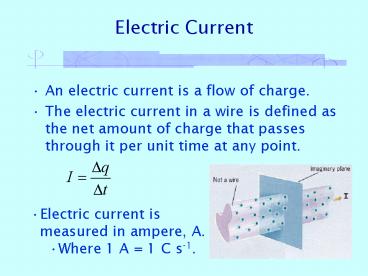
Electric Current

• An electric current is a flow of charge.
• The electric current in a wire is defined as the
  net amount of charge that passes through it per
  unit time at any point.

• Electric current is
• measured in ampere, A.
• Where 1 A 1 C s-1.

2
Conventional Current

• The direction of a Conventional current is the
  direction along which imaginary positivecharge
  carriers may be imagined to flow.
• In a wire, electrons are the only
  chargedparticles moving in an electrical
  current.

• At the right, negative
• charges moving to the left
• is equivalent to positive
• charges moving to the
• right.

3
Microscopic view of Electric Current

• In a conducting wire, the free electrons are
  moving about randomly at high speeds, (about
  1/1000 of the speed of light) bouncing off the
  atoms.
• Normally, the net flow of charge is zero.

4
The Mechanism of current flow (1)

• When an electric field exists in the wire, the
  electrons feel a force and begin to accelerate
  and gain kinetic energy.
• On colliding inelastically with lattice ions,the
  motion is repeated very rapidly at short time
  intervals.

• The electrons soon reach a steady speed known as
  their drift speed.

• The macroscopic effect is a steady current flow.

5
The Mechanism of current flow (2)

• Microscopically electric field energy is
  converted initially to the mechanical kinetic
  energy of the drifting electrons, and then to the
  kinetic energy and potential energy of the
  vibrating lattice ions.
• Macroscopically the internal energy of the metal
  increases resulting in a temperature rise.

6
Drift Speed (1)

• The diagram below shows part of a wire of
  cross-sectional area A.
• The current in the wire is I.
• There are n free electrons per m3 of the wire.
• The charge on each electron is e.
• The electrons move with a drift speed of v.
• It can be shown that
• I nAve

7
Drift Speed (2)

• The drift speed is normally (10-4 m s-1) very
  much smaller than the electrons average random
  speed (106 m s-1).
• For example, the drift speed through a copper
  wire of cross-sectional area 3.00 x 10-6 m2, with
  a current of 10 A will be approximately 2.5 x
  10-4 m/s.

8
Free Electron Number Density

• The table below shows some typical values for n.

9
Speed of Electric Signal

• The speed of the electric signal is the speed of
  light.  This means that, at the speed of light,
  the removal of one electron from one end of a
  long wire would affect electrons elsewhere. 
• If you think of a copper wire as a pipe
  completely filled with water, then forcing a drop
  of water in one end will result in a drop at the
  other end being pushed out very quickly. This is
  analogous to initiating an electric field in a
  conductor.

10
Electromotive Force (e.m.f.)

• The e.m.f. of an electric source is defined as
  the energy (chemical, mechanical or light, etc.)
  converted into electrical energy when unit charge
  passes through it.
• Unit volts (V)
• The e.m.f. equals the potential difference across
  the terminals of an electric source on open
  circuit.

11
Potential Difference

• The potential difference across two points in a
  circuit is defined as the energy converted from
  electrical energy to other forms of energy per
  unit charge passing between the points outside
  the source.

• V IR

12
Internal Resistance

• The resistance within a source of electric
  current such as a cell or generator is called the
  internal resistance.
• Some of the electrical energy is wasted due to
  the heating effect inside the cell.
• A real cell can be modelled as it had a perfect
  emf ? in series with a resistor r as shown.

13
Measurement of Internal Resistance

• The circuit below shows an experiment to measure
  the emf and internal resistance of a cell.

?
Slope - r
14
Variation of power output with external resistance
Power output to R is a maximum when R r,
internal resistance.
Pmax
r
15
Variation of efficiency with the external
resistance
The efficiency equals 50 when R r
100
50
r
16
Examples of Loads in an Electric Circuit (1)

• Loading for greatest power output is common in
  communication engineering.
• For example, the last transistor in a receiver
  delivers electrical power to the loudspeaker,
  which speaker converts into mechanical power as
  sound waves.
• To get the loudest sound, the speaker resistance
  (or impedance) is matched to the internal
  resistance (or impedance) of the transistor, so
  that maximum power is delivered to the speaker.

17
Examples of Loads in an Electric Circuit (2)

• The loading on a dynamo or battery is generally
  adjusted for high efficiency.
• If a large dynamo were used with a load not much
  greater than its internal resistance, the current
  would be so large that the heat generated would
  ruin the machine.
• With batteries and dynamos, the load resistance
  is made many times greater than the internal
  resistance.

18
Resistance in a Conductor (1)

• Notice that the electrons seem to be moving at
  the same speed in each one but there are many
  more electrons in the larger wire.  
• This results in a larger current which leads us
  to say that the resistance is less in a wire with
  a larger cross sectional area.

It can be shown that R?1/A.
19
Resistance in a Conductor (2)

• The length of a conductor is similar to the
  length of a hallway.  A shorter hallway would
  allow people to move through at a higher rate
  than a longer one.
• So a shorter conductor would allow electrons to
  move through at a higher rate than a longer one
  too.
• It can be shown that R ? l .

20
Resistivity of a material

• ? is called the resistivity of the material.

The unit of ? is ?m.
21
Resistivities of various materials
22
Effect of temperature on the resistance of a
metal conductor (1)

• Heat on the atomic or molecular scale is a
  direct representation of the vibration of the
  atoms or molecules.  Higher temperature means
  more vibrations.

• When the wire is cold the protons are not
  vibrating much so the electrons can run between
  them fairly rapidly. 

23
Effect of temperature on the resistance of a
metal conductor (2)

• As the conductor heats up, the protons start
  vibrating and moving slightly out of position. 
  As their motion becomes more erratic they are
  more likely to get in the way and disrupt the
  flow of the electrons. 

As a result, the higher the temperature, the
higher the resistance. 
24
The variation of Current with applied potential
difference (1)

• Filament lamp

• Ohmic conductor

25
The variation of Current with applied potential
difference (2)

• Thermionic diode

• Thermistor

26
The variation of Current with applied potential
difference (3)

• Electrolyte

• Gases

27
The variation of Current with applied potential
difference (4)

• Semiconductor diode

28
Slide-wire potentiometer

• The potentiometer consists of a long wire placed
  on a metre rule. A fixed potential difference is
  maintained across this wire by a cell E called
  the driver cell.

• A sliding contact is used to apply a fraction of
  this potential difference across another wire PQ,
  connected in parallel across AJ. The p.d. in this
  wire is then known to be equal to the p.d. across
  the part AJ of the potentiometer wire.

29
Rotary Potentiometer

• By rotating the wiper to touch the different
  places on the horse-shoe, we can 'tap-off' any
  fraction of the input voltage we want from zero
  up to the full size of the input.

30
Multimeters

• A multimeter is a moving-coil galvanometer
  adapted to measure current, p.d. and resistance.
• A rotary switch allows the various ranges to be
  chosen.

31
Connections in a Multimeter (1)

• For measuring current ranges, some internal
  resistors in parallel formed a shunt across the
  meter.
• For measuring p.d. ranges, more internal
  resistors in series formed a multiplier in series
  with the meter.

32
Connections in a Multimeter (2)

• For measuring resistance, an internal battery and
  rheostat are connected in series with the meter
  and the unknown resistance.
• To measure resistance the terminals are
  short-circuited and the rheostat adjusted until
  the pointer gives a full deflection, i.e. is on
  the zero of the ohms scale.
• The zero resistance reading will correspond to
  the maximum current value.