Radical and Rational Functions - PowerPoint PPT Presentation
Title:
Radical and Rational Functions
Description:
Evaluation of Radicals and Rational Functions – PowerPoint PPT presentation
Number of Views:22
Title: Radical and Rational Functions
1
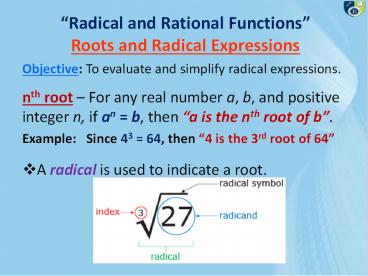
Radical and Rational Functions Roots and
Radical Expressions
- Objective To evaluate and simplify radical
expressions. - nth root For any real number a, b, and positive
integer n, if an b, then a is the nth root of
b. - Example Since 43 64, then 4 is the 3rd root
of 64 - A radical is used to indicate a root.
2
Evaluating Radicals
- Find each real number root.
- 1. 576 2. -64
- 3. 3 125 4. 3 -1000
- 5. 4 81 6. 5 -32
- Even roots of a positive number have two real
roots a positive and a negative. - The positive root is called the principal root.
3
Simplifying Radicals
1 4 9 16 25 36 49 64 81 100 121 144
- For every number a 0 and b 0,
- ?? ???? ?? ?? ?? ??
- 50
- 192
- 180
- 51
4
Simplifying Radicals
- ?? ???? ?? ?? ?? ??
- 3 54
- 3 40
- 3 375
5
Simplifying Radicals with Variables
- ?? 4 ?? 5
- ?? 7 ?? 6 ?? 9
- 24 ?? 11 ?? 8 ??
6
Simplifying Radicals with Variables
- 3 ?? 4 ?? 5
- 3 ?? 7 ?? 6 ?? 9
- 3 24 ?? 11 ?? 8 ??
7
Multiply Two Radicals
- 12 32
- 5 3 4 6
- 3 7?? 3 ?? 5 2 21?? 3 ?? 2
8
Multiply Two Radicals
- 3 3 3 9
- 2 3 4 5 3 6
- -3 3 25?? ?? 8 4 3 4 ?? 4 ?? 3
9
Dividing Two Radicals
500 5
49 16
3 250 ?? 7 ?? 3 3 2 ?? 3 ??
10
Rationalizing the Denominator
?? 2
5 ?? 4 ?? 2 ?? 2 ?? 3
3 5 3??
11
Adding and Subtracting Two Radicals
In order to add or subtract two radical
expression they must be LIKE RADICALS. Like
radicals have the same index and same radicand.
12
Adding Radicals
2 3 5 3
2 12 2
72 32 18
13
Subtracting Radicals
3 5 - 11 5
12 ?? - 3 ??
23 3 5 - 34 3 5
14
Multiplying Binomial Radicals
( 3 4) ( 2 -3)
(4 10 ) ( 10 - 3 )
( 5 7 ) ( 5 - 7 )
15
Rationalizing Binomial Denominators
5 1 3
4 - 2 4 2
5 2 6 - 3
16
Solving Radical Equations
- ?? -29
17
Solving Radical Equations
- 2 3??-4 6
18
Solving Radical Equations
- 10 - 2??1 5
19
Solving Radical Equations
- 2 5??1 -6 0
20
Solving Radical Equations
- 3 4?? 75
21
Solving Radical Equations
- 3 2?? -9 -3
22
Solving Radical Equations
- 3 4??5 -64
23
Solving Radical Equations
- ?? 3 2 734
24
Solving Radical Equations
- (4??-5) 3 2 16
25
Solving Radical Equations
- 3(??1) 3 4 -717
26
Solving Radical Equations
- When solving by taking an even root of both sides
you must include a . - (??-1) 2 3 25
27
Solving Radical Equations
- (3??8) 4 5 319
28
Solving Radical Equations
- extraneous solution is a solution of a derived
equation that is NOT a
solution of the original equation. - ?? ?? -2
29
Solving Radical Equations
- 5??-1 3??
30
Solving Radical Equations
- -3 ??2 2??
31
??1 2?? 5??3
?? 2?? 2
??25 ??5