Flow computation Formula - PowerPoint PPT Presentation
Title: Flow computation Formula
1
Chapter Three Flow computation
Formula
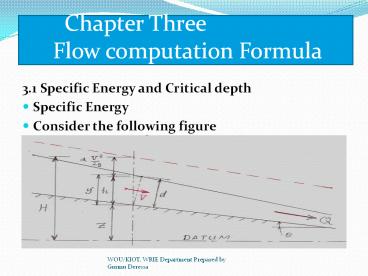
- 3.1 Specific Energy and Critical depth
- Specific Energy
- Consider the following figure
2
Chapter Three Flow computation
Formula
3
Chapter Three Flow computation
Formula
4
Chapter Three Flow computation
Formula
5
Chapter Three Flow computation
Formula
6
3.2 Critical Depth
- A minimum specific energy occurs at E Ec.
- The flow at this condition is known as the
critical state of flow and the depth
corresponding to this is known as the critical
depth.
7
3.2 Critical Depth
8
3.2 Critical Depth
9
3.3 Critical flow
- The characteristics of critical flow are
- The specific energy and specific force are
minimum for the given discharge. - The Froude number is equal to unity.
- For a given specific energy the discharge is
maximum at the critical flow. - The velocity head is equal to half the hydraulic
depth in a channel of small slope. - The velocity of flow in a channel of small slope
with uniform velocity distribution, is equal to
the celerity of small gravity waves ( ) C is
shallow water caused by local disturbance. - Flow at the critical state is unstable.
10
3.3 Critical flow
- Critical flow may occur at a particular section
or in the entire channel, then the flow in the
channel is called "Critical flow". - Yc f(A,D )for a given discharge.
- For a prismatic channel for a given discharge the
critical depth is constant at all sections of a
channel. - The bed slope which sustains a given discharge at
a uniform and critical depth is called "Critical
slope Sc". - A channel slope causing slower flow in sub
critical state for a given discharge is called
"sub critical slope or mild slope". A slope
greater than the critical slope is called steep
slope or super critical slope.
11
3.3 Critical flow
- For a given specific energy and discharge per
unit width q, there are two possible (real)
depths of flow, and that transition from one
depth to the other can be accomplished under
certain situations. - These two depths represented on the two different
limbs of the E-y curve separated by the crest c,
are characteristic of two different kinds of
flow a rational way to understand the nature of
the difference between them is to consider first
the flow represented by the point c. - Here the flow is in a critical condition, poised
between two alternative flow regimes, and indeed
the word critical " is used to describe this
state of flow it may be defined as the state at
which the specific energy E is a minimum for a
given q.
12
3.3 Critical flow
- Analytical Properties of Critical Flow
- Consider the Specific energy equation
in which y is the depth of flow andq is the
discharge per unit width.
Differentiating the above equation with respect
to y and equating to zero t can be written as
13
Analytical Properties of Critical Flow
The subscript c indicates critical flow
conditions. Thus the critical depth yc is a
function of discharge per unit width alone.
14
Analytical Properties of Critical Flow
- Further, the above equation it can be written as
15
Analytical Properties of Critical Flow
- The second derivative should be negative i.e
- The above equations are established by
considering the variation of specific energy with
y for a given q. - Clearly the curve will be of the general form as
shown in Figure.
16
Analytical Properties of Critical Flow
17
Analytical Properties of Critical Flow
- Variation of the Discharge with depth for a given
specific energy value - How q varies with y for a given E Eo?
- When y?E0and then q? 0. Similarly when y ?0,
q?0 and there will clearly be a maximum value of
q for some value of y between 0 and E0(y cannot
be greater than E0). - The relationship can be written as ( and
differentiating the above equation with respect
to y,
18
Analytical Properties of Critical Flow
19
Analytical Properties of Critical Flow
Alternative approach
Show that the flow is maximum when it is critical
flow for a given specific energy plot the graph "
E0 verses q
20
Alternative approach
21
Alternative approach
22
Alternative approach
- Which is essentially equation representing the
critical flow. - Thus critical flow cannotes not only minimum
specific energy for a given discharge per unit
width, but also maximum discharge per unit width
for given specific energy. - Any one of the above three equations may be used
to define critical flow
23
Alternative approach
Any one of the above three equations may be used
to define critical flow
24
Alternative approach
25
Quiz(10)
- Show that for a trapezoidal channel the mininimum
specific energy EC is related to critical depth
Yc as
Where,
26
1 The section factor for critical
flow computation
- The section factor for critical flow computation
(Z) is the product of the water area and the
square root of the hydraulic depth.
27
1 The section factor for critical
flow computation
- For critical flow ,
- by substituting
28
1 The section factor for critical
flow computation
Where Qc represent the discharge that would make
the depth y critical and know as the critical
discharge. When the energy coefficient is not
assumed to be unity
29
1 The section factor for critical
flow computation
- Eq. (3.1 and 3.2) are very useful tool for the
computation and analysis of critical flow in open
channel, when the discharge is given the
equations will give the critical section factor
(Zc), and hence the critical Depth yc. - Section factor (Z) for different channel section
shape is given as
30
1 The section factor for critical
flow computation
- To simplify the computation of critical flow,
dimensionless curves showing the relation between
the depth and the section factor Z have been
prepared for different type of channels sections.
- These self-explanatory curves will help to
determine the depth y for a given section factor
Z and vice versa.
31
1 computationComputation of
critical flow
- Computation of critical flow involves the
determination of critical depth and velocity when
the discharge and channel section are known. - The methods illustrated by examples are given
below. On the other hand ,if critical depth and
channel section are known, the critical discharge
can be determined from the relation
32
1 computationComputation of
critical flow
- Algebraic Method.
For geometrically simple channel sections, the
uniform-flow condition may be determined by an
algebraic solution, as illustrated by the
following example
33
1 computationComputation of
critical flow
- Example For a trapezoidal channel with base
width b6.0m, side slope x 2(i.e. 1vertical2
horizontal) and Mannings n 0.02, calculate the
critical velocity, criticaldepth and critical
slope if its discharge Q17m3/s. - Solution
34
1 computationComputation of
critical flow
35
1 computationComputation of
critical flow
- By trial and error for value of YC
- YC 0.87m
36
1 computationComputation of
critical flow
37
QUESTIONS
CRITICAL FLOW
38
QUESTIONS
CRITICAL FLOW
39
B.Graphical method
- For channels of complicated cross section and
variable flow conditions, a graphical solution of
the problem is found to be convenient. - Method of design chart
- The design chart for determining the critical
depth can be used with great expediency.
40
The Section Factor for Uniform-flow Computation
- '11h expression AR2/3 is called the section
factor for uniform-flow computation It IS an
important element in the computation of uniform
flow. - this factor may be expressed as
41
The Section Factor for Uniform-flow Computation
- This equation applies to a channel section when
the flow is uniform. - The right side of the equation contains the
values of n, Q, and S but the left side depends
only on the geometry of the water area. - Therefore, it shows that, for a given condition
of n,Q, and S, there is only one possible depth
for maintaining a uniform flow, provided that the
value of AR2/3 is always increases with increase
in depth, which is true in most cases
42
The Section Factor for Uniform-flow Computation
- In order to simplify the computation,
dimensionless curves showing the relation between
depth and section fuctor AR2/3 (Fig. 6-1) have
been prepared for rectangular,. trapezoidal,
and cirpular channel' sections. - These self-explanatory curves will help to
determine, the depth for a given section factor
AR2/3, and vice versa.
43
The Section Factor for Uniform-flow Computation
44
Control section
- Control of flow in open channel or at structure
means the establishment of explicit relationship
(one to one relationship) between the stage
(water level) and the discharge of flow. - When the control of channel is achieved at
certain part of channel or structure, this
section is called control section.
45
Control section
- Holding fixed stage-discharge relationship,
control section is always suitable for gauging
station since it is always suitable site for
developing the discharge rating curve, a curve
representing depth-discharge relationship. - At critical state of flow a definite stage
discharge relationship can be established and
represented by the equation
46
Control section
- This section equation is theoretically
independent of the channel roughness and other
uncontrolled circumstances. - Therefore, critical flow section is a control
section. - For Further knowledge read the open channel
hydraulics books.
47
Flow measurement
- It was mentioned in the preceding article that,
at critical control section, the relationship
between the depth and discharge is explicit
independent of the channel roughness and other
uncontrolled circumstances such explicit
relationship between stage-discharge
relationship offers a theoretical basis for the
measurement of discharge in open channels.
48
Flow measurement
- Based on the principle of critical flow, various
devices for flow measurement have been developed.
- In such devices the critical depth is created by
developing low hump on the channel bottom such as
a weir or by contraction in the cross section,
such as the critical flow flume(venture flume
or par shall flume) in the transition part. - The use of weir is a simple method, but it causes
high head loss. - If water contains suspended particles, some will
be deposited in the pool at upstream of the weir,
resulting in gradual
49
Flow measurement
- change in discharge coefficient. These
difficulties, however, can be overcome by at
least partially by the use of critical flow
flume.
50
Channel with a Hump
- a) Subcritical Flow
- Consider a horizontal, frictionless rectangular
channel of width B carrying discharge Q at depth
y1. - Let the flow be subcritical. At a section 2
(Fig.3. 1) a smooth hump of heights ?Z is built
on the floor. - Since there are no energy losses between
sections 1 and 2, construction of a hump causes
the specific energy at section to decrease by
Z. - Thus the specific energies at sections 1 and 2
are,
51
Channel with a Hump
52
Channel with a Hump
- Since the flow is subcritical, the water surface
will drop due to a decrease in the specific
energy. - In Fig. (3.2), the water surface which was at P
at section 1 will come down to point R at section
2. The depth y2 will be given by,
53
Channel with a Hump
Figure 3.2. Specific energy diagram for Fig.
(3.1)
54
Channel with a Hump
- It is easy to see from Fig. (3.2) that as the
value of ? Z is increased, the depth at section
2, or y2 , will decrease. - The minimum depth is reached when the point R
coincides with C, the critical depth. - At this point the hump height will be maximum,
?Zmax , y2 yc critical depth, and E2 Ec
minimum energy for the flowing discharge Q. - The condition at ?Zmax is given by the rela tion
55
Channel with a Hump
The question may arise as to what happens when
?Z gt ? Zmax. From Fig. (3.2) it is seen that
the flow is not possible with the given
conditions (given discharge). The upstream
depth has to increase to cause and increase in
the specific energy at section 1. If this
modified depth is represented by
56
Channel with a Hump
At section 2 the flow will continue at the
minimum specific energy level, i.e. at the
critical condition. At this condition, y2 yc ,
and
57
(No Transcript)
58
Channel with a Hump
while y2 will continue to remain yc). The
variation of y1 and y2 with ?Z in the
subcritical regime can be clearly seen in Fig.3.3
Recollecting the various sequences, when 0 lt ? Z
lt ?Zmax the upstream water level remains
stationary at y1 while the depth of flow at
section 2 decreases with ?Z reaching a minimum
value of yc at ?Z ?Zmax . (Fig.3.2). with
further increase in the value of ?Z, (i.e., for
?Z gt?Zmax, y1will change to y1
59
b) Supercritical Flow
- If y1 is in the supercritical flow regime Fig
(3.2) shows that the depth of flow increases due
to the reduction of specific energy. - In Fig (3.2) point P corresponds to y1 and point
R to depth at the section 2. - Up to the critical depth, y2 increases to reach
yc at ?Z ? Zmax For ? Z gt ?Zmax, the depth
over the hump y2 yc will remain constant and
the max upstream depth y1 will change. - It will decrease to have a higher specific energy
E1 by increasing velocity V1. - The variation of the depths y1 and y2 with ?Z
in the supercritical flow is shown in Fig. (3.4).
60
Example 3.3
- A rectangular channel has a width of 2.0 m and
carries a discharge of 4.80m /sec with a depth of
1.60 m. At a certain cross-section a small,
smooth hump with a flat top and a height 0.10 m
is proposed to be built. - a). Calculate the likely change in the water
surface. Neglect the energy loss. - b). If the height of the hump is 0.50 m,
estimate the water surface elevation on the hump
and at a section upstream of the hump
61
Solution
62
Solution
At section 2
it show the upstream flow is subcritical and hump
will cause a drop in the water surface elevation.
Let the suffixes 1 and 2 refer to the upstream
and downstream sections respectively as shown in
the fig
63
Solution
64
Solution
65
solution
- The drop at water surface elevation is
66
solution
67
solution
- The minimum specific energy required at section 2
is greater than E2, (Ec21.26 gtE21.215), the
available specific energy at that section . - Hence , the depth at section 2 will be at the
critical depth and E2Ec21.26m. - The upstream depth y1 will increase to a depth y1
such that the new specific energy at the upstream
section 1 is
68
Uniform flow
- Uniform flow in open channel has the following
main features - The depth, water area, velocity and discharge at
every section of the channel are constant. - The energy line, water surface and channel
bottom are all parallel i.e their slopes are
all equal
69
Uniform flow
- Uniform flow is considered to be steady only,
since unsteady uniform flow is practically
nonexistent. - In natural streams, even steady uniform flow is
rare, for rivers and streams in natural state
scarcely ever experience a strict uniform flow
condition. - Despite this deviation from the truth, the
uniform flow condition is frequently assumed in
the computation of flow in natural streams. - The results obtained from this assumption are
understood to be appropriate and general,but they
offer a relatively simple and satisfactory
solution to many practical problems.
70
Establishment of uniform flow
- When flow occurs in open channel resistance is
encountered by the water as it flows downstream. - This resistance generally counteracted by the
components of gravity forces acting on the body
of lthe water in the direction of motion . - A uniform flow will be developed if the the
resistance is balanced by the gravity forces,
example the head loss due to turbulent flow is
exactly balanced by the reduction of in potential
energy due to the uniform decrease in the
elevation of the channel. - The magnitude of the resistance, when other
physical factors of the channel are kept
unchanged, depends on the velocity of flow.
71
Establishment of uniform flow
- If the water enters the channel slowly,the
velocity and hence the resistance are small and
the resistance is out balanced by gravity
forces,resulting in an accelerating flow in the
upsteam reach. - The velocity and the resistance gradually
increase until a balance between resistance and
garavity fdorce is reached. - At this moment and afterwards the flow become
uniform. - The upstream rreach isrequired for establishment
of uniform flow is known as the the transitory
zone.In this zone the flow is accelerating and
varied. - .
72
Establishment of uniform flow
- If the channel is shorter than the transitory
length required by the given conditions, - uniform flow could not be attained.
- Towards the end of the channel the resistance may
again exceeded by the gravity forces and the flow
become varied. - Ingeneral,uniform flow can acquire only in very
long ,straight,prismatic channels where terminal
velocity of can be achieved.For purpose of
explanation a long channel is channel is shown
with three different slopes (figures given during
your lecture or refer ventechow)subcritical,criti
cal and supercritical
73
Establishment of uniform flow
- At the subcritical slope the water surface in the
transitory zone appears adulatory. - The flow is uniforn in the middle reach of
channel but varied at the two ends.At the
critidcal slope middle sketch). - The water surface of critical flow is un stable.
Possible undulation may occur in the middle reach
but on the average the depth is constant and the
flow may be considered uniform.
74
Establishment of uniform flow
- At the super critical slope the transitory waater
surface passess from the subcritical stage t o
the super critical stage through gradual
hydraulic drop. - Beyond the transitory zone the flow is
approaching uniformidy. - The depth of uniform flow is called normal depth.
- In the figures the long dashed lines represents
the normal depth line abbreviated as N.D.L and
the short dashed lines represents the dcritical
depth line or C.D.L.
75
Expressing the Velocity of a Uniform Flow.
- For hydraulic computations the mean velocity of a
turbulent uniform flow in open channels is
usually expressed approximately by a so-called
uniform-flow formula. - Most practical uniform-flow formula can be
expressed in the following general form
76
Expressing the Velocity of a Uniform Flow.
- where
- V is t,he mean velocity in m/s R is the
hydraulic radius m S is the energy slope, x and
yare exponents and, C is a factor of flow
resistance varying with the mean velocity,
hydraulic channel roughness, viscosity, and many
other factors.
77
(No Transcript)
78
(No Transcript)
79
(No Transcript)
80
(No Transcript)
81
(No Transcript)
82
READING ASSIGNMENT
- Refer reference books chow for other resistance
formula.
83
Computation of uniform flow
84
Computation of uniform flow
85
Computation of uniform flow
86
(No Transcript)
87
(No Transcript)
88
(No Transcript)
89
(No Transcript)
90
Computation of uniform flow
- In computation of uniform flow there are two
common problems to solve
91
solution
92
(No Transcript)
93
solution
94
(No Transcript)
95
QUESTIONS
UNIFORM FLOW
96
QUESTIONS
UNIFORM FLOW
97
(No Transcript)
98
Best( economic) hydraulic section
99
Best( economic) hydraulic section
100
Best( economic) hydraulic section
101
Best( economic) hydraulic section
102
Best( economic) hydraulic section
103
Best( economic) hydraulic section
104
trapezoidal
- Example
105
Exercise
- A trapezoidal, concrete lined channel (Mannings
n0.015) is to be constructed to carry flood
water. The slope of the channel bed slope is 1 in
500.The design discharge is 10m3/s. - calculate the proportion of the trapezoidal
channel that will minimize excavation and result
in optimum hydraulic section. - If the cross sectional area kept the same as that
of part (a) but for safety reasons depth of flow
is limited to1m,what will be the discharge now
take side slope1v2H.
106
(No Transcript)
107
The end!
- Thank you!