Vertical and Horizontal Translations - PowerPoint PPT Presentation
1 / 21
Title:
Vertical and Horizontal Translations
Description:
Use the graph of. to obtain the graphs of. HORIZONTAL TRANSLATIONS ... Reflections about the x-Axis ... a, the graph of the new function y=af(x) is obtained ... – PowerPoint PPT presentation
Number of Views:183
Avg rating:3.0/5.0
Title: Vertical and Horizontal Translations
1
Vertical and Horizontal Translations
2
(No Transcript)
3
(2, 6)
(1, 3)
(2, 4)
(1, 1)
(0, 2)
(0, 0)
4
(No Transcript)
5
(No Transcript)
6
(2, 4)
(0, 0)
(1, 1)
(2, 1)
(1, -2)
(0, -3)
7
Vertical Shifts
- cgt0
- The graph of g(x) f(x) c is the same as the
graph of f(x) but shifted UP by c. - The graph of g(x) f(x) - c is the same as the
graph of f(x) but shifted DOWN by c.
8
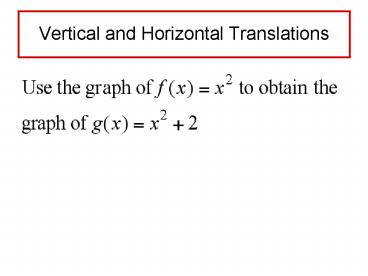
- Use the graph of
- to obtain the graphs of
9
HORIZONTAL TRANSLATIONS
10
Horizontal Shifts
- The graph of is the
graph of shifted h units to the left. - The graph of is the
graph of - shifted h units to the right.
11
The graph below is obtained by translating the
graph of . Find a formula for the
function graphed.
12
The graph below is obtained by translating the
graph of . Find a formula for the
function graphed.
13
The graph below is obtained by translating the
graph of . Find a formula for the
function graphed.
14
Reflections about the x-Axis and the y-Axis
- The graph of y - f(x) is obtained by
reflecting the graph of f(x) about the x-axis. - The graph of y f(-x) is obtained by reflecting
the graph of f(x) about the y-axis.
15
(No Transcript)
16
Vertical Compression
- When the right side of a function yf(x) is
multiplied by a positive number a, the graph of
the new function yaf(x) is obtained by
multiplying each y-coordinate on the graph of
yf(x) by a. The new graph is a vertically
compressed (if 0 lt a lt 1) or a vertically
stretched (if a gt 1) version of the graph of
yf(x).
17
(No Transcript)
18
(No Transcript)
19
Horizontal Compression
If the argument x of a function yf(x) is
multiplied by a positive number a, the graph of
the new function yf(ax) is obtained by
multiplying each x-coordinate of yf(x) by
1/a. A horizontal compression results if agt1,
and a horizontal stretch occurs if 0 lt a lt 1.
20
yx²-1 y(2x)²-1 y((1/3)x)²-1 (1,0) ((1/2),0)
(3,0) (-1,0) (((-1)/2),0) (-3,0) (0,-1) (0,-1)
(0,-1)
21
(No Transcript)