Chemical Arithmetic

1
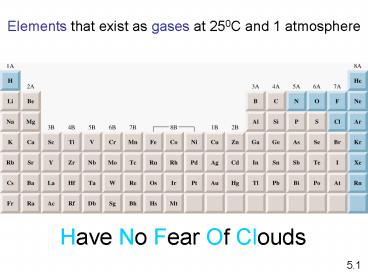
Elements that exist as gases at 250C and 1
atmosphere
Have No Fear Of Clouds
5.1
2
5.1
3
Microscopic View of Molecular Solids, Liquids and
Gases

• Not looking at ionic substances, or metals or
  network covalent substances or atomic substances
• Gaseous state - molecules are in constant rapid
  motion, are widely separated, are not attracted
  to each other and when they collide they bounce
  off each other
• Liquid state - molecules are moving slowly, are
  close to each other, have attractions to each
  other that are constantly forming and breaking
• Solid state - molecules are close together, have
  strong attractions for each other, are locked
  into position and only have vibrational motion

4
Transitions between states

• Addition of energy to the molecules, usually as
  heat (increase in temperature), causes transition
  to more dispersed state (state with more kinetic
  energy motion)
• Melting ice, boiling water
• Removal of energy from the molecules through
  cooling causes transition to more organized state
  (state with more potential energy attractions)
• Freezing water, condensing steam

5
Gases - Kinetic Molecular Theory

• The molecules do not attract or repel each other.
• Each molecule behaves as an independent particle.
• The average distance between the molecules is
  very large in comparison to the size of a
  molecule.
• Most of the container is empty space.
• BUT gases can be compressed
• The average speed of the molecules increases as
  the temperature increases.
• Gas molecules are in constant, random motion,
  colliding with each other and the walls of the
  container.

6
Pressure macro and microscopic

• Pressure - microscopic level (from Kinetic
  Molecular Theory)
• number and power of collisions of the molecules
  with the walls of the container (including where
  the gauge is located) per second
• small gas molecules move around faster and hit
  the sides of the container more often but with
  low power larger gas molecules move slower, hit
  less frequently but hit with more power
• Pressure - macroscopic level (observable)
• Force exerted per unit area
• Pressure of different shoes
• Force felt at different depths
• In water
• In air

7
Pressure
(force mass x acceleration)
Units of Pressure
1 pascal (Pa) 1 N/m2 1 atm 760 mmHg 760
torr 1 atm 101,325 Pa
5.2
8
10 miles
0.2 atm
4 miles
0.5 atm
Sea level
1 atm
5.2
9
Figure 5.4
5.2
10
As P (h) increases
V decreases
5.3
11
Pressure - mathematical

• Mathematical what can be quantified and
  compared
• Pressure constant x ½mv2
• Units
• lbs/in2 (everyday units) 1 atmosphere (atm)
  760 mm Hg (scientific units) Pascals
• For a fixed amount of any gas under the same
  conditions, the pressure is the same Ideal Gas
  Law
• the differences in power and speed exactly cancel
  each other
• 20 hits x 2 push units/hit 40 push units
• 10 hits x 4 push units/hit 40 push units
• 5 hits x 8 push units/hit 40 push units

12
Common Sense Observations about Gases

• Pressure gauges on hard wall containers
• the more gas you put in, the higher the pressure

P (pressure)
n (moles)

• Pressure cookers
• the higher the temperature, the higher the
  pressure

P (pressure)
T (temperature)

• Pistons or Syringes
• the pressure increases as the piston is pushed in

P (pressure)
V (volume)
13
Absolute Ideal Gas Law
PV nRT

• P Pressure (atmospheres)
• V Volume (Liters)
• n number of moles (moles)
• R gas constant 0.082 L-atm/mole-K
• T Temperature in K (oC 273)
• Sense of the equation
• fits common sense observations
• For equation to work correct units have to be used

14
Using the Absolute Ideal Gas Law

• Start with the memorized equation

PV nRT

• Rearrange to solve for unknown component

• Change each of the quantities to the correct
  units (if needed)
• Insert values and calculate (convert back to oC
  if required)
• Does your answer make sense!!!!

15
Generalized Relative Ideal Gas Law

• Rearrange ideal gas law to comparative form

PV nRT

• Since any set of conditions for a certain gas is
  equal to a constant we can compare two different
  conditions for any gas

• For the relative law, units are not as important
  as long as they are consistent BUT temperature
  must always be in K

16
Relative Gas Laws

• The generalized relative gas law can be
  simplified depending on what variables are
  present in the problem
• Consider a problem that involves only pressure
  and volume

• Start with the generalized law then cross out
  variables that are not in the problem
• Rearrange the variables to solve for the unknown
  component
• Add the values that are known (convert T to units
  of K if needed)
• Do math (convert back to oC if you have to)
• Does your answer make sense!!!!

17
Solving gas law problems

• Figuring out which equation to use
• Relative cues
• Look for two volumes, or two pressures or two
  temperatures or two number of moles
• Look for change or new or will be
• Absolute cues
• Only one of each variable given
• R often given
• Often if g of gas given (need to get to moles)
• Reducing/rearranging equation to get unknown
• Changing all variables to appropriate units that
  can be used in equations
• Always have to use K
• Absolute always have to use L, atm, moles
• Relative can use different volume and pressure
  units as long as consistent

18
Example 1 of using strategy

• Problem What is the volume, in milliliters,
  occupied by 89.2 g CO2 (g) at 37 ºC and 737 mmHg?
• Solution
• Cues
• g of CO2 ?absolute
• Only one of each variable ? absolute
• Equation need volume ? V nRT/P
• Conversions
• 89.2 g of CO2 x 1 mole CO2/44.0 g CO2 2.03
  moles
• 37 273 310 K
• 737/760 0.970 atm
• R 0.082 L-atm/mole-K
• Substitute and solve V 2.03x0.082x310/.970
  53.1 L

19
Example 2 of using strategy

• Problem A sample of gas has a volume of 4.25 L
  at 25.6 ºC and 748 mmHg. What will be the volume
  of this gas at 26.8 ºC and 742 mmHg? Solution
• Cues
• Will be ? relative
• Two temperature two pressures
• Reduce equation (moles constant) ? P1V1/n1T1
  P2V2/n2T2
• Conversions
• T1 25.6 273 298.6 K T2 26.8 273
  299.8 K
• Can use pressure values as given and L value
• Substitute and solve P1V1/T1 P2V2/T2
• (748)(4.25)/298.6 (742)V2/299.8
• ALGEBRA!!!!
• V2 4.30 L

20
Some more problems 1

• A light bulb with an internal pressure of 720.
  torr at 20ºC is thrown into an incinerator
  operating at 750ºC. What internal pressure must
  the light bulb be able to withstand if it does
  not break?
• Relative P and T only make sure convert T to K
• A 12.8-L cylinder contains 35.8 g O2 at 46 ºC.
  What is the pressure of this gas, in atmospheres?
• Absolute convert g O2 to moles convert T to K

21
Some more problems 2

• A 72.8-L constant-volume cylinder containing 1.85
  mol He is heated until the pressure reaches 3.50
  atm. What is the final temperature in degrees
  Celsius?
• Absolute only one of each OK to go ? get T
• BUT ? convert back to oC
• If a 1500.-mL sample of air at 22 ºC is cooled
  enough to cause the volume to decrease to 750. mL
  at constant pressure, what is the final Celsius
  temperature required? What was the temperature
  change?
• Relative Two volumes V (use mL) and T only
  convert T to K
• Get K answer ? convert back to oC also get
  temperature change

22
Gas Stoichiometry

• Stoichiometry with gases obeys the same rules as
  stoichiometry with g moles molarity
• Usually relationships in equations in which gas
  is consumed or produced
• Two general problems
• Information about gases known and information
  about other components in equation sought
• Information about other components known and
  information about gases in equation sought
• Can expand switching yard!!

23
Gas Stoichiometry
PV nRT
24
How many mg of magnesium metal must react with
excess HCl(aq) to produce 28.50 mL of H2(g),
measured at 26 ºC and 758 Torr? Mg (s) 2 HCl
(aq) ? H2 (g) MgCl2 (aq)
PV/RT? moles of H2 ? moles of Mg ? g of Mg
n 1.16 x10-3 moles H2
1.16 x10-3 moles H2
1 mole Mg 1 mole H2
1.16 x10-3 moles Mg
24.3 g Mg 1 mole Mg
0.0282 g Mg
28.2 mg Mg
0.0282 g Mg
25
What volume of CO2 (g) is produced at a
temperature of 20. ºC and a pressure of 1.00 atm
by the fermentation of 500. g of glucose?
C6H12O6 ? 2 C2H5OH 2 CO2 (g)
g of glucose ?moles of glucose ? moles of CO2 ?V
of CO2 ( PV/RT)
V 134 L
5.56 mole CO2
2 mole CO2 1 mole glucose
2.78 mole glucose
1 mole glucose 180 g glucose
500 g glucose
0.0282 g Mg
26
A 3.57-g sample of a KCl-KClO3 mixture is
decomposed by heating and produces 119 mL O2 (g),
measured at 22.4 ºC and 738 mmHg. What is the
mass percent of KClO3 in the mixture? 2 KClO3
(s) ? 2 KCl(s) 3 O2 (g)
Moles of O2 ? moles of KClO3 ? g of KClO3 ?
KClO3 of 3.57g
n 4.77 x10-3 moles O2
4.77 x 10-3 mole O2
2 mole KClO3 3 mole O2
3.18 x 10-3 mole KClO3
122.6 g KClO3 1 mole KClO3
0.390 g/3.57 g x 100
10.9
0.390 g KClO3
0.0282 g Mg
27
Complex gas stoichiometry

• How many liters of SO3(g) can be produced by the
  reaction of 1.15 L SO2(g) and 0.65 L O2(g) if all
  three gases are measured at the same temperature
  and pressure?
• 2 SO2(g) O2(g) ? 2 SO3(g)
• Since R is a constant the term RT/P is a constant
  since we are not changing temperature and
  pressure
• We can therefore compare volumes in balanced
  equations just like we can compare moles
• This only applies if T and P are constant
• This is a limiting reagent problem
• SO2 O2
• HAVE 1.15 L 0.65 L
• NEED 2(0.65)L ½ (1.15)L
• 1.30 L 0.575 L
• SO2 is the limiting reagent since we need 1.30 L
  to react with O2 but only have 1.15 L. Therefore
  we will form the same amount of SO3 gas
• 1.15 L