Rock Engineering Basics

1
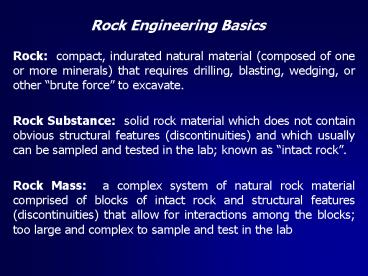
Rock Engineering Basics

• Rock compact, indurated natural material
  (composed of one or more minerals) that requires
  drilling, blasting, wedging, or other brute
  force to excavate.
• Rock Substance solid rock material which does
  not contain obvious structural features
  (discontinuities) and which usually can be
  sampled and tested in the lab known as intact
  rock.
• Rock Mass a complex system of natural rock
  material comprised of blocks of intact rock and
  structural features (discontinuities) that allow
  for interactions among the blocks too large and
  complex to sample and test in the lab

2
(No Transcript)
3
Geologic Info for Rock Slope Engineering

• 1. Geologic mapping of formations and units
  needed to
• generate surface-geology maps and
  cross-sections
•  
• 2. Site topography and proposed cut-slope
  geometries (best to display cross-sections 11
  with no vertical exaggeration)
•  

4
(No Transcript)
5
Geologic Info for Rock Slope Engineering

• 1. Geologic mapping of formations and units
  needed to
• generate surface-geology maps and
  cross-sections
•  
• 2. Site topography and proposed cut-slope
  geometries (best to display cross-sections 11
  with no vertical exaggeration)
•  
• 3. Relevant rock-strength data for the rock
  substance
•  
• 4. Engineering properties of rock
  discontinuities, including
• orientation, geometry, shear strength
• 5. Groundwater regime (water table, piez. head
  distributions)

6
(No Transcript)
7
(No Transcript)
8
(No Transcript)
9
Uniaxial Compressive Strength

•  
• A cylinder of rock taken from drill-core is cut
  square on the ends, then the ends are ground
  smooth, and the specimen loaded to failure in a
  testing machine. The length-to-diameter ratio
  (L/d) typically ranges between 2 and 3.
•  
• UCS Pf / A (stress units of psi, psf,
  MPa, tsm)
• where Pf ultimate failure load (at
  rupture)
• A cross-sectional area of the
  cylindrical specimen
• pd2/4

10
Reporting of UCS Standardized Results

• Empirical corrections of the tested value of UCS
  to standardized Ld values are given below
• For Ld of 21
•  
• UCS21 UCS / 0.88 0.24(d/L)
• For Ld of 11
• UCS11 UCS / 0.778 0.222(d/L)

11
Point Load Index

• The point load test is conducted on a piece of
  drill core (with ragged ends) with L/d gt 1.5
  whereby the core piece is loaded perpendicular to
  the core axis between cone-shaped platens until
  failure occurs and the core is split. The core
  diameter and instrument gage pressure at failure
  are recorded. The Point Load Index then is given
  by
•  
• PtL Pg(Ar) / d2
• where d core diameter, Pg instrument gage
  pressure at specimen failure, and Ar
  cross-sectional area of instrument loading ram.

12
(No Transcript)
13
(No Transcript)
14
Using PtL to Estimate UCS

•  
• UCS ? PtL(14 0.175d)
• for d measured in units of mm
• For typical core diameters (47 61 mm), use
  the
• approximation
• UCS ? 23(PtL)

15
Estimating UCS Using a Schmidt Hammer

• A Schmidt Type-L rebound hammer can be used to
  approximate the UCS. A reasonable estimate of
  the rock unit weight also is needed.
•  
• Rebound measurements often are quite variable, so
  the field investigation should include at least
  10 measurements at a given sampling site (for
  averaging purposes).

16
Brazilian Disk Tension Testing

•  
• A small disk of rock core with known diameter (d)
  and thickness (h) is loaded along its diameter to
  induce an apparent tensile stress field and cause
  the disk to rupture. The tensile strength then
  is given by
•  
• T 2(Pf) / (pdh)
• where Pf failure load at which the disk
  ruptured
•  
• A general rule-of-thumb (10 x T) ? UCS

17
Mapping Display of Discontinuity Data

• Field mapping methods to obtain information on
  discontinuity orientations, spacing, length,
  roughness, etc.
• Scanline mapping detailed mapping of individual
  discontin-uities that intersect a designated
  mapping line or linear window

18
(No Transcript)
19
(No Transcript)
20
Mapping Display of Discontinuity Data

• Field mapping methods to obtain information on
  discontinuity orientations, spacing, length,
  roughness, etc.
• Scanline mapping detailed mapping of individual
  discontin-uities that intersect a designated
  mapping line or linear window
• Fracture-Set mapping (Cell mapping) mapping of
  fracture-set properties observed within
  user-defined cells on the rock exposure

21
Mapping Display of Discontinuity Data

• Field mapping methods to obtain information on
  discontinuity orientations, spacing, length,
  roughness, etc.
• Scanline mapping detailed mapping of individual
  discontin-uities that intersect a designated
  mapping line or linear window
• Fracture-Set mapping (Cell mapping) mapping of
  fracture-set properties observed within
  user-defined cells on the rock exposure
• Oriented core logging mapping of oriented drill
  core to obtain orientations, fracture spacings,
  roughness

22
(No Transcript)
23
(No Transcript)
24
(No Transcript)
25
(No Transcript)
26
(No Transcript)
27
Display of Discontinuity Orientations

• The orientations of planar discontinuities are
  best displayed and evaluated by plotting their
  poles (normals) on lower-hemisphere stereographic
  projections (known as stereonet plots). A
  cluster of such poles then represents a fracture
  set having planes in similar orientations.

28
Display of Discontinuity Orientations

• Poles near the center of the stereonet are for
  shallow-dipping (fairly flat) fractures, and
  poles near the outer edge of the stereonet are
  for steeply dipping fractures.
• Thus, a cluster of fracture poles in the
  upper-right portion of the lower-hemisphere
  stereonet plot indicates a fracture set with
  planes dipping toward the southwest.

29
Shear Strength Modeling for Discontinuities

• 1. Linear Mohr-Coulomb failure envelope with
  y-intercept (known as cohesion) and slope (known
  as the coefficient of friction, tanf)
•  
• t c sn tanf
•  
• where t shear strength along the
  discontinuity
• sn effective normal stress acting on the
  discontinuity
• c cohesion (generally equal to zero or a very
  small
• value for clean rock fractures)
• f friction angle.

30
Shear Strength Modeling for Discontinuities

• 2. General nonlinear, power-curve model
•  
• t c a(sn )b
•  
• where t shear strength along the
  discontinuity
• sn effective normal stress acting on the
  discontinuity
• a, b, c power-curve parameters.
•  
• Note that when b 1.0, this model reduces to a
  linear model with the parameter a tanf.
  Therefore, this general model also covers the
  special case of the linear model.

31
Shear Strength Modeling for Discontinuities

• 3. JRC model of shear strength (nonlinear model)
•  
• t sn tan(JRC)log10(JCS/sn) fb
•  
• where t shear strength along the
  discontinuity
• sn effective normal stress acting on the
  discontinuity
• JRC joint roughness coefficient (typ. values
  2 to 6)
• JCS joint-wall compressive strength (UCS of
  intact rock)
• fb base friction angle (i.e., for saw-cut,
  smooth surfaces).

32
Shear Strength Modeling for Discontinuities

• 4. Back-analysis of a rock-slope failure with
  well-defined geometry and groundwater conditions
•  
• We set the FOS equal to 1.0, and
  back-calculate the corresponding combinations of
  f and waviness that seem appropriate (linear
  shear-strength model with zero cohesion). We can
  follow the same approach with the JRC model of
  shear strength (select appropriate values of fb,
  JCS, and JRC that give FOS 1.0).

33
Shear Strength

• Analysis of Laboratory Direct-Shear Data
•  
• During the laboratory direct-shear test of a
  natural
• rock joint, data are collected to record the
  shear load
• as a function of the applied normal load and the
  shear
• displacement. The graph of shear load vs. shear
• displacement for each applied normal load
  provides
• the basis for describing the shear strength of
  the
• specimen.
•  

34
(No Transcript)
35
 

• Laboratory Direct-Shear Data
•  
• The contact area in shear when the specimen
  attains either the
• peak shear load or the residual shear load is
  needed to
• calculate the corresponding normal stress and
  shear stress
• (strength) for any particular graph trace
  (trial).
•  
• For circular or rectangular specimens, this
  contact area can be
• calculated directly, once the pertinent shear
  displacement is
• identified. For irregularly shaped specimens, a
  reference table
• must be constructed that displays the contact
  area as a
• function of shear displacement.
•  

36
 

• Laboratory Direct-Shear Data
•  
• A least-squares regression program (such as
  Taussm
• or the Mathcad sheet entitled TauRegr) then
• provides the linear and power models for shear
• strength, as shown in the typical plots of shear
• strength on the overheads 

37
 

• Overall Shear Strength for Highly Fractured
• Rock Masses
•  
• Exponential RQD Method
• Required input
• Average RQD (Rock Quality Designation) of
  the
• rock mass ()
• Estimated c (psi) and f for intact rock
• Estimated c (psi) and f for natural fractures
• Intermediate factors (weights)
• A .475exp(.007 x RQD) B .188exp(.013
  x RQD)
• Then
• cm cr (B2) cf (1-B2) in psi
• fm fr (A2) ff (1-A2) in deg.

38
 

•  
• 2. Hoek-Brown Rock Mass Strength Model
• Required input
• mi - Hoek-Brown constant (a material
  constant
• ranging from about 4 to 33)
• GSI - Geological Strength Index (see handout)
• Ci - uniaxial compressive strength of intact
  rock
• D - estimated rock-mass disturbance factor (0
  for
• insitu rock or for carefully designed blasting
  
• programs 1 for poor blasting practices with
• considerable overbreak)
• See Mathcad calculation sheet for examples.