? ~ 0?? [u(x,y)/Ue] (1 - PowerPoint PPT Presentation
1 / 32
Title:
? ~ 0?? [u(x,y)/Ue] (1
Description:
MOMENTUM INTEGRAL EQUATION ASSUMPTIONS: steady, incompressible, two-dimensional no body forces, p = p(x) in boundary layer, d – PowerPoint PPT presentation
Number of Views:79
Avg rating:3.0/5.0
Title: ? ~ 0?? [u(x,y)/Ue] (1
1
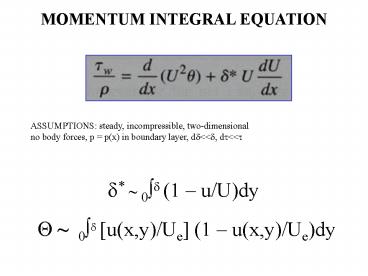
MOMENTUM INTEGRAL EQUATION
ASSUMPTIONS steady, incompressible,
two-dimensional no body forces, p p(x) in
boundary layer, d?ltlt?, d?ltlt?
? 0?? (1 u/U)dy
? 0?? u(x,y)/Ue (1 u(x,y)/Ue)dy
2
(No Transcript)
3
(plate is 2 thick, RexL 10,000 air bubbles
in water)
For flat plate with dP/dx 0, dU/dx 0
4
Realize (like Blasius) that u/U similar for all x
when plotted as a function of y/ ?.
Substitutions ? y/? so dy/? d?
Not f(x)
- y/?
- 0 when y0
- 1 when y ?
u/U
? y/?
5
f(?)
6
? 0.133 for Blasius exact solution, laminar,
dp/dx 0
u/U f(?)
Strategy obtain an expression for ?w as a
function of ?, and solve for ?(x)
7
Laminar Flow Over a Flat Plate, dp/dx 0
Want to know ?w(x)
Assume velocity profile u a by
cy2 B.C. at y 0 u 0 so a 0
at y ? u U so U b? c?2 at
y ? ?u/?y 0 so 0 b 2c? and b -2c?
U -2c?2 c?2 -c?2 so c -U/?2 u
-2c?y (U/?2) y2 2U?y/?2 (U/?2) y2 u/U
2(y/?) (y/?)2 Let y/? ? u/U 2? -?2
Strategy obtain an expression for ?w as a
function of ?, and solve for ?(x)
8
Laminar Flow Over a Flat Plate, dp/dx 0
u/U 2? -?2
Strategy obtain an expression for ?w as a
function of ?, and solve for ?(x)
9
?w 2?U/?
u/U 2? -?2
2? - 4?2 2?3 - ?2 2?3 - ?4
Strategy obtain an expression for ?w as a
function of ?, and solve for ?(x)
10
2?U/(??U2) (d?/dx) (?2 (5/3)?3 ?4
(1/5)?5)01 2?U/(??U2) (d?/dx) (1 5/3 1
1/5) (d?/dx) (2/15)
Assuming ? 0 at x 0, then c 0
?2/2 15?x/(?U)
Strategy obtain an expression for ?w as a
function of ?, and solve for ?(x)
11
?2/2 15?x/(?U) ?2/x2 30?/(?Ux) 30
Rex ?/x 5.5 (Rex)-1/2 ? ? x1/2
Strategy obtain an expression for ?w as a
function of ?, and solve for ?(x)
12
(No Transcript)
13
Three unknowns, A, B, and C- will need three
boundary conditions. What are they?
14
y
15
(No Transcript)
16
u/U sin(?/2)(?)
17
(No Transcript)
18
(?/? 0.344)
19
0.344
20
LAMINAR VELOCITY PROFILES dp/dx 0
21
y / ?
u / U
Sinusoidal, parabolic, cubic look similar to
Blasius solution.
22
(No Transcript)
23
FLAT PLATE dp/dx 0 TURBULENT FLOW u/U
(y/?)1/7 for pipe had u/U (y/R)1/7
?1/7 But du/dy infinity, so use ?w from pipe
for a u/U (y/R)1/7 profile ?w
0.0233?U2?/(RU)1/4 Replace Umax with Ue U and
R with ? to get for flat plate ?w
0.0233?U2?/(? U)1/4
24
u/Umax (y/?)1/7
u/Ue 2(y/?) (y/?)2
25
FLAT PLATE dp/dx 0 TURBULENT FLOW
u/U (y/?)1/7 ?w 0.0233?U2?/(? U)1/4
Cf skin friction coefficient ?w/( ½ ?U2) Cf
0.0466 ?/(? U)1/4
CD Drag coefficient FD/(½?U2A)
??wdA/(½?U2A) (1/A)?CfdA
26
FLAT PLATE dp/dx0 TURBULENT FLOW
u/U (y/?)1/7 ?w
0.0233?U2?/(? U)1/4 ?w ?U2
(d?/dx) ?01?1/7(1- ?1/7)d?
?U2 (d?/dx) (1/(8/7) 1/(9/7)) ?U2
(d?/dx) (63-56)/72 ?U2 (d?/dx) (7/72)
27
FLAT PLATE dp/dx0 TURBULENT FLOW
u/U (y/?)1/7
- 0.0233?U2?/(? U)1/4 ?U2 (d?/dx) (7/72)
- ?1/4d? 0.240 (?/U)1/4dx
- (4/5) ?5/4 0.240 (?/U)1/4 x c
- Assume turbulent boundary layer begins at x0
- Then ? 0 at x 0, so c 0
- 0.382 (?/U)1/5 x4/5 (x/x)1/5
- /x 0.382 (?/Ux)1/5 0.382/Rex1/5
28
FLAT PLATE dp/dx0 TURBULENT FLOW
u/U (y/?)1/7
- /x 0.382 (?/Ux)1/5 0.382/Rex1/5
Cf skin friction coefficient ?w/( ½ ?U2) Cf
0.0466 ?/(? U)1/4
Cf 0.0594/Rex1/5
29
(No Transcript)
30
Favorable Pressure Gradient ?p/?x lt 0 U
increasing with x
Unfavorable Pressure Gradient ?p/?x gt 0 U
decreasing with x When velocity just above
surface 0, then flow will separate causes
wake.
Gravity workingagainst friction
Gravity working with friction
31
Favorable Pressure Gradient ?p/?x lt 0 U
increasing with x
Unfavorable Pressure Gradient ?p/?x gt 0 U
decreasing with x When velocity just above
surface 0, then flow will separate causes
wake.
Gravity workingagainst friction
Gravity working with friction
32
Favorable Pressure Gradient (dp/dxlt0), flow will
never separate. Unfavorable Pressure Gradient
(dp/dxgt0), flow may separate. No Pressure
Gradient (dp/dx 0), flow will never separate.
Logic for flow to separate the velocity just
above the wall must be equal to zero uydy uo
?u/?y?y0 ?u/?y?y0 0 for flow
separation ?w ??u/?y?y0 Laminar Flow
?w(x)/(?U2) constant/Re1/2 flat plate
dp/dx0 Turbulent Flow ?w(x)/(?U2)
constant/Re1/5 flat plate dp/dx0 For both
laminar and turbulent ?w is always positive so
?u/?y?y0 is always greater than 0, so uydy is
always greater than zero