FUNCTION TRANSFORMATIONS - PowerPoint PPT Presentation
1 / 20
Title:
FUNCTION TRANSFORMATIONS
Description:
FUNCTION TRANSFORMATIONS I can translate linear and quadratic functions along a vector. I can reflect linear and quadratic functions across the x-axis, y-axis, y = x ... – PowerPoint PPT presentation
Number of Views:378
Avg rating:3.0/5.0
Title: FUNCTION TRANSFORMATIONS
1
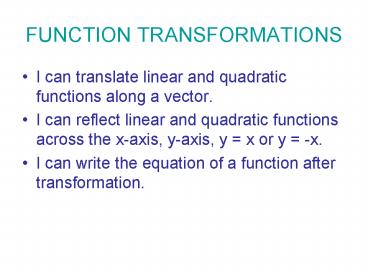
FUNCTION TRANSFORMATIONS
- I can translate linear and quadratic functions
along a vector. - I can reflect linear and quadratic functions
across the x-axis, y-axis, y x or y -x. - I can write the equation of a function after
transformation.
2
- Graph y x2
x
y
Must plot a minimum of 5 points no
calculator!!!
3
- Graph y x2 3
x
y
Must plot a minimum of 5 points no
calculator!!!
4
- Graph y x2 1
x
y
Must plot a minimum of 5 points no
calculator!!!
5
- Graph y -x2
x
y
Must plot a minimum of 5 points no
calculator!!!
6
- Graph y -x2 1
x
y
Must plot a minimum of 5 points no
calculator!!!
7
- Reflect the function y x across the y-axis
New Equation
8
- Reflect the function y 2/3x -2 across the
x-axis
New Equation
9
- Reflect the function y x2 2 across the x-axis
x
y
New Equation
10
- Reflect the function y -2x 1 across the line
y x
11
- Reflect the function y x2 2 across the y x
x
y
12
- Translate the function y 3x 1 along lt-1,3gt
13
- Translate the function y x2 2 along lt2,-4gt
x
y
14
COMPOSITIONS OF TRANSFORMATIONS
- I can perform a composition of two or more
transformations.
15
Compositions of Transformations
- Putting two or more transformations together
- ex Reflect across the y-axis, then
rotate 90 - ORDER MATTERS
16
Example 1B Drawing Compositions of Isometries
Draw the result of the composition of isometries.
?KLM has vertices K(4, 1), L(5, 2), and M(1,
4). Rotate ?KLM 180 about the origin and then
reflect it across the y-axis.
17
Example 1B Continued
Step 1 The rotational image of (x, y) is (x,
y).
K(4, 1) ? K(4, 1), L(5, 2) ? L(5, 2), and
M(1, 4) ? M(1, 4).
Step 2 The reflection image of (x, y) is (x, y).
K(4, 1) ? K(4, 1), L(5, 2) ? L(5, 2), and
M(1, 4) ? M(1, 4).
Step 3 Graph the image and preimages.
18
Check It Out! Example 1
?JKL has vertices J(1,2), K(4, 2), and L(3, 0).
Reflect ?JKL across the y-axis and then rotate it
180 about the origin.
19
Check It Out! Example 1 Continued
Step 1 The reflection image of (x, y) is (x, y).
Step 2 The rotational image of (x, y) is (x,
y).
Step 3 Graph the image and preimages.
20
Lesson Quiz
PQR has vertices P(5, 2), Q(1, 4), and P(3, 3).
1. Translate ?PQR along the vector lt2, 1gt and
then reflect it across the x-axis.
P(3, 1), Q(1, 3), R(5, 4)
2. Reflect ?PQR across the line y x and then
rotate it 90 about the origin.
P(5, 2), Q(1, 4), R(3, 3)