Exponential Distribution.

1
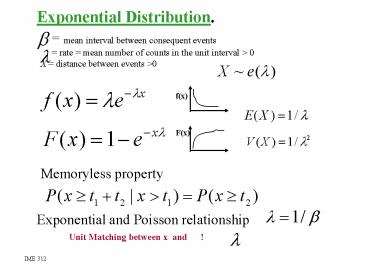
Exponential Distribution.
mean interval between consequent events
rate mean number of counts in the unit
interval gt 0 X distance between events gt0
f(x)
F(x)
Memoryless property
Exponential and Poisson relationship
Unit Matching between x and !
IME 312
2
Exponential Dist. Poisson Dist.
IME 312
3
Relation betweenExponential distribution ?
Poisson distribution Xi Continuous random
variable, time between arrivals, has
Exponential distribution with mean 1/4

X11/4 X21/2
X31/4 X41/8 X51/8 X61/2
X71/4 X81/4
X91/8 X101/8 X113/8 X121/8
0
100

200

300
Y13

Y24

Y35
Yi Discrete random variable, number of
arrivals per unit of time, has Poisson
distribution with mean 4. (rate4) Y
Poisson (4)
IME 301 and 312
4
Continuous Uniform Distribution


f(x)
a
b
F(x)
a
b
IME 312
5

• Gamma Distribution
• K shape parameter gt0
• scale parameter gt0

For Gamma Function, you can use and if K is
integer (k) then
IME 312
6

• Application of Gamma Distribution
• K shape parameter gt0 number of Yi added
• scale parameter gt0 rate
• if X Expo ( )
• and Y X1 X2 Xk
• then Y Gamma (k, )
• i.e. Y is the time taken for K events to occur
  and X is the time between two consecutive events
  to occur

IME 312
7
Relation betweenExponential distribution ?
Gamma distribution Xi Continuous random
variable, time between arrivals, has
Exponential distribution with mean 1/4

X11/4 X21/2
X31/4 X41/8 X51/8 X61/2
X71/4 X81/4
X91/8 X101/8 X113/8 X121/8
0
100

200

300




Y11
Y27/8
Y31/2
Y43/4
Yi Continuous random variable, time taken for
3 customers to arrive, has Gamma
distribution with shape parameter k 3 and
scale4
IME 312
8

• Weibull Distribution
• a shape parameter gt0
• scale parameter gt0
• 
  for
• 
  for
• 
  
  

IME 312
9
Normal Distribution
Standard Normal Use the table in
the Appendix
IME 312
10

• Normal Approximation to the Binomial
• Use Normal for Binominal if n is large XBinomial
  (n, p)

Refer to page 262
IME 312
11
Central Limit Theorem
random sample from a
population with and
sample
mean Then
has standard normal distribution N(0,
1) as commonly
IME 312
12

• What does Central Limit Theorem mean?
• Consider any distribution (uniform, exponential,
  normal, or ). Assume that the distribution has a
  mean of and a standard deviation of
  .
• Pick up a sample of size n from this
  distribution. Assume the values of variables are
• Calculate the mean of this sample . Repeat
  this process and find many sample means. Then our
  sample means will have a normal distribution with
  a mean of and a standard deviation of
  .

IME 312
13
degrees of freedom
probability Distribution Definition
Notation Chi-Square t dist. F
dist. Where
IME 312