Chapter 12. Light scattering (determination of MW without calibration)

1
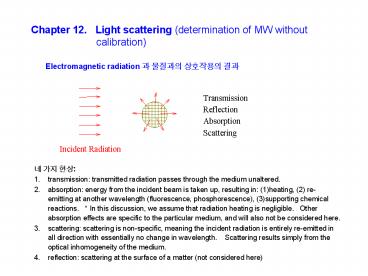
Chapter 12. Light scattering (determination of
MW without calibration)
Electromagnetic radiation ? ???? ????? ??

• ? ?? ??
• transmission transmitted radiation passes
  through the medium unaltered.
• absorption energy from the incident beam is
  taken up, resulting in (1)heating, (2)
  re-emitting at another wavelength (fluorescence,
  phosphorescence), (3)supporting chemical
  reactions. In this discussion, we assume that
  radiation heating is negligible. Other
  absorption effects are specific to the particular
  medium, and will also not be considered here.
• scattering scattering is non-specific, meaning
  the incident radiation is entirely re-emitted in
  all direction with essentially no change in
  wavelength. Scattering results simply from the
  optical inhomogeneity of the medium.
• reflection scattering at the surface of a matter
  (not considered here)

2

• Now we focus on the light scattering.
• Application of Light Scattering for Analysis
• Classical Light Scattering (CLS) or Static Light
  Scattering (SLS)
• Dynamic Light Scattering (DLS, QELS, PCS)
• CLS
• ?? Scattering center small volumes of material
  that scatters light. ? individual molecule in
  a gas.
• Consequences of the interaction of the beam with
  the scattering center depends, among other
  things, on the ratio of the size of the
  scattering center to the incident wavelength
  (?o). Our primary interest is the case where
  the radius of the scattering center, a, is much
  smaller than the wavelength of the incident light
  (a lt 0.05?o, less than 5 of ?o). This
  condition is satisfied by dissolved polymer coils
  of moderate molar mass radiated by VISIBLE light.
  When the oscillating electric field of the
  incident beam interacts with the scattering
  center, it induces a synchronous oscillating
  dipole, which re-emits the electromagnetic energy
  in all directions. Scattering under these
  circumstances is called Rayleigh scattering.
  The light which is not scattered is transmitted
  , where Is and It are the
  intensity of the scattered and transmitted light,
  respectively.

3

• Oscillating electric field of incident beam
  interacts with scattering center, induces a
  synchronous oscillating dipole, which re-emits
  electromagnetic energy in all directions.

Rayleigh scattering? ?? ???? ??? ?? ??? ?? ???
(1cos2?)? ????, scattering center? observer???
??(r)? ??? ???.

• 1944, Debye

• Rearrange

Constant, K
?o ?????, dn/dc refractive index
increment no ??? refractive index, p???,
c????g/mL
4
I? is inversely proportional to ?o. Shorter
wavelength scatters more than longer
wavelength Assume system is dilute, the net
signal at the point of observation is sum of all
scattering intensities from individual scatterer
- no multiple scattering (scattered light from
one center strike another center causing
re-scattering, etc.).

• Two ways to access the light scattering
  information experimentally
• Turbidimeter (or spectrophotometer)
• Light scattering

5
1. Turbidimeter experiment (Transmitted light
intensity, It is measured)
Solution is dilute, so higher order concentration
terms can be ignored.
6
Procedure Measure t at various conc. ? Plot Hc/T
vs. c (straight line) ? Determine M from
intercept, 2nd virial coeff., B from slope
7
2. Light Scattering experiment (measure I? at
certain ? and r)
?6? ?4? ??
??? ??? ? 5 (?/20) ??? ??? ??? Rayleigh
limit
8
?-condition?? ???0.
9
lt??gt For polydisperse sample, Turbidity (?? light
scattering) is contributed by molecules of
different MW. Define ti ??? Mi? ?? ???? ??
turbidity ?
??? turbidity? light scattering???? ?? ????
weight-average MW??.

10
Rayleigh-Gans-Debye (RGD scattering) when the
scattering centers are larger than Rayleigh limit
Different part of more extended domain (B)
produce scattered light which interferes with
that produced by other part (A) - constructive or
destructive
11
Distribution is symmetrical for small particles
(lt?/20). For larger particles, intensity is
reduced at all angles except zero.
Contributions from two scattering centers can be
summed to give the net scattering intensity.
The result is a net reduction of the scattered
intensity
P? "shape factor" or "form factor"
Always P? lt 1, function of size and shape of
scattering volume. Now we start seeing the
angle dependence of the scattered light !
12

• p(?) decreases with ?.
• p(?) decreases more for higher MW.

13
Effect of MW and Chain Conformation on P?, and on
measured MW at 90o.
Conformation MW (g/mol) RG (nm) P(90o) MW(90o)
Random coil
Polystyrene 51K 8 0.98 51K
Polystyrene( ? condition) 420K 19 0.95 400K
PMMA 680K 36 0.70 480K
Polyisoprene(70 cis) 940K 48 0.56 530K
Spherical
Bovine serum albumin 66K 3 1.00 66K
Bushy stunt virus 10700K 12 0.98 10500K
Rod shaped
Poly- -benzyl-L-glutamate 130K 26 0.91 118K
Myosin 493K 47 0.74 365K
DNA 4000K 117 0.35 1400K
14
Final Rayleigh equation for random coil polymer
? ?? ?? ??
Case 1 ??0
Plot Kc/R? vs. c y-??1/M, ???2A2
Case 2 c?0
Plot Kc/R? vs. sin2(?/2) y-??1/M, ???
(16p2/3M?2) rg2
Three information!
15
??? ?? ?? (1) ??? ??? ???? R???. (2) Kc/R? vs.
c, Kc/R? vs. sin2(? /2) plot ??. (3) ? 0 ? c
0 ? extrapolate.
Kc/R? vs. sin2(? /2)
Kc/R? vs. c
Zimm plot
??? ? ?? ???. ? ? extrapolated points
16
Cases
1. Small polymers ????? ??. (Horizontal line)
Zimm plot for PMMA in butanone ?o546 nm, 25?, no
1.348, dn/dc 0.112 cm3/g
- ?? ???? ??? ???. - Mw ? A2 ?? ?? - ???? ?? ???.
2. Small polymers in ?-solvent ?? ? ?? ??? ??.
Zimm plot of poly(2-hydroxyethyl methacrylate) in
isopropanol ?o436 nm, 25?, no 1.391, dn/dc
0.125 cm3/g
?-solvent A20? ?? ??, ???-???, ???-????? ?????
???? ??, ????? ?? ??.

• Calculated values Mw 66,000 g/mol
• A2 0 mol
  cm3/g2
• - Kc/R? at small angles fall mostly below the
  horizontal line plotted through the points from
  medium and large angles.

17
3. Larger polymers in good solvent ?? ? ??? ??.
Zimm plot of polystyrene in toluene ?o546 nm,
25?, no 1.498, dn/dc 0.110 cm3/g
- ??? ? 2x105 ??? ??, Kc/R? ? ?? ??? (A2??)?
???. - Athermal Condition - No effect of
temperature on polymer structure
4. Polymers in poor solvent A2 ? ??? ? (? ???
? ? ??. ? ?? ?? ?? ??)
Zimm plot of polybutadiene in dioxane ?o546 nm,
25?, no 1.422, dn/dc 0.110 cm3/g

• - ?????? ??? ?? (nonlinear).
• - ?? microgel, ??, aggregate? ?? ? ?? ??.
• Curve-fitting? ??? ??.
• ???? ??? ???? ??.
• ??? ??? good solvent???
• ???? ??? ? ??.

18
Stand-alone vs. On-line MALS

• ltStand-alone modegt
• Stand-alone mode LS instrument is used itself.
  
• Zimm plot ? ?? M, A2, R?? ??

• ltOn-line modegt
• LS instrument is used as a detector for a
  separator.
• c0 ?? ??.
• ? slice? ?? Kc/R? vs. sin2(?/2) ???? ??,
  y-?????? ??? (M), ???????? rg? ??. y-??1/M,
  ????? (16p2/3M?2) rg2
• ? slice? monodisperse??? ???? ?????? ????? ??.
  ??? ?? ???? ??? (?????? ? ?????? ???).

• Average Molecular Weights
• No-average Mn(Sci)/(S(ci/Mi))
• Wt-average MwS(ci Mi)/ S(ci)
• Z-average Mz S(ci Mi2)/S(ci/Mi)
• Average Sizes (mean square radii)
• No-average ltrg2gtn S(ci/Mi)ltrg2gti/S(ci/Mi)
• Wt-average ltrg2gtw S(ciltrg2gti)/Sci
• Z-average ltrg2gtzS(ciMiltrg2gti)/S(ciMi)

19
Light scattering instruments MALLS (Multi Angle
Laser Light Scattering) I? is measured at 15
angles (1) Stand-alone mode Measure scattered
light at different angles for different
concentrations ? Make a Zimm plot ? Determine M,
B, Rg
Assuming each slice is narrow distribution, Mw ?
Mi Average M can be calculated. It is therefore
very important to have a good resolution.
20

21
Angular Dependence of Kc / R?(?? high molecular
weight DNA)
22
Effect of Particles/Gels on Light Scattering
Measurement Note the delicacy of extrapolation to
zero angle from larger distances.
23

• DALLS (Dual Angle) I?? is measured at 15o and
  90o
• LALLS (Low Angle) I? is measured at one low
  angle (assume ?? 0)
• Static mode measure LS at a few c ? Plot Kc/R?
  vs. c ? Determine M and B from intercept and
  slope.
• On-line mode determine Kc/R? for each slice (
  calculate M). Considering each slice is
  narrow distribution, let Mw ( Mi, from which
  average MW's can be calculated (as learned in
  chapter 1). It is therefore again very
  important to have a good resolution.

• RALLS (Right Angle)
• I? is measured at 90o.
• Simple design
• Higher S/N ratio, Application is limited to
  cases where P? is close to 1 (e.g., less than
  200K of linear random polymer)
• RALLS combined with differential viscometer
  (commercially available from Viscotek, "TRISEC")

24
ltTRISEC ?? ??gt Assume P? 1 and A2 0.
Determine Mest.
? is determined by differential viscometer, and
M determined in step 2.
Calculate new MW by
Go to step 2. Repeat until Mest does not change.
25
ltLight scattering experiment? ??? ???gt
?? K? B? ??? ?? parameter? ?? ?? ??. ???
??? ?? ? ?? ??? ??.

1. n ??? refractive index
2. dn/dc Specific refractive index increment
3. B 2nd virial coefficient (Static mode??? B? ???
   ?? ??? ? ?? ??? Static mode? ??).

26
1. ??? Refractive Index ?? ?? ??? ?? RI ??? ???
??.
?? ??? ??? (R?? ???? ?)
Solvent RI R? x 106 cm-1
Carbon disulfide 1.6207 57.5
a-chloronaphthalene (140 oC) 1.5323 52.8
1,2,4-Trichlorobenzene (135 oC) 1.502 35.7
Chlorobenzene 1.5187 18.6
o-Xylene (35 oC) 1.50 15.5
Toluene 1.49 14.1
Benzene 1.50 12.6
Chloroform 1.444 6.9
Methylene chloride 1.4223 6.3
Carbon tetrachloride 1.46 6.2
Dimethyl formamide 1.43 (589 nm) 5.6
Cyclohexane 1.425 5.1
Cyclohexanone 1.4466 4.7
Methyl ethyl ketone 1.38 4.5
Ethyle acetate 1.37 4.4
THF 1.41 4.4
Acetone 1.36 4.3
Dimethyl sulfoxide 1.478 (589 nm) 4.1
Methanol 1.33 2.9
Water 1.33 1.2

• Except where otherwise noted, all measurements
  made at ? 632.8 nm and T23 oC. RI at 632.8 nm
  calculated by extrapolation from values measured
  at other wavelengths.
• Extrapolation? ?? reference Johnson, B. L.
  Smith, J. "Light Scattering from Polymer
  solutions" Huglin, M. B. ed., Academic press, New
  York, 1972, pp 27

27
2. Specific refractive Index, dn/dc

• ???? ?? ? ?? (Polymer Handbook, Huglin, ed.,
  Light Scattering from Polymer Solutions, Academic
  Press, 1972)
• ???? ?? ? ?? ?? ??? ?? ??
• Conventional method
• DRI? ??
• ? ?? ?? ???? (n2-n1)? ?? (recommended conc. 2,
  3, 4, 5 x 10-3 g/mL) ? (n2-n1)/c2 vs. vs. c2?
  plot ? zero concentration?? extrapolate ? dn/dc?
  intercept? ? ? ???.

28
For concentration ranges generally used, the
refractive index difference, n2-n1, is a linear
function of concentration. In other words,
(n2-n1)/c2 is constant. ? (n2-n1)/c2 vs. c2 ????
???0.
This means that (n2-n1) needs to be measured for
only one or two different concentrations. If
(n2-n1)/c2 shows no significant dependence on c,
then dn/dc can be obtained by averaging
(n2-n1)/c2 values
29

• SEC/RI? ??
• ?? ?? ?? ??

?? dn/dc? ?? ????? ???? kR? ??

• ???? ??? ?? ?? ? ?? ?? estimate? ? ?? ??.
• extrapolate to desired wavelength

?? 2) polymer? ??? refractive index? ??
estimate
???? n2? polymer? partial specific volume
mL/g??. ?? n2 ? 1.

• lt????gt
• dn/dc ? ??? ????? light scattering ??? ?? ??? ???
  ??? ?? ???? ???? ??.
• Dn/dc ? ??? ????? ???? ??? ??. Dn/dc ? ???? ??.
• ??? dn/dc ?? ??. ???? ???? ?? ??? ??.

30
3. Virial Coefficient, B or A2

• ???? ?? ? ?? (? Polymer Handbook). ???? ?? ? ??
  ?? ??? ?? ?? (stand-alone Light scattering)
• 2nd Virial Coefficient? Solute-Solvent
  interaction? ??.
• Polymer-solvent interaction, good solvent (the
  higher, the better solvent).
• 0 Unperturbed system
• - Polymer-polymer interaction, poor solvent.
• A2? ???? ?? A2 b M-a ? log A2 vs. log M?
  ??. ?? ???? ??, ? ???? ???.
• dn/dc? A2? ???? ?? ???? S. Lee, O.-S. Kwon,
  "Determination of Molecular Weight and Size of
  Ultrahigh Molecular Weight PMMA Using Thermal
  Field-Flow Fractionation/Light Scattering" In
  Chromatographic Characterization of Polymers.
  Hyphenated and Multidimensional Techniques,
  Provder, T., Barth, H. G., and Urban, M. W. Ed.
  Advances in Chemistry Ser. No. 247 ACS
  Washington, D. C., 1995 pp93.

31

• Light scattering ??? ? ? ?? ?? ? ?? (concerns)
• ??? dn/dc, RI constant, A2? ??.
• As dn/dc increases, calculated MW decrease,
  calculated mass decrease, and no effect on
  calculated RG.
• As RI constant increases, calculated MW
  decreases, calculated mass increases, and no
  effect on RG .
• As A2 increases, calculated MW increases, no
  effect on calculated mass, RG slightly increases.
• Refractive Index Detector Calibration ? ????? ?
  ??
• RI Calibration constant inversely proportional
  to the detector sensitivity.
• Sensitivity of most RI detector is
  solvent-dependent.
• A calibration constant measured in a solvent may
  not be accurate for other solvents. It is
  recommended to use a solvent that will be used
  most often (e.g., THF or toluene).
• For RI calibration, only the RI signal is used.
  Light scattering instrument calibration is not
  needed.
• Concentration of standards should be such that
  the output of RI detector varies between about
  0.1 - 1.0 V and should correspond to normal peak
  heights of samples (For a Waters 410 RI at
  sensitivity setting of 64, this corresponds
  roughly to concentrations of 0.1 - 1.0 mg/mL. RI
  output can be usually monitored by light
  scattering instrument (e.g., channel 26 of DAWN).
• Use NaCl in water as a standard for aqueous
  system.
• The RI calibration constant will change if you
  change the sensitivity setting of the detector
  So it is important to use the same sensitivity
  setting of RI detector as that used when the
  detector was calibrated.

32

• RI calibration preparation One Manual injector
  with at least 2 mL loop, Five or more known
  concentrations (0.1 - 1 mg/mL) of about 200 K
  polystyrene in THF.
• RI calibration Procedure
• Remove columns. Place manual injector with loop.
• Pump THF through a RI detector at normal flow
  rate (about 1 mL/min). Purge both reference and
  sample cells of detector until baseline becomes
  flat stable.
• Stop purging and wait till baseline becomes
  stable.
• Set up the light scattering data collection
  software (enter filename, dn/dc, etc.) Enter 1 x
  10-4 for RI constant (light scattering instrument
  usually requires the RI constants to be entered).
  Set about 60 mL for Duration of Collect .
• Begin collecting data with ASTRA.
• Inject pure solvent first followed by stds from
  low to high conc, and finish with pure solvent.
  
• Repeat the measurements if you want.
• Data Analysis (1)set baseline using signals from
  pure solvent at the beginning and the end
  (2)calculate each concentration as a separate
  peak by marking exactly 1 mL as peak width (or 30
  slices at 1 mL/min, 2 seconds of collection
  interval).(3)calculate the mass of the peak
  (4)plot the injected mass (y-axis) vs. calculated
  mass (x-axis) (5)do linear regression on data by
  forcing the intercept be zero (6)calculate RI
  constant using RI constant slope x 1x10-4

33

• Chemical heterogeneity within each slice leads to
  non-defined dn/dc ? Quantitation of chemical
  heterogeneous samples is very difficult.
• Limited sensitivity to low MW components.
  Mn(exp)gtMn(true). The same concern with
  differential viscometer experiments.
• Limited Sensitivity of Light Scattering and RI
  Detector

• g' values may be in error if each peak slice
  contains both linear and branched polymer or
  different types of long-chain branching g' will
  be overestimated.
• Quality of data is highly affected by the
  presence of particles.
• Lower limit of RG with MALS? ? 10 nm (about 100K
  MW)
• Inter-detector volume must be known accurately.

34
Comparison of online LS vs. viscometer
LS Viscometer
MWD Absolute Relative
need precise n and dn/dc Universal calibration must be valid or need M-H coefficient
independent of separation mechanism Independent of separation mechanism if M-H coefficients are used. Dependent on separation mechanism if universal calibration is used.
? distribution indirect from universal calibration direct, independent of separation mechanism
RG direct from MALS (limited to gt10 nm) indirect from universal cal. and Flory-Fox eqn. applicable to linear molecules only
Chain conformation MALLS RG vs. M plot ? vs. M plot (M-H coefficients can be obtained) RG vs. M plot.
Branching g obtained directly from MALS, indirectly from LALLS universal calibration g' obtained directly
heterogeneous samples limited because of dn/dc uncertainty directly applicable with univ. calib., but the change in dn/dc will affect DRI responses
Lower MW detectability 2K. depends on dn/dc and polydispersity as low as 300-400 has been reported
Response to particle contamination LALLS highly sensitive, MALLS less sensitive Insensitive
35
Information Content
Primary Secondary
LALLS M
MALLS M RG
PCS D Rh, M
Viscometer ? M, RG
Primary information high precision and accuracy,
insensitive to SEC variables, requires no SEC
column calibration.
36
ltSEC-VISC-LS instrumentgt

• Features
• MWD measured by LS
• IVD measured by Viscometer

37

• Both Viscometer and LS are insensitive to
  experimental conditions and separation mechanism
• No band broadening corrections are needed for Mw,
  ? , a, k, and g
• Precise and accurate calculation of hydrodynamic
  radius distribution, M-H constants, and Branching
  distribution

38

• Dynamic light scattering (DLS, QELS, PCS)
• Classical light scattering "time-averaged
  scattering intensity"? ?? ???? ??? ? scattering
  center??? ?? ?? ?? ??? ? (algebraic summation).
  
• ??? algebraic summation? ??? ? ???? random??
  array????, ?? phase relationship? scattering
  volume dimension? ??? ?? ?? ??? ?????? ??
  interference effect ?? average-out?? ??? ????
  ???.
• Scattering volume dimension? ?? ???, ???? ??? ?
  scattering center? ?? ?? ?? ?? ?? ??? interfere
  (constructive or destructive) ???? ?? ???? ???
  ???? ???? ??? ?? ????.
• ? ???? Brownian motion (diffusion) ? ?? ?? ?????
  ???? ???? ?? ?? ?? ????. ??? ???? ???? ??? ???
  ?? fluctuate??.
• Fluctuate?? ??? ???? diffusion rate? ??
  (diffusion rate? ???? ??? fluctuate).
• nanometer ?? micron??? ??? ??? ???? ?? viscosity?
  ??? viscosity? ??? media? disperse?? ?? ?, ????
  ??? ?? ?? (fluctuation)? microsecond ??
  millisecond??.

39

• A vertically polarized laser beam is scattered
  from a colloidal dispersion. The
  photomultiplier detects single photons scattered
  in the horizontal plane at an angle ? from the
  incident beam, and the technique is referred to
  as "photon correlation spectroscopy (PCS)
• Because the particles are undergoing Brownian
  motion, there is a time fluctuation of the
  scattered light intensity, as seen by the
  detector. The particles are continually
  diffusing about their equilibrium positions.
  Analyzing the intensity fluctuations with a
  correlator yields the effect diffusivity of the
  particles.
• Measured intensity, I vector sum of scattering
  from each particle
• Brownian motion motion caused by thermal
  agitation, that is, the random collision of
  particles in solution with solvent molecules.
  These collisions result in random movement that
  causes suspended particles to diffuse through the
  solution. For a solution of given viscosity, ?,
  at a constant temperature, T, the rate of
  diffusion (diffusion coefficient) D is given by
  the Stokes-Einstein equation, D(kT)/(6p?d),
  where k Boltzman's constant, d equivalent
  spherical hydrodynamic diameter. ??? diffusion
  coefficient (D)? ?????? ?? ?? (?? ???)? ??? ? ??.
• DLS??? ? ??? ??? ?? ?? ???? ??? ?? ??(t time
  interval)?? ???? ??? ????. ???? ??? ???? ???
  ??? t? ?? ?, I(0)? I(t)? ??. ?? ?? ?? interval?
  ?? ???? I(0)? I(t)? ??? ? intensity product,
  I(0)I(t)? ???? ltI2(0)gt, ? average of the square
  of the instantaneous intensity ? ???? - ?? "I(0)?
  I(t)? correlate????"?? ??. ???? ??? ???? ???
  ??? t? ? ?, I(0)? I(t)? ??? ??? ?? ??? - "I(0)?
  I(t)? correlate???? ??" ?? "I(0)? I(t)?
  un-correlate ????" ?? ??. ???? intensity
  product, I(0)I(t)? ???? ??? ltI2gt, ? square of the
  long-time averaged intensity? ??. ???? ??? ????
  ??? ??? t? ??? ??? ?? ?, "I(0)? I(t)? ?????
  correlate????".

40

• Measured intensity, I vector sum of scattering
  from each particle
• Measure I at various time interval, ?,
• I(0) I(t) for short t ? correlated,
  correlation decreases as ? increases.
• I(0)? I(t)? ?????? Correlation? ??? ??? ? ??.
  correlation? ??? ???? ?? average of the intensity
  product, G(t)? ????.
• ?? G(t)Anto correlation function
  ltI(t)I(tt)gt average of the intensity product.

• ?? ???? t ? ???? ?? G(t)? ??.
• G(t) is high for high correlation, and is low for
  low correlation.
• High correlation means that particles have not
  diffused very far during t. Thus G(t)
  remaining high for a long time interval indicates
  large, slowly moving particles.
• The time scale of fluctuation is called "decay
  time
• Decay time is directly related with the particle
  size. The inverse of decay time is the decay
  constant, ?.
• Usefulness of G(t) directly relatable to the
  particle diffusivity
• For monodisperse samples,

41
?? ?? ??? ?? ??? interval?? autocorrelation
function, G(t)? ???
G(t) vs. t? ???? ??? Exponential function? ????
G(t)? fit??.
Rh? ??, ??? ?? ???? Measure I(t) at various ? ?
G(t) ?
?? DLS ? ??? ???? diffusion? ?? ??? ?? ?? ??
dispersion ( ? 0.03)? ??? ???. ? volume
fraction of suspended spheres.
, where N Avogadro's no., M MW, Vh
hydrodynamic vol.). Infinite dilution D?? ??
???? ?? ? 0.005? ?? ??? ??.
42
???? f(a) distribution function, I(a,?)
scattering intensity function for RGD spheres.
PC? ??, normal?? log-normal distribution
function? G(t)? fit??.
?? Narrow, mono-modal distribution ??? ??,
"method of cumulant"? ??, ??? ?? ??? ? ??.

• ???? an nth moment of f(a).
• We see that DLS yields a somewhat unusual
  average radius (the inverse "z-average", and one
  which is quite highly sensitive to the presence
  of outsized particles.
• DLS uses a single exponential decay function, and
  thus it does not give information on sample
  polydispersity.

43

• ??
• RI values of medium and sample are needed for DLS
  experiments.
• RI 1.333 for water, and 1.5 - 1.55 for typical
  polymers and proteins.
• RI of sample is needed only when the intensity
  weight needs to be converted to the volume weight
  (e.g., for samples having broad distributions).
  
• Theory to convert the intensity to the volume
  is only for solid particles. So the conversion
  will not be accurate for samples such as
  liposomes which are hollow inside.
• For samples such as liposome, a value between 1.5
  - 1.55 can be used as it is typical values for
  polymers and proteins.
• For samples having narrow distributions, only the
  unimodal analysis is performed, and thus there is
  no need to convert the intensity to the volume
  .
• RI value will not make any difference in the
  average size data because only the RI of medium
  is need for unimodal analysis.

DLS summary

• D depends on MW and conformation
• Diffusion coefficient distribution can be
  obtained
• D is independent on chemical composition. ?D can
  be obtained without knowing chemical composition.
• Concentration is not needed to determine D
• Input parameters (T, n, ? ) are easily measured.
• Concerns sensitivity, interference from
  particulates, inconsistency, not very useful
  for polydispersed or multi-modal distributions.

44
lt??gt Particle Size Conversion Table
Mesh size Approximate ?µ size
4 4760
6 3360
8 2380
12 1680
16 1190
20 840
30 590
40 420
50 297
60 250
70 210
80 177
100 149
140 105
200 74
230 62
270 53
325 44
400 37
625 20
1250 10
2500 5