6.2 Simplifying Radical Expressions - PowerPoint PPT Presentation
1 / 21
Title:
6.2 Simplifying Radical Expressions
Description:
6.2 Simplifying Radical Expressions Product rule for radicals is useful in simplifying radicals. The product rule can be written as: For example 36 = 9 4 – PowerPoint PPT presentation
Number of Views:238
Avg rating:3.0/5.0
Title: 6.2 Simplifying Radical Expressions
1
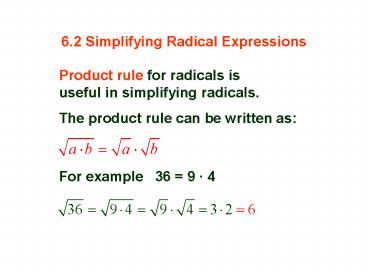
6.2 Simplifying Radical Expressions
Product rule for radicals is useful in
simplifying radicals.
The product rule can be written as
For example 36 9 4
2
Any number that can be written in the form a2 is
a perfect square.
4 22 is a perfect square.
9 32 is a perfect square.
121 112 is a perfect square.
196 142 is a perfect square.
Write some perfect square numbers on your own.
3
Any number that can be written in the form a3 is
a perfect cube.
8 23 is a perfect cube.
27 33 is a perfect cube.
64 43 is a perfect cube.
125 53 is a perfect cube.
4
Any number of the form a4 is a perfect 4th power.
16 24 is a perfect 4th power.
81 34 is a perfect 4th power.
5
It is easy to obtain the square root of a perfect
square.
6
To find the square root of a number with an
exponent, divide the exponent by 2
7
Similarly it is easy to obtain the cube root of a
perfect cube.
8
To find the cube root of a number with an
exponent, divide the exponent by 3.
9
We will use the following steps to simplify a
radical expression.
- Write each factor inside the radical as the
product of the largest perfect power for the
radical.
27 is a perfect cube.
16 and x4 are perfect squares
10
2. Use the product rule to write the expression
as a product of radicals.
- Simplify the radical containing perfect powers.
11
We write 24 as a product containing the largest
perfect square.
4 is the largest perfect square in 24
24 4 6
Write this as the product of two radicals
Simplify the perfect square radical
12
We write 18 as a product containing the largest
perfect square.
9 is the largest perfect square in 18
18 9 2
Write this as the product of two radicals
Simplify the perfect square radical
13
We write 24 as a product containing the largest
perfect cube.
8 is the largest perfect cube in 24
24 8 3
Write this as the product of two radicals
Simplify the perfect cube radical
14
We write 81 as a product containing the largest
perfect cube.
27 is the largest perfect cube in 81
81 27 3
Write this as the product of two radicals
Simplify the perfect cube radical
15
We write b23 as a product containing the largest
perfect 4th power. A perfect 4th power is
divisible by 4
b20 is the largest perfect 4th power in b23
b23 b20 b3
Write this as the product of two radicals
Simplify the perfect 4th power radical
16
Write each number inside the radical as the
product of the highest perfect square.
a7 a6 a
b11 b10 b
75 25 3
25, a6 and b10 are perfect squares.
17
Write each number inside the radical as the
product of the highest perfect cube.
16 8 3
8 is a perfect cube
x3 and y6 are perfect cubes.
18
24 4 6
Here 4 22 is a perfect square
y20 y20
z27 z26 z
x15 x14 x
x14 , y20 and z26 perfect squares as the
exponents can be divided by 2
19
This can be simplified as
5
20
This can be written as
21
This can be written under one cube root as
2