Math 2400 Test

1
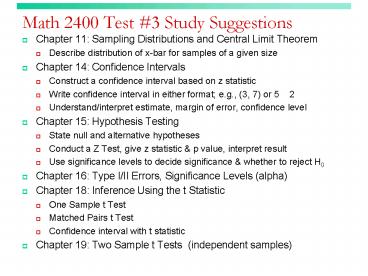
Math 2400 Test 3 Study Suggestions

• Chapter 11 Sampling Distributions and Central
  Limit Theorem
• Describe distribution of x-bar for samples of a
  given size
• Chapter 14 Confidence Intervals
• Construct a confidence interval based on z
  statistic
• Write confidence interval in either format e.g.,
  (3, 7) or 5 2
• Understand/interpret estimate, margin of error,
  confidence level
• Chapter 15 Hypothesis Testing
• State null and alternative hypotheses
• Conduct a Z Test, give z statistic p value,
  interpret result
• Use significance levels to decide significance
  whether to reject H0
• Chapter 16 Type I/II Errors, Significance Levels
  (alpha)
• Chapter 18 Inference Using the t Statistic
• One Sample t Test
• Matched Pairs t Test
• Confidence interval with t statistic
• Chapter 19 Two Sample t Tests (independent
  samples)

2
Parameters and statistics

• 1. The mean distance traveled in a year by a
  sample of truck drivers can be represented by
• .
• .
• .
• .

3
Sample size

• 2. We wish to estimate the mean price, ?, of all
  hotel rooms in Las Vegas. The Convention Bureau
  of Las Vegas did this in 1999 and used a sample
  of n 112 rooms. In order to get a better
  estimate of ? than the 1999 survey, we should
• Take a larger sample because the sample mean will
  be closer to ?.
• Take a smaller sample since we will be less
  likely to get outliers.
• Take a different sample of the same size since it
  does not matter what n is.

4
Sampling distributions

• 3. What effect does increasing the sample size,
  n, have on the spread of the sampling
  distribution of ?
• The spread of the sampling distribution gets
  closer to the spread of the population.
• The spread of the sampling distribution gets
  larger.
• The spread of the sampling distribution gets
  smaller.
• It has no effect. The spread of the sampling
  distribution always equals the spread of the
  population.

5
Sampling distributions

• 4. What effect does increasing the sample size,
  n, have on the center of the sampling
  distribution of ?
• The mean of the sampling distribution gets closer
  to the mean of the population.
• The mean of the sampling distribution gets closer
  to 0.
• The variability of the population mean is
  decreased.
• It has no effect. The mean of the sampling
  distribution always equals the mean of the
  population.

6
Sampling distributions

• 5. What effect does increasing the sample size,
  n, have on the shape of the sampling distribution
  of ?
• The shape of the sampling distribution gets
  closer to the shape of the population.
• The shape of the sampling distribution gets more
  bell-shaped.
• It has no effect. The shape of the sampling
  distribution always equals the shape of the
  population.

7
Statistical Inference

• 6. What is statistical inference on ??
• Drawing conclusions about a population mean based
  on information contained in a sample.
• Drawing conclusions about a sample mean based on
  information contained in a population.
• Drawing conclusions about a sample mean based on
  the measurements in that sample.
• Selecting a set of data from a large population.

8
Sampling distributions

• 7. Time spent working out at a local gym is
  normally distributed with mean ? 43 minutes and
  standard deviation ? 6 minutes. The gym took a
  sample of size n 24 from its patrons. What is
  the distribution of ?
• Normal with mean ? 43 minutes and standard
  deviation ? 6 minutes.
• Normal with mean ? 43 minutes and standard
  deviation ? minutes.
• Cannot be determined because the sample size is
  too small.

9
Stating hypotheses

• 8. If we test H0 ?? 40 vs. Ha ? lt 40, this
  test is
• One-sided (left tail).
• One-sided (right tail).
• Two-sided.

10
Stating hypotheses

• 9. If we test H0 ?? 40 vs. Ha ? ? 40, this
  test is
• One-sided (left tail).
• One-sided (right tail).
• Two-sided.

11
Inference

• 10. Why do we need a normal population or large
  sample size to do inference on ??
• So that the sampling distribution of is
  normal or approximately normal.
• So that the distribution of the sample data is
  normal or approximately normal.
• So that equals ?.
• So that ? is known.

12
Conclusions

• 11. Suppose the P-value for a hypothesis test is
  0.304. Using ? 0.05, what is the appropriate
  conclusion?
• Reject the null hypothesis.
• Reject the alternative hypothesis.
• Do not reject the null hypothesis.
• Do not reject the alternative hypothesis.

13
Conclusions

• 12. Suppose the P-value for a hypothesis test is
  0.0304. Using ? 0.05, what is the appropriate
  conclusion?
• Reject the null hypothesis.
• Reject the alternative hypothesis.
• Do not reject the null hypothesis.
• Do not reject the alternative hypothesis.

14
Conclusions

• 13. Suppose a significance test is being
  conducted using a significance level of 0.10. If
  a student calculates a P-value of 1.9, the
  student
• Should reject the null hypothesis.
• Should fail to reject the null hypothesis.
• Made a mistake in calculating the P-value.

15
Stating hypotheses

• 14. A consumer advocate is interested in
  evaluating the claim that a new granola cereal
  contains 4 ounces of cashews in every bag. The
  advocate recognizes that the amount of cashews
  will vary slightly from bag to bag, but she
  suspects that the mean amount of cashews per bag
  is less than 4 ounces. To check the claim, the
  advocate purchases a random sample of 40 bags of
  cereal and calculates a sample mean.
• What alternative hypothesis does she want to
  test?

16
Statistical significance

• 15. A consumer advocate is interested in
  evaluating the claim that a new granola cereal
  contains 4 ounces of cashews in every bag. The
  advocate recognizes that the amount of cashews
  will vary slightly from bag to bag, but she
  suspects that the mean amount of cashews per bag
  is less than 4 ounces. To check the claim, the
  advocate purchases a random sample of 40 bags of
  cereal and calculates a sample mean of 3.68
  ounces of cashews.
• The consumer advocate should declare statistical
  significance only if there is a small probability
  of
• Observing a sample mean of 3.68 oz. or less when
  ? 4 oz.
• Observing a sample mean of exactly 3.68 oz. when
  ? 4 oz.
• Observing a sample mean of 3.68 oz. or greater
  when ? 4 oz.
• Observing a sample mean of less than 4 oz. when ?
  4 oz.

17
Statistical significance

• 16. A consumer advocate is interested in
  evaluating the claim that a new granola cereal
  contains 4 ounces of cashews in every bag. The
  advocate recognizes that the amount of cashews
  will vary slightly from bag to bag, but she
  suspects that the mean amount of cashews per bag
  is less than 4 ounces. To check the claim, the
  advocate purchases a random sample of 40 bags of
  cereal and calculates a sample mean of 3.68
  ounces of cashews.
• Suppose the consumer advocate computes the
  probability described in the previous question to
  be 0.0048. Her result is
• Not statistically significant
• Statistically significant at a .10 but not at a
  .05
• Statistically significant at a .05 but not at a
  .01
• Statistically significant at a .01 but not at a
  .001
• Statistically significant at a .001

18
Statistical significance

• 17. A consumer advocate is interested in
  evaluating the claim that a new granola cereal
  contains 4 ounces of cashews in every bag. The
  advocate recognizes that the amount of cashews
  will vary slightly from bag to bag, but she
  suspects that the mean amount of cashews per bag
  is less than 4 ounces. To check the claim, the
  advocate purchases a random sample of 40 bags of
  cereal and calculates a sample mean of 3.68
  ounces of cashews.
• If the probability described in the previous
  question is 0.0048, then the consumer advocate
  should conclude that the granola is
• Correctly labeled.
• Incorrectly labeled.

19
Confidence Intervals

• 18. The purpose of a confidence interval for ? is
  
• To give a range of reasonable values for the
  level of confidence.
• To give a range of reasonable values for the
  sample mean.
• To give a range of reasonable values for the
  population mean.
• To give a range of reasonable values for the
  difference between the sample mean and the
  population mean.

20
Confidence intervals

• 19. A very large school district in Connecticut
  wants to estimate the average SAT score of this
  years graduating class. The district takes a
  simple random sample of 100 seniors and
  calculates the 95 confidence interval for the
  graduating students average SAT score at 505 to
  520 points.
• For the sample of 100 graduating seniors, 95 of
  their SAT scores were between 505 and 520 points.
• Correct interpretation of interval.
• Incorrect interpretation of interval.

21
Confidence intervals

• 20. A very large school district in Connecticut
  wants to estimate the average SAT score of this
  years graduating class. The district takes a
  simple random sample of 100 seniors and
  calculates the 95 confidence interval for the
  graduating students average SAT score at 505 to
  520 points.
• The school district can be 95 confident that the
  mean SAT score of the 100 students is contained
  in the interval of 505 to 520 points.
• Correct interpretation of interval.
• Incorrect interpretation of interval.

22
Confidence intervals

• 21. A very large school district in Connecticut
  wants to estimate the average SAT score of this
  years graduating class. The district takes a
  simple random sample of 100 seniors and
  calculates the 95 confidence interval for the
  graduating students average SAT score at 505 to
  520 points.
• The school district can be 95 confident the
  interval of 505 to 520 captures the true average
  SAT score of their graduating students.
• Correct interpretation of interval.
• Incorrect interpretation of interval.

23
Margin of error

• 22. Increasing the confidence level will
• Increase the margin of error.
• Decrease the margin of error.

24
Margin of error

• 23. Increasing the sample size will
• Increase the margin of error.
• Decrease the margin of error.

25
t-distribution

• 24. When do we use the t-distribution to make
  inference about ??
• When we know ? but ? is unknown.
• When we have a very large sample size.
• When the data are very skewed or when outliers
  are present.
• When we do not know ? or ?.

26
Type of scenario

• 25. A university professor wanted to know if the
  attitudes towards statistics changed during the
  course of the semester. She took a simple random
  sample of students and gave them a test at the
  beginning of the term to assess their feelings
  toward statistics. When the semester was
  finished she administered another test to the
  same group of students and wanted to see if there
  was a difference between the average attitude
  towards statistics.
• What type of scenario is this?
• Matched pairs (dependent samples)
• Two independent samples

27
Type of scenario

• 26. The National Park Service is interested in
  comparing the amount of money that visitors in
  two different national parks spend. They sample
  visitors on the same day in each of the two parks
  and then compare the mean dollar amounts spent
  from each sample.
• What type of scenario is this?
• Matched pairs (dependent samples)
• Two independent samples

28
Type of scenario

• 27. Researchers at a pharmaceutical company were
  developing a new formula for their sunscreen.
  They wanted to see if the new formula provided
  better protection against sunburns than the
  formula that was already on the market. They
  applied the new formula to one arm of an
  individual and the old formula to the other arm,
  randomly choosing the arms for each formula.
  Then they compared the color difference between
  the arms.
• What type of scenario is this?
• Matched pairs (dependent samples)
• Two independent samples

29
Hypotheses

• 28. An experiment was conducted to see if elderly
  patients had more trouble keeping their balance
  when loud, unpredictable noises were made
  compared to younger patients who were also
  exposed to the noises. Researchers compared the
  amount of forward and backward sway for the two
  groups.
• If we wanted to test whether the younger patients
  had less average forward/backward sway, we would
  use which of the following hypotheses?

30
t procedures

• 29. A train operator claims that her trains on
  average are not more than 7 minutes late. A
  commuter takes a simple random sample of trains
  arriving at her local station of size n 21 and
  records their estimated arrival time and the
  actual arrival time. She creates the following
  stemplot for these data
• Based on the stemplot, should she continue with
  her use of the t-procedure?
• No, because the sample size is not large enough.
• Yes, because the data show no strong skewness or
  outliers.

31
t procedures

• 30. The following histogram represents the yearly
  advertising budgets (in millions of dollars) of
  21 randomly selected companies. A statistics
  student wants to create a confidence interval for
  the mean advertising budget of all companies. By
  looking at the histogram, is the use of a t
  procedure appropriate in this case?
• Yes, because data were from an experiment.
• Yes, because the sample size is large enough.
• No, because the data are skewed and have
  outliers.
• No, because we did not repeat the sampling enough
  times.

32
Hypothesis testing

• 31. A tire manufacturer claims that one
  particular type of tire will last at least 50,000
  miles. A group of angry customers does not
  believe this is so. They take a sample of 14
  tires and want to test if the mean mileage of the
  tires is really less than 50,000. What set of
  hypotheses are they interested in testing?

33
Hypothesis testing

• 32. A tire manufacturer claims that one
  particular type of tire will last at least 50,000
  miles. A group of angry customers does not
  believe this is so. They take a sample of 14
  tires and want to test if the mean mileage of the
  tires is really less than 50,000.
• If P 0.028, what decision should be made if
  testing at the ? 0.05 level?
• Reject H0 and conclude that the tires were not
  performing as claimed.
• Reject H0 and conclude that the mean tire life
  really is 50,000 miles.
• Do not reject H0 and conclude that the tires were
  not performing as claimed.
• Do not reject H0 and conclude that the mean tire
  life really is 50,000 miles.

34
Distributions

• 33. When we replace ? with s in our standardized
  test statistic formula, the distribution of the
  test statistic
• Changes to a t-distribution.
• Stays as the standardized Normal.
• Changes to an estimate of the Normal distribution.

35
Parameters and statistics

• 34. The average fuel tank capacity of all cars
  made by Ford is 14.7 gallons. This value
  represents a
• Parameter because it is an average from all
  possible cars.
• Parameter because it is an average from all Ford
  cars.
• Statistic because it is an average from a sample
  of all cars.
• Statistic because it is an average from a sample
  of American cars.

36
Solutions

• B
• A
• C
• A
• B
• A
• B
• A
• C
• A

• C
• A
• C
• C
• A
• D
• B
• C
• B
• B

37
Solutions Part 2

• B
• B
• A
• A
• A
• B
• A
• D
• B
• C

• A
• A
• A
• B