MAXWELLS EQUATIONS - PowerPoint PPT Presentation
1 / 15
Title:
MAXWELLS EQUATIONS
Description:
Proof of Conservation of EM energy from the Maxwell's Equations. EM ... Dissipation in conductors. Generators produce ( ) or dissipate (-) energy/unit time ... – PowerPoint PPT presentation
Number of Views:97
Avg rating:3.0/5.0
Title: MAXWELLS EQUATIONS
1
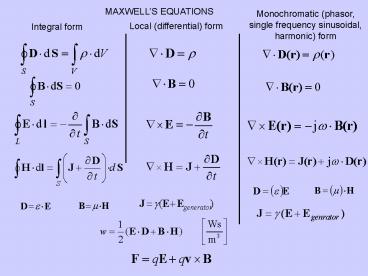
MAXWELLS EQUATIONS
Monochromatic (phasor, single frequency
sinusoidal, harmonic) form
Local (differential) form
Integral form
2
Electromagnetic Power and Poynting Theorem
ENERGY DENSITY
LORENTZ FORCE (Mechanical force)
CONSERVATION OF ENERGY IN EM FIELDS
Total power generated by the sources
(batteries, generators, etc.)
Rate if increase of electric and magnetic stored
energy
Power density emanating RADIATION
Power dissipated
3
Proof of Conservation of EM energy from the
Maxwells Equations
Quod erat demonstrandum
4
EM energy stored in Volume V
Dissipation in conductors
Generators produce () or dissipate (-)
energy/unit time
Power leaves the volume through RADIATION
Poynting vector
5
Conservation of EM energy Illustration of the
terms
The energy flows in dielectrics, not in wires
Illustration
The energy flows from the generator to the load
in the dielectric (not in the wires)
6
If the wires are lossy, the energy which is
dissipated by the wires flows from the field into
the wires through the surface of the wires
The energy per unit time flowing into the wire of
length l through its surface
This is the Joule power dissipated by the wire
7
IF IS NOT ZERO ? ENERGY
FLOWS
If we discharge the capacitor, the electric
field disappears and the circulation of energy
stops
The discharge and the magnetic field interacts,
and the momentum of the interaction is equal to
the momentum of the circulating EM energy
Mass
Momentum
8
UNIQUENESS OF THE SOLUTION OF MAXWELLS EQUATIONS
We assume, that there are TWO SOLUTIONS, both
satisfying the SAME INITIAL AND BOUNDARY
CONDITIONS. We show that THEY ARE IDENTICAL.
Proof
Two solutions
Both satisfies Maxwells Equations, initial and
boundary conditions. From linearity it follows
that their difference also satisfy them.
We can wrtite for the diffenrence
Generators are the same, and at the boundary
fields are the same, thus
Note that the rhs is always positive, thus the
total energy can only decrease in time
From the initial conditions it follows that at t
t0 the total energy is ZERO. The total
energy can never be negative, thus
Quod erat demonstrandum
9
IF inside a closed volume THE INITIAL
CONDITIONS are given , i.e. the ELECTRIC AND
MAGNETIC FIELDS ARE KNOWN at a given moment
of time t t0,
AND the GENERATORS either the TANGENTIAL
ELECTRIC or the TANGENTIAL MAGNETIC fields are
given at the SURFACE enclosing the volume from
t0 to t (BOUNDARY CONDITIONS),
THEN the ELECTROMAGNETIC FIELD INSIDE THE VOLUME
can be determined from the Maxwell equations
UNIQUELY.
Power generated inside the volume
Increases the total EM energy
Dissipated power
Radiated power
10
MAXWELLS EQUATIONS ? KIRCHOFFS EQUATIONS
KIRCHHOFF I. CONSERVATION OF CHARGE
KIRCHHOFF II. CONSERVATION OF ENERGY
IN DIRECT CURRENT CIRCUITS (DC)
11
GENERATORS AND IDEAL CIRCUIT ELEMENTS
Battery
GEBERATORS CHARGE SEPARATORS
12
Lineáris ido-invariáns koncentrált paraméteru
áramkörök
Ha kondenzátor is van az áramkörben, akkor
13
TRANSMISSION LINE AS LINEAR DISRIBUTED CIRCUIT
14
(No Transcript)
15
(No Transcript)