This Handout:

1
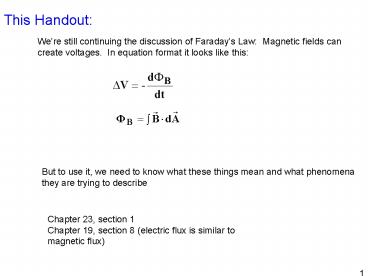
This Handout
Were still continuing the discussion of
Faradays Law Magnetic fields can create
voltages. In equation format it looks like this
But to use it, we need to know what these things
mean and what phenomena they are trying to
describe
Chapter 23, section 1 Chapter 19, section 8
(electric flux is similar to magnetic flux)
2
From Experiments we know the following are
important when B fields create voltages. A
voltage is created if one of the following is
true

• The magnetic field changes
• The area of the circuit changes
• The orientation of the circuit with respect to
  the magnetic field changes (i.e. the circuit is
  twisted or rotated)

It also depends on how quickly these changes
occur. The faster the change, the more voltage is
created.
What concepts to use? Well, B matters, A matters
and cos q matters (where the angle is measured
relative to A and B to quantify the
rotation/twist). And since these must change
with time, we know the derivative is important
too.
3
Flux is what emboddies these ideas
As well see later, this is only true if the B
field is the same value everywhere on the area.
If it isnt well have to do an integral
And Faradays law indicates that it is the time
rate of change of flux that matters
Subbing in for flux
4
But what is flux?
It also means flow but in the case of magnetic
fields, nothing is moving. The relation between
flow and flux comes from fluids moving. Consider
the following three screens in the stream of a
flowing fluid
Which screen has the largest amount of fluid
flowing through it?
5
Which screen has the largest amount of fluid
flowing through it?
A
A
A
6
So flux is defined as
That is only true if the angle and the magnetic
field are the same across the entire area. If
they are not, we must resort to the integral form
Where we think of the large area as being made up
of a bunch of little patches, dA
dA
7
Lecture Question What is the flux through this
area, A 1.2 m2, with a magnetic field of 5
Tesla and the area is tilted at an angle of q
30o ?

• 6 Tm2
• 5.2 Tm2
• 3 Tm2
• 0 Tm2

B
8
Before we leave the introduction to flux, it
might be useful to compare the two ideas that
weve had this semester which involve the dot
product Flux and Voltage
For simplicity, assume that the Electric Field
and Magnetic field are constant
path
Area
DV ?
Flux ?
E
B
DV ?
Flux ?
E
B
9
Lecture Question Which path has the largest
change in voltage? The field, the length of the
path and the angle are all the same.

• Path A
• Path B
• They both have the same change in voltage

Path B
Path A
E
E