Band diagrams for materials

1
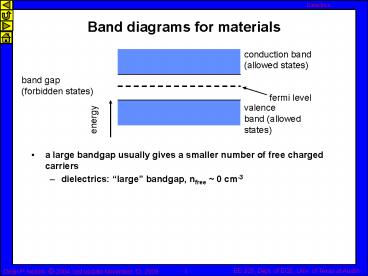
Band diagrams for materials

• a large bandgap usually gives a smaller number of
  free charged carriers
• dielectrics large bandgap, nfree 0 cm-3

2
Conductors in an applied field

• when an electric field is applied to a material
  the charges that make up the atoms in the
  material will respond

• recall E inside a conductor (in the absence of
  current flow) is zero
• the surface charge density is equal to Dnormal

• in a conductor, which contains free charges, the
  charges move to the surfaces of the conductor in
  such a way that they produce an induced field
  that exactly cancels the applied field inside the
  conductor (must be true so the current is zero)

• the separation of induced charge between the
  surfaces could be viewed as an induced dipole

3
Dielectric materials

• when an electric field is applied to a material
  the charges that make up the atoms in the
  material will respond
• in an insulator, or dielectric, which contains NO
  (or at least very few) truly free charges, the
  charges cannot move all the way to the surfaces
• but the charge displacement at the atomic level
  can still produce an induced field that PARTIALLY
  cancels the applied field inside the material
• the separation of induced charge between the
  surfaces could be viewed as an induced dipole

imagine a small piece of the material
Dinduced
Eapplied
4
Dielectric materials

• imagine a small piece of the material
• the charge displacement at the atomic level
  produces an induced field that PARTIALLY cancels
  the applied field inside the material
• the separation of induced charge is viewed as an
  induced dipole
• the induced D field is called the polarization
  P
• it makes sense that the induced charge
  separation, and hence the induced polarization
  field, would be proportional to the applied field
• P    e0 c E
• c is the dimensionless dielectric susceptibility
  of the material
• well apply Gausss law to this separation of
  BOUND charge Qb

Dinduced P
Eapplied
5
Dielectric materials

• imagine a small piece of the material
• the induced D field is called the polarization
  P
• P    e0 c E
• c is the dimensionless dielectric susceptibility
  of the material
• apply Gausss law to this BOUND charge Qb as well
  as the total and free charges

Dinduced P
Eapplied
gaussian surface
6
Dielectric materials

• the induced D field is called the polarization
  P
• P    e0 c E
• c is the dimensionless dielectric susceptibility
  of the material

Dinduced P
Eapplied
gaussian surface
7
Tangential E field at the interface between two
dielectrics

• imagine there is some E at a dielectric interface
• lets find the voltage going aroundthe path
  a-b-c-d-a
• let the sides Dh of path get very small

dielectric e1

• the component of the electric field tangent to
  the interface between two dielectrics is
  continuous

8
Normal D field at the interface between two
dielectrics

• image there is some D at the interface of two
  dielectrics
• Gausss law
• let the sides shrink to zero (i.e., let Dh?0)
• all that is left is the top and bottom!!
• D?dS picks out the normal component of D

dielectric e1
normally there is no free charge so
9
Summary page Electrostatic boundary conditions
for dielectrics

• at the interface between two dielectrics
• the component of the electric field tangent to
  the interface between two dielectrics is
  continuous
• Dtan is discontinuous
• the component of the D field normal to the
  interface between two dielectrics is may change
• if there is no free charge at the interface
• if there is a surface charge
• Enorm is discontinuous
• material properties

10
Summary page Electrostatic boundary conditions
as you cross the surface between two media

• at the interface between two materials
• the component of the electric field tangent to
  the interface between two materials (conductor or
  dielectric!) is continuous
• for a conductor with no currents Einside Etan2
  0 ? Etan conductor 0
• for a dielectric Dtan is discontinuous ? Dtan
  1/e1 Dtan 2/e2
• the component of the D field normal to the
  interface (i.e., perpendicular to the interface)
  between two materials is changes by the free
  surface charge density
• for a conductor with no currents Dinside D- 2
  0 ? D- cond rS
• for a dielectric with no surface charge D- 1 D-
  2

11
The continuity equation

• consider the difference between flux of charged
  particles entering a small volume and flux
  leaving that volume
• must be related to change in concentration within
  that volume
• (flux out) (flux in) rate that charged
  carriers accumulate in the small volume
• we already know that the divergence of J gives
  the net flux emerging from a point
• so adding conservation of charge gives

12
What happens to changes in free carriers
concentration?

• this is no longer a STATICS problem
• but well try it anyway
• we have Ohms law and the continuity equation
• we also have the relation between D and E
• lets assume the material is homogeneous

13
Dielectric relaxation time

• we have so far
• now recall Gausss law in differential form
• if we assume that s and e are constant then we
  could solve this differential equation
• there is a characteristic decay time for charge
  perturbations
• the dielectric relaxation time t is given by
• order of magnitude e 10-11 F/m s 107 /Om

14
demonstration applet

• lots of configurations http//www.falstad.com/ems
  tatic/directions.html
• local version
• What now
• Lets combine dielectrics and conductors

15
Piezoelectric effect

• depends on polarization, P, in a material
• symmetry of crystal is critical
• for crystal with center of symmetry, application
  of external stress does NOT produce a net
  polarization

16
Piezoelectric effect

• depends on polarization, P, in a material
• symmetry of crystal is critical
• for crystal without simple center of symmetry
  application of external stress DOES produce a net
  polarization

17
Piezoelectric effect

• reciprocity between applied voltage resulting in
  displacement and applied force/displacement
  producing voltage
• constants relating voltage to displacement have
  units of charge/force

for force applied along any axis, voltage across
thickness is
18
Piezoelectric materials

• unit check dV (coul/newt)volt
  (C/N)(Ncm/C) cm

19
Piezoresistive effects

• again fundamental origin is distortion of crystal
  structure
• for conducting material can be viewed as
  distortion of bands
• leads to change in effective mass
• leads to change in resistivity / conductivity
• is dependent on direction of stress s relative to
  resistor axis and crystal directions

• for silicon
• depends on doping type and concentration, as well
  as orientation of wafer and layout of resistor
• drops rapidly for doping gt 1018 cm-3

20
Piezoresitive coefficients for (100) Si, n, p lt
1018

• also depends on temperature
• 0.25 per C