The conditioned stimulus

1
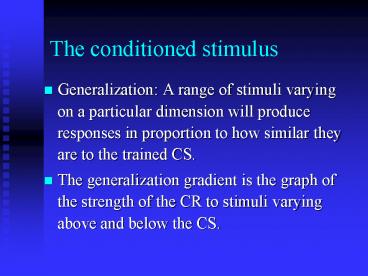
The conditioned stimulus

• Generalization A range of stimuli varying on a
  particular dimension will produce responses in
  proportion to how similar they are to the trained
  CS.
• The generalization gradient is the graph of the
  strength of the CR to stimuli varying above and
  below the CS.

2
A generalization gradient
3
Discrimination learning

• If stimuli other than the CS are presented, but
  never paired with the US, the generalized
  responses to similar stimuli will diminish, so
  that the individual discriminates between CS and
  non-CS.
• Examples of classically-conditioned
  discrimination learning abound in education
  colors, shapes, letters...The color or shape or
  letter is CS, the teachers word for it is the US.

4
Results of discrimination learning
Generalization around a light CS
40
30
20
Eyeblinks per minute
10
0
470
480
490
500
510
520
530
Wavelength of light, nm (Color)
5
The nature of the CR

• The CR is always different from the UR.
• Sometimes the CR differs from the UR only in
  magnitude, duration, or latency.
• But sometimes, the CR is opposite to the UR
  Relaxation and slowed heart rate as a CR to a CS
  for shock, but agitation and increased heart rate
  as a UR to the actual shock US.

6
Opponent Process Theory

• Opponent processes are bodily reactions opposite
  to the effect of a US, in order to preserve
  physiological balance.
• If a CS is conditioned to a US, the opponent
  process rather than the UR becomes the CR.
• Wagner (1981) argued that opponent processes are
  conditioned as CRs only for URs which provoke
  compensatory reactions, but not for monophasic
  URs SOP.

7
Applications of SOP

• Drug tolerance
• CS (setting for drug taking) US (drug)
  ----gt CR (tolerance)
• US (drug) without CS (setting) ----gt Overdose
• Spousal boredom
• CS (setting Usual routine) US (spouse)
  ----gt CR (boredom)
• US (spouse) without CS (new setting) ----gt
  Excitement

8
Contiguity vs. contingency

• The S-S and S-R theories we have studied operate
  on the associationist principle of contiguity
  Research on CS-US intervals.
• A competing view is called contingency
  differential prediction of the US by the CS, not
  mere co-occurrence.
• In a contingent relationship,
• p(US CS) gt p(US not-CS)

9
Rescorla and contingency learning

• The greater the differential contingency, the
  greater the learning (Correct legend p.59)
• The suppression ratio (A - P)/(A P)
• A is the response rate in the Absence of the CS
• P is the response rate in the Presence of the CS
• If A P, there is no suppression, and the ratio
  0
• If A gt P, there is suppression, expressed in the
  ratio.
• Thus, the higher the ratio, the greater the
  suppression, and the greater the learning.

10
Contiguity or contingency Rescorla (1968)

• Notice that the greater the difference between
  the pUSCS and the pUSCS, the greater the
  suppression ratio Contingency.

11
Occasion setting and conditioned inhibition

• Conditioned inhibition Summation paradigm
• Separately condition CS to US and CS- to not-US
• Test CS and CS- together
• Amount of reduction of CR shows strength of CS-
• Conditioned inhibition Retardation paradigm
• First condition CS- to not-US
• Then, keeping CS-, try to condition CS to US
• Amount of retardation of conditioning shows
  strength of CS-
• Occasion setting
• Clocks and kisses

12
Preparedness and associative bias Garcia

• Shock US conditions better to light/tone CS than
  to taste CS
• Nausea-inducing US drug conditions better to
  taste CS than to light/tone CS
• Possible conclusion Associations are reasonable
  inferences

13
Stimulus combinations

• Kamin (1968) Informativeness, blocking, and
  unblocking
• Group 1 CS1 CS2 ---gt US (eight trials)
• Group 2 CS1 ---gt US (sixteen trials)
• CS1 CS2 ---gt US (eight trials)
• Group difference?
• Configural cue learning not individual cue
  learning A B but not A or B A or B but not
  A B AC and BD but not A C or B D

14
Rescorla-Wagner theory

• The theory has an equation with three parts
• V, the amount of learning acquired
• a , the rate of learning
• l , the maximum amount of learning that can
  happen to the particular US.
• Together, DV a ( l - V)
• The equation is applied once for each learning
  trial, to see how much learning will happen on
  each trial.

15
Example of Rescorla-Wagner computations

• DV a ( l - V)
• If a .20 and l 100, work through 4 trials
• Trial 1 .20 ( 100 - 0) 20
• Trial 2 .20 ( 100 - 20) 16
• Trial 3 .20 ( 100 - 36) 12.8
• Trial 4 .20 ( 100 - 48.8) 10.24
• Note the diminishing returns with repeated
  trials. Will learning ever stop?

16
Another example

• DV a ( l - V)
• If a .10 and l 200, work through 5 trials
• Trial 1 .10 ( 200 - 0) 20
• Trial 2 .10 ( 200 - 20) 18
• Trial 3 .10 ( 200 - 38) 16.2
• Trial 4 .10 ( 200 - 54.2) 14.58
• Trial 5 .10 ( 200 - 68.78) 13.12
• In a relative sense, learning is happening more
  slowly here.

17
A learning curve from Rescorla-Wagner theory
18
Rescorla-Wagner and compound stimuli

• Competitive learning The total learning
  available, l , must be shared by each stimulus in
  a compound. Thus, the total amount of learning
  to each stimulus is less in a compound than if
  that stimulus is alone.
• Rescorla-Wagner predicts blocking and conditioned
  inhibition accurately.

19
Problems with Rescorla-Wagner

• CS preexposure produces slower conditioning to CS
  later, a phenomenon known as latent inhibition.
  For example, displaying the letters of the
  alphabet around the classroom before they are
  learned is CS preexposure.
• Latent inhibition is not predicted by
  Rescorla-Wagner, unless you assume that
  preexposure lowers the learning rate (a) by
  lowering salience.

20
Connectionism A Neural model

• Learning may involve forming patterns of synaptic
  connections.
• ABCD --gt CR1, ie US1
• ABCF --gt CR2, ie US2
• The rule for connection formation is the delta
  rule, based on Rescorla-Wagner
• CSs are inputs USs are outputs.

21
Applications of classical conditioning

• Classroom structure
• Make salient what is to be learned
• Eliminate redundant CSs
• Establish differential contingency
• Extinction
• Counterconditioning
• Desensitization
• Flooding