Line groups - PowerPoint PPT Presentation
1 / 33
Title:
Line groups
Description:
taken in turn & extended with conforming 3-D lattice translation groups. ... What about 1-D line groups? Consider a 3-D object of arbitrary pt. ... – PowerPoint PPT presentation
Number of Views:55
Avg rating:3.0/5.0
Title: Line groups
1
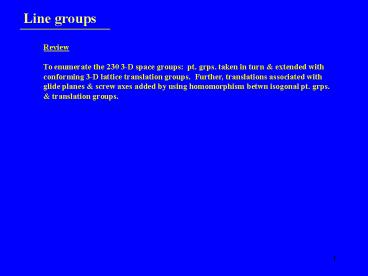
Line groups
Review To enumerate the 230 3-D space groups
pt. grps. taken in turn extended with
conforming 3-D lattice translation groups.
Further, translations associated with glide
planes screw axes added by using homomorphism
betwn isogonal pt. grps. translation groups.
2
Line groups
Review To enumerate the 230 3-D space groups
pt. grps. taken in turn extended with
conforming 3-D lattice translation groups.
Further, translations associated with glide
planes screw axes added by using homomorphism
betwn isogonal pt. grps. translation
groups. The 17 2-D plane groups can be
developed in a similar manner. Removal of a
dimension severely restricts no. of symmetry
combinations.
3
Line groups
Review To enumerate the 230 3-D space groups
pt. grps. taken in turn extended with
conforming 3-D lattice translation groups.
Further, translations associated with glide
planes screw axes added by using homomorphism
betwn isogonal pt. grps. translation
groups. The 17 2-D plane groups can be
developed in a similar manner. Removal of a
dimension severely restricts no. of symmetry
combinations. What about 1-D line
groups? Some surprises(?)
4
Line groups
What about 1-D line groups? Consider a 3-D
object of arbitrary pt. symmetry repeated along
1-D lattice
5
Line groups
What about 1-D line groups? Consider a 3-D
object of arbitrary pt. symmetry repeated along
1-D lattice Only one type of translation -
no possibility for centering translations Translat
ions simply described by single lattice parameter
6
Line groups
What about 1-D line groups? Consider a 3-D
object of arbitrary pt. symmetry repeated along
1-D lattice Only one type of translation -
no possibility for centering translations Translat
ions simply described by single lattice
parameter But lattice parameter not enough
to describe structure of array
7
Line groups
Need to also describe symmetry of
object Can use pt. grps. for this All
objects can be described by one of the 32 pt.
grps.
8
Line groups
Need to also describe symmetry of
object Can use pt. grps. for this All
objects can be described by one of the 32 pt.
grps. Doesn't quite work Consider
9
Line groups
Note glide in this planar structure
repeat unit
10
Line groups
Note glide in this planar structure c-glid
es allowed
repeat unit
11
Line groups
Note glide in this planar structure
repeat unit
1 C2 C2' C2" m m' m" i
1 1 C2 C2' C2" m m' m" i
C2 C2 1 C2" C2' i m" m' m
C2' C2' C2" 1 C2 m" i m m'
C2" C2" C2' C2 1 m' m i m"
2/m 2/m 2/m
m
C2
m'
C2"
m"
C2'
12
Line groups
Note glide in this planar structure
repeat unit
G 1t 2t 3t zt xt yt it
G G 1t 2t 3t zt xt yt it
1t 1t G 3t 2t it zt xt yt
2t 2t 3t G 1t yt it zt xt
3t 3t 2t 1t G xt zt it yt
2/m 2/m 2/m
m,zt
C2,1t
m'.xt
C2",3t
m",yt
C2',2t
Rules 1t 2t 3t 1t zt it 1t xt
yt (as before) 1t it zt 1t yt xt
13
Line groups
Note glide in this planar structure
repeat unit
G 1t 2t 3t zt xt yt it
G G 1t 2t 3t zt xt yt it
1t 1t G 3t 2t it zt xt yt
2t 2t 3t G 1t yt it zt xt
3t 3t 2t 1t G xt zt it yt
2/m 2/m 2/m
m,zt
C2,1t
m'.xt
C2",3t
m",yt
C2',2t
Rules 1t 2t 3t 1t zt it 1t xt yt
Choose 1t c/2, 3t 0 (as before) 1t
it zt 1t yt xt it 0, yt
0 Then 2t zt xt c/2
14
Line groups
Note glide in this planar structure
C2,1t
m'.xt
C2",3t
L 21/sm s2/c 2/m or L 21/mcm
m,zt
repeat unit
m",yt
C2',2t
G 1t 2t 3t zt xt yt it
G G 1t 2t 3t zt xt yt it
1t 1t G 3t 2t it zt xt yt
2t 2t 3t G 1t yt it zt xt
3t 3t 2t 1t G xt zt it yt
2/m 2/m 2/m
m,zt
C2,1t
m'.xt
C2",3t
m",yt
C2',2t
Rules 1t 2t 3t 1t zt it 1t xt yt
Choose 1t c/2, 3t 0 (as before) 1t
it zt 1t yt xt it 0, yt
0 Then 2t zt xt c/2
15
Line groups
Note glide in this planar structure
Also consider
repeat unit
16
Line groups
Note glide in this planar structure
Also consider "Non-crystallographic" rotatio
ns can be crystallographic in 1-D lattices
repeat unit
17
Line groups
Rotation axes of any order, up to C8, proper or
improper, allowed
C8
18
Line groups
Rotation axes of any order, up to C8, proper or
improper, allowed Multiple rotation
axes (n/n) allowed (n even perpendicular
mirror)
C8
19
Line groups
Rotation axes of any order, up to C8, proper or
improper, allowed Multiple rotation
axes (n/n) allowed (n even perpendicular
mirror) Screw axes of any kind (21, 53, 149)
allowed
C8
20
Line groups
Summary In line groups, pt. symmetry extended
considerably Translations along lattice
direction allowed No translations in any other
direction
21
Line groups
- Procedure
- Combine lattice translations w/ 32 pt. grps.
- As in 2-D 3-D, lattice group must be invariant
under all pt. grp. operations - Thus, while infinite no. of types of rotation
axes allowed along lattice direction, only 2 2
axes allowed otherwise must be perpendicular to
lattice direction
22
Line groups
Procedure 1. Combine lattice translations w/ 32
pt. grps. 2. Add rotation axes not allowed in
2-D 3-D (use Euler construction) Or
remember New axis appears at angle of 1/2
throw of main rotation axis
new axis
23
Line groups
Procedure 1. Combine lattice translations w/ 32
pt. grps. 2. Add rotation axes not allowed in
2-D 3-D (use Euler construction) 3. Determine
line groups isogonal w/ all allowed point
groups Infinite no. of line groups possible
24
Line groups
Procedure 1. Combine lattice translations w/ 32
pt. grps. 2. Add rotation axes not allowed in
2-D 3-D (use Euler construction) 3. Determine
line groups isogonal w/ all allowed point
groups Infinite no. of line groups
possible Examples for 1 L1, L4/m, L622,
L2/m 2/m 2/m
25
Line groups
Procedure 1. Combine lattice translations w/ 32
pt. grps. 2. Add rotation axes not allowed in
2-D 3-D (use Euler construction) 3. Determine
line groups isogonal w/ all allowed point
groups Infinite no. of line groups
possible Examples for 1 L1, L4/m, L622,
L2/m 2/m 2/m Examples for 2 L5, L14/m, L8/m
2/m 2/m
26
Line groups
Line groups (from Vujicic, Bozovic, Herbut, J.
Phys. A 10, 1271 (1977) Pt. grp. n
odd n even (w/example) Cn Ln
Lnp Cnv (4mm) Lnm Lnmm Lnc Lncc L(2q)q
mc Cnh (4/m) Ln/m L(2q)q/m S2n
(3) Ln L(2n) Dn (422) Ln2 Ln22 Lnp2 Lnp22
Dnd (42m) Lnm L(2n)2m Lnc L(2n)2c Dnh (4/m
2/m 2/m) L(2n)2m Ln/mmm L(2n)2c Ln/mcm L(2
q)q/mcm
n 1, 2, . p 1, 2, ., n-1
27
Line groups
Line groups - example - nanotubes
84
1/2
(4,0)
28
Line groups
Line groups - example - nanotubes
84
1/2
84/m
(4,0)
1/2
29
Line groups
Line groups - example - nanotubes
84
1/2
84/m_m
(4,0)
1/2
30
Line groups
Line groups - example - nanotubes
84
1/2
84/mcm
(4,0)
1/4
1/2
31
Line groups
Line groups - example - nanotubes
0
Ch na1 ma2 (n,m)
32
Line groups
Line groups - example - nanotubes
33
Line groups
Line groups - example - nanotubes
armchair zigzag chiral