Solving Inequalities

1
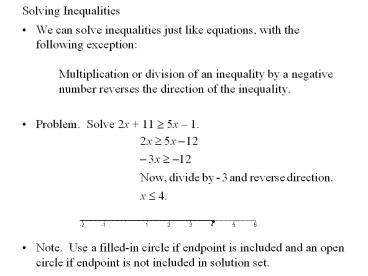
Solving Inequalities

• We can solve inequalities just like equations,
  with the following exception
  Multiplication or division of an
  inequality by a negative number reverses the
  direction of the inequality.
• Problem. Solve 2x 11 5x 1.
• Note. Use a filled-in circle if endpoint is
  included and an open circle if endpoint is not
  included in solution set.

2
Compound Inequalities

• Problem. Solve the inequality
  Solution.
• The solution is the half-open interval
  which may be represented graphically as

3
Critical Value Method

• The Critical Value Method is an alternative to
  the algebraic approach to solving inequalities.
• The critical values of an inequality are 1.
  those values for which either side of the
  inequality is not defined (such as a denominator
  equal to 0). 2. those values that are solutions
  to the equation obtained by replacing the
  inequality sign with an equal sign.
• The critical values determine endpoints of
  intervals on the number line. A given inequality
  is satisfied at all points in one of these
  intervals or it is satisfied at none of these
  points.
• In order to find out in which intervals the given
  inequality holds, we may test any point in each
  interval.

4
Example for Critical Value Method

• Solve the inequality.
• The solution is the half-open interval
  which may be represented graphically as

5
Solving a polynomial inequality using the
critical value method

• Solve (x 2)(2x 5)(3 x) lt 0.
• The critical values are
• Graphically, the solution is
• The solution consists of two open intervals

6
Misuse of inequality notation

• Students often write something similar to 1 gt x gt
  5. This is incorrect since x cannot
  simultaneously be less than 1 and greater than 5.
  What is likely intended is that either x lt 1 or
  x gt 5.
• Two additional misuses of inequality notation are
  given in the following examples. Do you see why
  they are incorrect or misleading?

7
Linear and Quadratic Inequalities We discussed

• Solving inequalities is like solving equations
  with one exception
• Interval notation and number line representation
• Critical value method
• Misuse of inequality notation

8
Absolute Value in Equations

• Solve 2x 7 11.
• When removing absolute value brackets, we must
  always consider two cases.

9
Graphical interpretation of certain inequalities

• Let a be a positive real number. The inequality
  x lt a has as solution all x whose distance from
  the origin is less than a. The solution set
  consists of the interval (a, a).
• The inequality x gt a has as solution all x
  whose distance from the origin is greater than a.
  For this inequality, the solution set consists
  of the two infinite intervals (?, a) and (a,
  ?).

a
0
a
a
a
0
10
Absolute Value in Inequalities

• To solve an inequality involving absolute values,
  first change the inequality to an equality and
  solve for the critical values. Once you have the
  critical values, apply the Critical Value Method.
• Example. Solve 2x 6 gt 4. First, determine
  that the critical values are 1 and 5 (do you see
  why?).

11
Absolute Value in Equations and Inequalities We
discussed

• Two cases when absolute value brackets are
  removed
• Graphical interpretation of x lt a and x gt
  a.
• Critical value method for absolute value
  inequalities