An Application of MultiObjective Optimization to

1
An Application of Multi-Objective Optimization to
Portfolio Optimization Erica D. Parker,
Operations Research, North Carolina State
University, Raleigh NC 27695, edparker_at_ncsu.edu
Introduction Every investor is faced with a
risk-return tradeoff when allocating their funds
into various stocks and securities. Because
there are several patterns in which stocks tend
to follow (i.e. moving together, moving in
opposite directions, and not having any relation
to one another at all), investing is essentially
a case of trial and error. However, through the
utilization of mathematical models and
probabilistic rules, investing may not have to
be. 

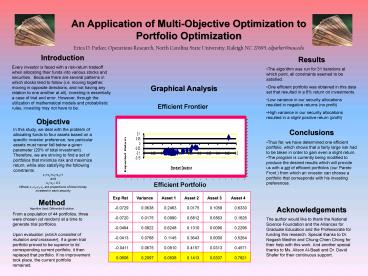
• Results
• The algorithm was run for 31 iterations at which
  point, all constraints seemed to be satisfied.
• One efficient portfolio was obtained in this data
  set that resulted in a 6 return on investments.
• Low variance in our security allocations resulted
  in negative returns (no profit)
• High variance in our security allocations
  resulted in a slight positive return (profit)

Graphical Analysis
Efficient Frontier
Objective In this study, we deal with the problem
of allocating funds to four assets based on a
specific investor preference two particular
assets must never fall below a given parameter
(20 of total investment). Therefore, we are
striving to find a set of portfolios that
minimize risk and maximize return, while also
satisfying the following constraints x1x2x3x4
1 and x1x2 0.2 Where x1,x2,x3,x4 are
proportions of total money invested in each
security

• Conclusions
• Thus far, we have determined one efficient
  portfolio, which shows that a fairly large risk
  had to be taken in order to gain even a slight
  return.
• The program is currently being modified to
  produce the desired results which will provide us
  with a set of efficient portfolios (our Pareto
  Front ) from which an investor can choose a
  portfolio that corresponds with his investing
  preferences.

Efficient Portfolio

• Method
• Algorithm Used Differential Evolution
• From a population of 44 portfolios, three were
  chosen (at random) at a time to generate trial
  portfolios.
• Upon evaluation (which consisted of mutation and
  crossover), if a given trial portfolio proved to
  be superior to its corresponding current
  portfolio, it then replaced that portfolio. If no
  improvement took place, the current portfolio
  remained.

Acknowledgements The author would like to thank
the National Science Foundation and the Alliances
for Graduate Education and the Professorate for
funding this research. Special thanks to Dr.
Negash Medhin and Chung-Chien Chong for their
help with this work. And another special thanks
to Ms. Alison Al-Baati and Dr. David Shafer for
their continuous support.