Traverse Calculations - PowerPoint PPT Presentation
1 / 18
Title:
Traverse Calculations
Description:
Double Meridian Distance. Use adjusted Lat's and Dep's. Meridian through west point. A. B. D. E. C. Use Lat, Dep to define triangles, trapezoids ... – PowerPoint PPT presentation
Number of Views:175
Avg rating:3.0/5.0
Title: Traverse Calculations
1
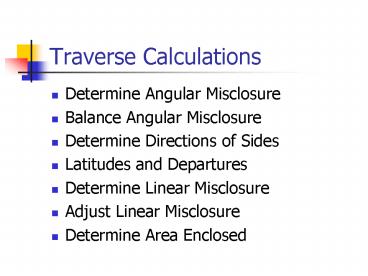
Traverse Calculations
- Determine Angular Misclosure
- Balance Angular Misclosure
- Determine Directions of Sides
- Latitudes and Departures
- Determine Linear Misclosure
- Adjust Linear Misclosure
- Determine Area Enclosed
2
Angular Misclosure
- Total (n-2)(180)
- n 5, Total 540
E
8626 10709 10825 9220
14537 53957 Misclosure -3 Acceptable?
D
9220
14537
A
8626
10825
C
1079
B
3
Balance Angular Error
- Contribution to Error
- Angles not consistent more work
- Assign contribution - C
- Correction C(-error/?C)
- Say angle A, B turned twice C,D, E 4 times
- A B are twice as likely to contribute to
errorCA CB 2, CC CD CE 1, ?C 7 - Correction C(3/7) 26C
- CorrA CorrB 51, CorrC CorrD CorrE
26 - Much easier if all angles contribute equally
4
Balancing Angular Error
8627 10709 10825 9221
14538 54000 OK!
- Assuming all work is consistent
- E error, n number of angles
- Correction -E/n
- Reflect precision
- C -(-3)/5 36
- Work recorded to 1
- Adjust 3 by 1
- Shortest shots
- Add 1 to A, E, D
D
E
9220
14537
A
8626
10825
C
1079
- Check that total works!
B
5
Determine Directions of Sides
- Use Adjusted Angles
- AzBC BackAzAB ABC
AZAB 14145 AZBC 32145 1079
6854 AZCD 24854 10825 35719 AZDE
17719 9221 26940 AZEA 8940
14538 23518 Check that last angle! AZAB
5518 8627 14145
D
E
9221
14538
A
8627
10825
1079
C
B
6
Latitudes and Departures
- Latitude LengthCos(Az or Bearing Angle)
- Departure LengthSin(Az or B.A.)
E
26940 502.06
LatAB 315.65 Cos(14145) -247.86
DepAB 315.65 Sin(14145)
195.40 Check your calculatorpolar -gt
rectangular key!
D
23518 187.05
35719 176.95
A
14145 315.62
C
6854 502.43
B
7
Latitudes and Departures
8
Determine Linear Misclosure
- You should end up where you started
- Sum of Lats 0
- Sum of Deps 0
- Linear Misclosure (error)
- A line connects starting and ending point
- Linear error length of line
9
Relative Error
- Is the linear error acceptable?
- Relative Error
- Relates error to total distance surveyed
- Expressed as 1/xxxx
10
Adjust Linear Error
- Transit rule
- When angles are more accurate than distances
- Proportion L error based on total N-S distance
- Proportion Dep error based on total E-W distance
- Compass Rule more common
- Assumes angles are as accurate as distances
- Proportion both errors based on total distance
- Least-Squares
- Uses square roots of sums of Lats and Deps
- Typically requires computer program
11
Adjust Linear Error
- Compass Rule
- Proportion Lat, Dep error to length of side
12
Adjusting Lats and Deps
13
Area by DMD
- Double Meridian Distance
- Use adjusted Lats and Deps
- Meridian through west point
E
D
- Use Lat, Dep to define triangles, trapezoids
- Note formulas
- A ½bh
- A ½b(h1h2)
- DMD double area
A
C
B
14
Area by DMD
- Process follows around the boundary
- DMDBC DMDAB DepAB DepBC
- Multiply DMD Lat for each side
- Add up Double area
- Divide total by 2
15
Areas by DMD
Area 321,352/2 160,676 s.f./43,560 3.69
acres
16
Coordinates
- Assign an origin W and S of point A
- N CoordB N CoordA LatAB
- E CoordB E CoordA DepAB
- Area by Coordinates
- Multiply E CoordA N CoordB, repeat, add
- Multiply E CoordB N CoordA, repeat, add
- 2A Difference of sums
17
Coordinates
Area (770,326 448,975)/2 160,676 s.f.
/43,560 3.69 acres
18
Why Use Coordinates?
- What line connects B and D?
- Lat ND NB 409.55 52.07 357.48
- Dep ED EB 755.84 295.39 460.45
- L (357.482 460.452)½ 582.93
- Az Tan-1(460.45/357.48) 521030
D
E
A
C
B