Uniform Acceleration - PowerPoint PPT Presentation
1 / 10
Title:
Uniform Acceleration
Description:
Sample problems #1: An HSE diver steps off the high dive and strikes the water ... Sample Problem #2: Determine the displacement of the diver. d = at2 vot ... – PowerPoint PPT presentation
Number of Views:33
Avg rating:3.0/5.0
Title: Uniform Acceleration
1
- Uniform Acceleration
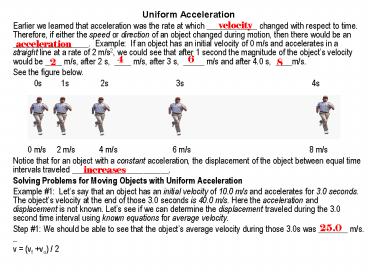
- Earlier we learned that acceleration was the rate
at which ____________ changed with respect to
time. Therefore, if either the speed or
direction of an object changed during motion,
then there would be an __________________.
Example If an object has an initial velocity of
0 m/s and accelerates in a straight line at a
rate of 2 m/s2, we could see that after 1 second
the magnitude of the objects velocity would be
____ m/s, after 2 s, ____ m/s, after 3 s, _____
m/s and after 4.0 s, ____m/s. - See the figure below.
- 0s 1s 2s
3s 4s - 0 m/s 2 m/s 4 m/s
6 m/s 8 m/s - Notice that for an object with a constant
acceleration, the displacement of the object
between equal time intervals traveled
_______________________. - Solving Problems for Moving Objects with Uniform
Acceleration - Example 1 Lets say that an object has an
initial velocity of 10.0 m/s and accelerates for
3.0 seconds. The objects velocity at the end of
those 3.0 seconds is 40.0 m/s. Here the
acceleration and displacement is not known. Lets
see if we can determine the displacement traveled
during the 3.0 second time interval using known
equations for average velocity. - Step 1 We should be able to see that the
objects average velocity during those 3.0s was
_______ m/s. _ - v (vf vo) / 2
velocity
acceleration
4
6
2
8
increases
25.0
2
- Step 2 The displacement could than be found
because we know that the object is in essence
traveling _______ m/s for 3.0 s. This would be
____________ m. - _
-
v d/t - d v t
- d 25.000 m/s(3.0 s)
- d 75.0 m
- We could have solved this problem in 1 step by
combining the 2 equations used above to obtain a
new equation. - v (vf vo ) d / t
- 2
- d (vf vo) t
- 2
- d (40.0 m/s 10.0 m/s) (3.0 s)
- 2
- d (50.0 m/s) (3.0 s) / 2
- d 75 m
25.0
75
_
_
3
- Example 2 Lets say that an object has an
initial velocity of 10.0 m/s and accelerates at a
rate of - 5.00 m/s2 for 3.00 s, determine the displacement
of the object using known equations for velocity
and acceleration. Here the final velocity and
displacement are not known. - Step 1 Here we can see that the final velocity
would be ________ m/s. - a (vf vo)
- t
- vf at vo
- vf (5.00 m/s2)(3.00 s) 10.0 m/s
- vf (15.000 m/s) 10.0 m/s
- vf 25.0 m/s
- Step 2 The average velocity of the object would
then be ________ m/s. - v (vf vo)
- 2
- v (25.000 m/s 10.0 m/s) / 2
- v (35.000 m/s) / 2
- v 17.5 m/s
25.0
17.5
_
_
_
_
4
- Step 3 The displacement of the object would
then be ________ m - v d/t
- d v t
- d (17.500 m/s)(3.00 s)
- d 52.5 m
- a 5.00 m/s2
- t 3.00 s
- vo 10.0 m/s
- vf wasnt given
- d we were to determine
52.5
_
_
Instead of using this 3 step thought process,
this problem can be calculated using 1 equation.
The equation can be derived using the equations
shown above.
vf vo d 2 t
a vf - vo t
vf at vo
at vo vo d 2 t
d ½ at2 vot
d ½ (5.00 m/s2)(3.00s)2 (10.0 m/s)(3.00s)
d 22.500 m 30.000 m
d 52.5 m
5
- Example 3 What if an object has an initial
velocity of 10.0 m/s and a final velocity of 60.0
m/s at an acceleration of 10.0 m/s2. Determine
the displacement of the object using known
equations for average velocity and acceleration.
Here, the time isnt known. - Step 1 Here, we could see that the average
velocity would be _____ m/s. - _
35.0
_
v (vf vo)
2
_
v (60.0 m/s 10.0 m/s)
2
_
v (70.0 m/s)
2
_
v 35.0 m/s
5.00
Step 2 We can also see that the object
accelerates for ________ s. a (vf - vo) t
t (vf - vo)
a
t (60.0 m/s 10.0 m/s)
10.0 m/s2
t (50.0 m/s)
10.0 m/s2
t 5.00 s
6
- Step 3 Therefore, the object travels __________
m.
175
_
d v t
d (35.000 m/s) (5.0000 s)
d 175 m
This problem can be solved using 1 equation
derived by combining the equations used above.
a (vf - vo) t
vf vo d 2 t
vo 10.0 m/s
vf 60.0 m/s
d vf2 vo2 2a
t (vf - vo) a
a 10.0 m/s2
vf vo d 2 (vf-vo) a
t not given
d (60.0 m/s)2 (10.0m/s)2 2(10.0 m/s2)
d ?
(vf vo) (vf vo) ad 2
d 3600.0 m2/s2 100.0 m2 /s2 2(10.0 m/s2)
(vf vo) (vf vo) 2 ad
d (3500.0 m2/s / 2(10.0 m/s2))
vf 2 vo2 2 ad
d 175 m
7
- Acceleration and Free Fall (pg. 17-24 pg 47-51)
- In free fall an object travels up or down with
only the force of ___________ acting upon it.
The force of gravity will cause the object to
accelerate towards earth at a rate of 9.80 m/s2,
meaning that every second the objects speed
changes by ________ m/s (that is if we can
neglect air resistance). - Sample problems 1 An HSE diver steps off the
high dive and strikes the water below 2.1 seconds
later. Determine divers speed at the time of
impact with the water. - vo 0 m/s
- t 2.1 s
- a 9.80 m/s2
- vf ?
- Sample Problem 2 Determine the displacement of
the diver. - d ½ at2 vot
- d ½ (9.80 m/s2)(2.1s)2
- d 22 m
- Sample Problem 3 King Kong holds Fay Wray at
the top of the Empire State Building. Her shoe
falls off and strikes the ground at 80.1 m/s.
Determine the displacement of the shoe during the
fall. - a 9.80 m/s2
- vf 80.1 m/s
- vo 0 m/s
- d ?
gravity
9.80
a vf vo / t vf at vo vf (9.80 m/s2)
(2.1s) vf 21 m/s
vf2 vo2 2ad d (vf2 vo2) / 2a d (80.1
m/s)2 / (2(9.80 m/s2)) d 327 m
8
- When an object is thrown straight upward (up
being the negative direction), the magnitude of
the velocity ___________. Upward velocity values
are given a __ sign (here the negative represents
direction). Downward velocity values are given a
__ sign. Further, upward displacements are __
while downward displacements are ___ (throwing up
is a negative thing!) - Example An object is thrown straight up with
the magnitude of its velocity being 29.4 m/s (the
complete velocity with sign would be ________
m/s). - 1.00 seconds after being thrown the object will
be traveling 9.80 m/s slower than it did the
previous second or ________ m/s (it travels
slower due to traveling in the opposite the
direction of the gravitational acceleration which
acts toward the earths center). - 2.00 seconds after being thrown the object will
be traveling 9.80 m/s slower than it did the
previous second or ______ m/s. - 3.00 seconds after being thrown the object will
be traveling 9.80 m/s slower than it did the
previous second or ______ m/s. - 4.00 seconds after being thrown the object will
be traveling 9.80 m/s faster than it did the
previous second or _______m/s (it travels faster
due to traveling in the downward direction or in
the same direction as the gravitational
acceleration). - 5.00 seconds after being thrown the object will
be traveling 9.80 m/s faster than it did the
previous second or ________m/s - 6.00 seconds after being thrown the object will
be traveling 9.80 m/s faster than it did the
previous second or _________m/s. (see fig. 2.6
pg. 18 3.8 pg. 50). Here the object would
return to your hand with the _________ magnitude
of velocity as when it left your hand but a
different _______ due to traveling in the
opposite direction. - Recall that the slope of a velocity vs. time
graph yields a value for the _________________. - Here, the acceleration (or slope of the line) of
the object is seen to be 9.80 m/s2 (a positive
value at all times). Even at the _________ of
the objects path when the velocity is zero, the
acceleration due to gravity is still positive
9.80 m/s2. Therefore we will __________use the
acceleration of gravity to be 9.80m/s2.
-
decreases
-
-29.4
-19.6
-9.8
0.0
9.8
19.6
29.4
same
sign
acceleration
top
always
9
- m (y2-y1)/(x2-x1)
- points (0.00 s, -29.4 m/s) and (3.00 s, 0.00
m/s) - y2 and x1 cancel so,
- m -y1 / x2
- m -(-29.4 m/s) / 3.00 s
- m 9.80 m/s2
Sample Problem 4 A Geyser in Yellowstone
National Park is capable of shooting water from
the ground with a speed of 48.0 m/s. Determine
the maximum upward displacement of the
water.
d ? vo - 48.0 m/s a 9.80 m/s2 vf
0 m/s
d (vf2 vo2) / 2a d (- 48.0 m/s)2 /
2(9.80m/s2) d - 118 m
10
- Sample Problem 5 A ball is thrown straight
upward at 39.2 m/s. Determine the velocity and
displacement 1.5s , 4.0s and 6.5 s after being
thrown. - vo -39.2 m/s vf at vo d
½ at2 vot - a 9.80 m/s2 vf (9.80 m/s2)(1.5s) (-39.2
m/s) d ½ (9.80 m/s2)(1.5s)2
(-39.2m/s)(1.5s) - t 1.5 s vf 14.70 m/s 39.2 m/s d
11.025 m 58.80 m - vf ? vf -25 m/s (-24.5 m/s) d
-48 m - d ?
- vo -39.2 m/s vf at vo d
½ at2 vot - a 9.80 m/s2 vf (9.80 m/s2)( 4.0s) (-39.2
m/s) d ½ (9.80 m/s2)(4.0s)2 (-39.2
m/s)(4.0s) - t 4.0 s vf 39.20 m/s 39.2 m/s d
78.4 m 156.8 m - vf ? vf 0. m/s d -80
m - d ?
- vo -39.2 m/s vf at vo d ½ at2
vot - a 9.80 m/s2 vf (9.80 m/s2)( 6.5s) (-39.2
m/s) d ½ (9.80 m/s2)(6.5s)2 (-39.2
m/s)(6.5s) - t 6.5 s vf 63.70 m/s 39.2 m/s d
207 m 254.8 m - vf ? vf 25 m/s d -50
m (-48 m) - d ?