Maxwell, time harmonic, transversetoz

1
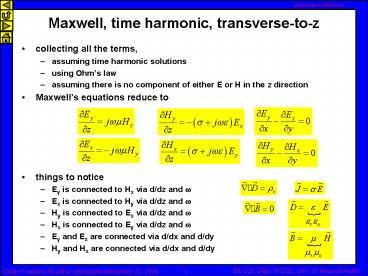
Maxwell, time harmonic, transverse-to-z

• collecting all the terms,
• assuming time harmonic solutions
• using Ohms law
• assuming there is no component of either E or H
  in the z direction
• Maxwells equations reduce to
• things to notice
• Ey is connected to Hx via d/dz and w
• Ex is connected to Hy via d/dz and w
• Hy is connected to Ex via d/dz and w
• Hx is connected to Ey via d/dz and w
• Ey and Ex are connected via d/dx and d/dy
• Hy and Hx are connected via d/dx and d/dy

2
Uniform plane wave solution to Maxwells equations

• the complete, time harmonic solution is
• g is called the complex propagation constant
• direction of propagation

3
Plane waves and boundaries

• we have found the TEM traveling wave solution
  to Maxwells equations that would work in a world
  that is made on one and only one material
• what would happen if there were a simple
  dielectric interface, i.e., half the world is
  filled with e1, and half is e2 ?
• we must still satisfy boundary conditions at the
  interface between the materials
• in anticipation of what we need to solve this
  problem, lets assume now that as a result of
  the incident wave there will be a transmitted
  wave and a reflected wave

medium 1 er1 mr1 s1
x
medium 2 er2 mr2 s2
y
z
4
Plane waves and boundaries

• as a result of the incident wave there will be a
  transmitted wave and a reflected wave

x
medium 1 er1 mr1 s1
y
medium 2 er2 mr2 s2
z
5
Fields at the interface

• the total fields at the interface between the two
  materials (i.e., at z0) are
• infinitesimally to the left of the interface
• infinitesimally to the right of the interface
• since the fields are tangential to the interface,
  and were assuming there is no surface current in
  this problem, the fields must be CONTINUOUS
  across the interface
• so we have two unknowns, Ereflect (Ex1o-) and
  Etransmit (Ex2o)

6
Transmitted and reflected fields

• two unknowns, two equations

7
Reflection coefficient

• we now have simple relation that gives the ratio
  of the reflected electric field to the incident
  electric field
• the reflection coefficient G is
• for our assumed coordinate system the sign of G
  will tells us which way the reflected electric
  field points
• Eincident pointed in the x direction
• if G is positive, then Ereflect also points in
  the x direction
• if G is negative, then Ereflect points in the -x
  direction

8
Transmission coefficient

• recall we had the equation from continuity of
  total tangential electric field at the interface,
  and we also have the reflection coefficient, so
• we define the transmission coefficient t to be
  the ratio of the transmitted electric field to
  the incident electric field

9
Magnetic fields

• recall we had the simple relation between the
  electric and magnetic fields
• so we can also define a magnetic field reflection
  coefficient
• and the transmitted magnetic field is

10
Power flow reflected power

• Poynting vectors at the interface (z 0)
• so the ratio of the incident power to the
  reflected power is

11
Power flow transmitted power

• Poynting vectors at the interface (z 0)
• so the ratio of transmitted power to incident
  power is

12
Example two lossless dielectrics

• assume the two materials are lossless, s 0, m
  mo
• so here
• limits
• er1 ltlt er2 ? G -1, t 0
• er1 gtgt er2 ? G 1, t 2

13
Example two lossless dielectrics

• assume the two materials are lossless, s 0, m
  mo
• lets assume that er1 lt er2

medium 1 e er1eo
medium 2 e er2eo
14
Example two lossless dielectrics, power flow

• assume two lossless materials, s 0, er1 , er2 ,
  m mo

15
Example two lossless dielectrics, power
conservation

• what happens if we add the reflected and
  transmitted powers?

16
Plane wave applets

• very nice, with ability to vary materials
  properties and frequency, includes power flow
• reflection at a dielectric interface
  http//www.amanogawa.com/archive/Oblique/Oblique-2
  .html
• index http//www.amanogawa.com/archive/wavesA.htm
  l
• this site is pretty useful for other things like
  transmissions lines
• they also have a pretty nice set of lecture slides

17
Power flow transmitted power

• Poynting vectors at the interface (z 0)
• so the ratio of transmitted power to incident
  power is