YIELD CRITERIA

1
YIELD CRITERIA
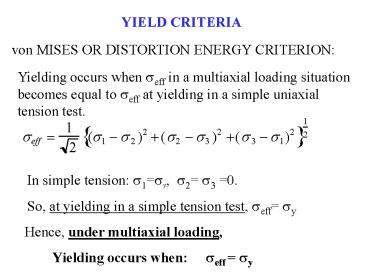
von MISES OR DISTORTION ENERGY CRITERION
Yielding occurs when seff in a multiaxial loading
situation becomes equal to seff at yielding in a
simple uniaxial tension test.
In simple tension s1sy, s2 s3 0. So, at
yielding in a simple tension test, seff sy
Hence, under multiaxial loading,
Yielding occurs when seff sy
2
YIELD CRITERIA
The von Mises criterion is also equivalent to the
octahedral criterion, which states that yielding
occurs when the total octahedral shear stress
(toct) on the octahedral plane reaches the value
that would occur in a simple tension test. Now
In simple tension,
3
BIAXIAL LOADING
s2 -s1
Torsion
s1
s30
4
VOLUME DOES NOT CHANGE DURING PLASTIC DEFORMATION
Assume principal coordinate system
e2
Final
e1
e(l-lo)/lo
e3
Sides of unit initial length Vo131
Length in x-direction 1(1e1) Length in
y-direction 1(1e2) Length in z-direction
1(1e3) So, V' (1e1) (1e2) (1e3)
dV/Ve1e2e30
Very Important
This derivation was done for small strains.
Using true strain definition, show that the above
relation also holds.
5
Principal coordinate system
BIAXIAL LOADING
s2 -s1
t1
t1
Torsion
s1
s1 t1
s1
Mohr's Circle Plane
s30
T
6
CONSEQUENCE OF DV/V0
Elastic Deformation
Recall from Invariant consideration
Note that subscripts 1,2, and 3 usually refer to
principal values.
Therefore,
Thus, plastic deformation is always associated
with an effective Poisson's contraction of 1/2
7
Levi - von Mises Equations In Plasticity
Note the Poisson's contraction of 1/2
8
PLASTICITY SOLUTION
Constitutive Behavior seffKeeffn
Levi-Mises Eqns
True Strain Eqns
True Stress Eqns
di
ei
eeff
seff
si
Pi
In the process, have to satisfy equilibrium,
compatibility, and boundary conditions. Arrow
directions vary with problem.
9
SOME COMMENTS

• In almost all the problems we will look at, the
  stress components are proportional to one
  another, and so are the strain components.
  Hence, the Levi-Mises equations can easily be
  integrated.

Thus, if
10
SOME COMMENTS
Similar for the other two strain components. One
will also find
11
EXAMPLE 1 TRIVIAL PROBLEM
2
Simple Uniaxial Tension (natural principal
coordinate system)
s1 s, e1 e
3
1
s2 s3 0
Could also get this from Levi-Mises equations.
In a simple tension test, we know that seff
s. Integrating, one gets e1 eeff, e2 e3-
eeff /2. Using the definition of eeff, one gets
eeff e
12
NORMALITY OF DEFORMATION (Not critical for this
course)
s3
s2
Yield surface, generated by drawing a cylinder at
equal angles to the three principal stress axis.
The resultant strain vector is perpendicular to
this surface. The radius of the cylinder is the
magnitude of the flow stress (stress at any point
in the plastic regime of the stress-strain curve).
s1
For a plane strain situation, we have e30. In
the s1- s2 plane, we have an ellipse.
The blue components show the signs and magnitudes
of the strain components
s2
Equi-biaxial Tension
1
p
e30.
Note that e20
3
2
s1
Plane strain tension
s1-p, s20 s3-p/2
Red arrows resultant strain vector
13
EXAMPLE 2 TORSION OF TUBE
Radius of tube 50 mm t5mm Applied Torque31.4
kN.m sy500 MPa Work hardening
seffKeeffn n0.2, K1100 MPa
F
tthickness
Determine if the tube has yielded, and what is
the angle of twist (F). Recall g r F/L.
Principal stresses (at 45 to tube) are
s1 t400, s2 -400 s30 MPa
14
EXAMPLE 2 TORSION OF TUBE
Integrating
rf/Lg. Therefore, f 0.17 radians9.7
degrees
g at 45 deg to princ. axis0.085
15
EXAMPLE 3 PLANE STRAIN COMPRESSION (Forging in
Die)
P
hf
ho
lo
lf
w
Given lo10 cm, ho5 cm, hf2.5 cm, w6 cm.
Material (6061-O, annealed) constitutive law
K205 MPa, n0.2 (pg.33).
Find the load at final h. Also, how much work is
done?
s1-p, s20
Where, p P/(lw)
e30. So, from the third Levi-Mises equation,
s3-p/2
16
EXAMPLE 3 PLANE STRAIN COMPRESSION
Since p is not known, we cannot proceed from left
to right. We have to proceed from the right.
Now e1ln (hf/ho) ln (2.5/5)-0.693 (69.3
deformation)
e30. Because e1 e2 e30, so e2-e1 0.693
17
EXAMPLE 3 PLANE STRAIN COMPRESSION
We get seff 196 MPa
Using the values of the stress components, we get
Therefore, p226 MPa Now, we need the area. The
width remained the same. We need the length.
Because lowholfwhf, so lf20cm. So, P lf w p
200 mm x60 mm x226 N/mm2 2712 kN609 kip
18
EXAMPLE 3 PLANE STRAIN COMPRESSION
We get, W 13.3 kJ. If operation done in 10 sec,
then Power 1.33 kW
19
EXAMPLE 3 PLANE STRAIN COMPRESSION
If n0, then note that
i.e., they provide the same amount of work.
20
EXAMPLE 4 BULGING OF CIRCULAR PLATE
to
Material (6061-O, annealed) constitutive law
K205 MPa, n0.2 (pg.33). Ro200 mm, to6 mm.
Find pressure needed to bulge plate to perfect
semicircle (q90 degrees).
21
EXAMPLE 4 BULGING OF CIRCULAR PLATE
Radius r Thickness t
po
sq
sq
Fupwards
The upward force is the projected area times the
pressure.
22
EXAMPLE 4 BULGING OF CIRCULAR PLATE
po
sq
q
q
sq
R
sqSinq
23
EXAMPLE 4 BULGING OF CIRCULAR PLATE
The rest of the problem can be easily solved.