74: Solving Compound Inequalities

1
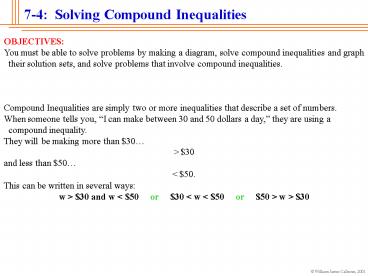
7-4 Solving Compound Inequalities
OBJECTIVES You must be able to solve problems by
making a diagram, solve compound inequalities and
graph their solution sets, and solve problems
that involve compound inequalities.
Compound Inequalities are simply two or more
inequalities that describe a set of numbers. When
someone tells you, I can make between 30 and 50
dollars a day, they are using a compound
inequality. They will be making more than 30 gt
30 and less than 50 lt 50. This can be written
in several ways w gt 30 and w lt 50 or
30 lt w lt 50 or 50 gt w gt 30
2
7-4 Solving Compound Inequalities
In compound inequalities, there will be two
conjunctions used

• and - used if both inequalities must be true
• The points where both inequalities are true are
  called intersections.
• or - used if either inequality must be true
• Both inequalities do not need to be true, just
  one.
• The trick will be finding out which conjunction
  to use in the problems.
• If it is or, the book will use the word or in
  the problem.
• If it is and, the book will either use the word
  and or give the solution set in a compound
  set-builder notation.
• Compound set-builder notation looks like this
• x -2 lt x lt 4

To start the examples, the book uses diagram
drawing as a method of problem solving. Remember
a picture is worth a thousand words!
3
7-4 Solving Compound Inequalities
EXAMPLE 1 On May 6, 1994, President Francois
Mitterrand of France and Queen Elizabeth II of
England officially opened the Channel Tunnel
connecting England and France. After the
ceremonies, a group of 36 English and French
government officials had dinner at a restaurant
in Calais, France, to celebrate the occasion.
Suppose the restaurant staff used small tables
that seat four people each, placed end to end, to
form on long table. How many tables were needed
to seat everyone?
tables
people
Use a diagram to solve this. Boxes will be
tables, Xs will be people at the table.
1
4
2
6
3
8
4
10
See the pattern? Use the pattern to solve without
drawing it all out.
17
36
It will take...
4
7-4 Solving Compound Inequalities
EXAMPLE 2 Graph the solution set of x ? -2 and
x lt 5.
The graph of x ? -2
The solution set is every point where the two
graphs overlap. That is what the and
means. Both inequalities must be true. The
solution set looks like this
The graph of x lt 5
In set-builder notation x -2 ? x lt 5.
5
7-4 Solving Compound Inequalities
EXAMPLE 3 Solve -1 lt x 3 lt 5. Then graph the
solution set.
This problem is actually two problems joined with
an and.
What is the letter? x On same side? positive
3 Get rid of it by subtracting 3 both sides.
What is the letter? x On same side? positive
3 Get rid of it by subtracting 3 both sides.
-4 lt x
x lt 2
The graph of x gt -4
The graph of x lt 2
The solution is the overlapping area.
In set-builder notation x -4 lt x lt 2.
6
7-4 Solving Compound Inequalities
EXAMPLE 4 Graph the solution set of x ? -1 or x
lt -3.
The graph of x ? -1
The graph of x lt -3
Since the problem has or in it, the solution
set is any point touched by either of the two
graphs. Therefore, the graph will include all
areas in red and look like this
In set-builder notation x x ? -1 or x lt
-3. Notice the set-builder notation is really no
different from the original problem.
7
7-4 Solving Compound Inequalities
EXAMPLE 5 Solve 3w 8 lt 2 or w 12 gt 2 -
w. Graph the solution set.
This is two different problems
3w lt -6
2w 12 gt 2
w lt -2
2w gt -10
The graph of w lt -2
w gt -5
The graph of w gt -5
The graph will include all areas in red and look
like this
Or simply
In set-builder notation x x is a real
number. Another way to give the answer is, all
solutions.
8
7-4 Solving Compound Inequalities
Need good review slide chart from first semester!
9
7-4 Solving Compound Inequalities
HOMEWORK
Page 410 19 - 39 odd