Physics 211 Lecture 16: Momentum and Collisions

1
Physics 211 Lecture 16 Momentum and Collisions

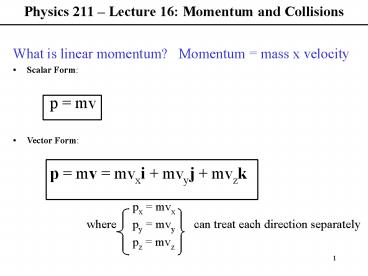
• What is linear momentum? Momentum mass x
  velocity
• Scalar Form
• p mv
• Vector Form
• p mv mvxi mvyj mvzk
• px mvx
• where py mvy can treat each direction
  separately
• pz mvz

2
Momentum relationship to force
Derivative of momentum equals force This means
forces cause changes in momentum
or
Impulse change in momentum

• Impulse-momentum theorem (integral form)
• Impulse-momentum theorem (average form)

If you dont know force as a function of time,
then an average force must be used.
3
Conservation of momentum - for any two objects
isolated from external forces, total momentum is
conserved .

• If they continue moving without any interaction
  (no collision)
• If they collide and stick together (completely
  inelastic collision)
• (note that kinetic energy is not conserved in
  this case)
• If they collide and bounce off each other
  perfectly (completely elastic collision)
• (note that kinetic energy is conserved in this
  case)

Conservation of momentum equations for two
particles
Vector form
Scalar form
where
treat each direction separately
4
Consv of mom in collisions one dimensional
inelastic
Diagram
Equations
mom. consv. eq., use x-direction   sub in
mom. formula   inelastic ? final speeds
equal   if know initial conditions sub in and
solve for vfx
5
Consv of mom in collisions one dimensional
elastic
Diagram
Equations
mom. consv. eq., use x-direction   Kinetic
energy consv eq   if know initial
conditions have two eqs/two unks to find v1fx and
v2fx Before/after rel. vel. is
equal/opposite
6
Consv of mom in collisions Two dimensional
General Diagram
Simplest Diagram (v2i 0 and v1 is along x axis)
General Equations
mom. consv. eq in x-direction   mom. consv.
eq in y-direction  
Simplest Equations
mom. consv. eq in x-direction   mom. consv.
eq in y-direction  
7
Consv of mom in collisions Two dimensional
DIFFICULTY for 2D calculations 4 unknowns, only
2 equations

• How to deal with specific 2D types of problems
• Look in problem statement for additional
  information
• Recognize results for specific types of
  collisions
• 1.      If perfectly inelastic
• a.       final x-velocities are equal
• b.      final y-velocities are equal
• c.       angles are equal
• 2.      If perfectly elastic
• a.       kinetic energy is also conserved gives
  another equation
• b.      Look for one extra piece of info given
  in problem to eliminate another
  unknown.
• 3.      If partially elastic
• a. Look for two extra pieces of info given
  in problem to
• eliminate two unknowns.

8
Example Ch9 4Two blocks of mass M 0.35kg and
3M are on horizontal, frictionless surface with
light spring attached to one and held together by
a cord that compresses the spring. When the cord
breaks, 3M moves right at 2m/s. A) what is
speed of block M?b) Find initial potential
energy stored in spring.
9
Example Ch9 93kg steel ball strikes vertical
wall at 10m/s and 60 angle to surface and
bounces off at equivalent angle. Ball contacts
wall for 0.2s. Find force exerted by ball on
wall.
10
Example Ch9 2890kg person runs E at 5m/s and
is tackled in an inelastic collision by 95kg
person running N at 3m/s.a) Find final speed
and direction of the two people.b) Find the
mechanical energy lost in the collision and state
where it went.
11
Questions for Groups

• 9.2 Speed of a particle is doubled.
• By what factor is momentum changed?
• By what factor is kinetic energy changed?
• 9.9 Ball of clay thrown against brick wall and
  sticks. Is the principle of conservation of
  momentum violated here? Why or why not?
• 9.13 Sharpshooter fires rifle with butt of gun
  against shoulder. Forward momentum of bullet
  then must equal backward momentum of rifle. So
  why isnt it so dangerous to be hit by the rifle
  butt rather than the bullet?