Time Value of Money

1
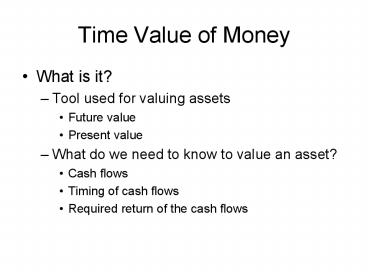
Time Value of Money

• What is it?
• Tool used for valuing assets
• Future value
• Present value
• What do we need to know to value an asset?
• Cash flows
• Timing of cash flows
• Required return of the cash flows

2

• Time lines show timing of cash flows.

0
1
2
3
r
CF0
CF1
CF3
CF2
Tick marks at ends of periods, so Time 0 is
today Time 1 is the end of Period 1 or the
beginning of Period 2.
3
Time line for a 100 lump sum due at the end of
Year 2.
0
1
2 Year
r
100
4
Time line for an ordinary annuity of 100 for 3
years.
0
1
2
3
r
100
100
100
5
Time line for uneven CFs -50 at t 0 and 100,
75, and 50 at the end of Years 1 through 3.
0
1
2
3
r
100
50
75
-50
6
Whats the FV of an initial 100 after 3 years if
r 10?
0
1
2
3
10
100
FV ?
Finding FVs is compounding.
FVn PV(1 r)t
7
Two Ways to Find FVs

• Solve the equation numerically with a regular
  calculator.
• Use a financial calculator.

8
Numerical solution

• It is very important to understand the numerical
  solutions to future and present value problems
• FVnPV(1r)n
• FV 100(1.10)3
• FV 1001.331 133.10

9
Financial Calculator Solution
Financial calculators solve this equation FVn
PV(1 r)t. There are 4 variables. If 3 are
known, the calculator will solve for the 4th.
10
Heres the setup to find FV
INPUTS
3 10 -100 0 N I/YR PV PMT FV
133.10
OUTPUT
Clearing automatically sets everything to 0, but
for safety enter PMT 0.
Set P/YR 1, END
11
Example

• Suppose that r invest 1000 today for retirement
  in an investment that pays 7 per year compound
  interest.
• What will this 1000 be worth in 30 years when I
  retire?
• What if interest rates were 14?

12
Example
0
1
...
30
7
1000
FV ?

• FV30 1000(1.07)30 10007.6123 7612.30

13
Example
INPUTS
30 7 -1000 0 N I/YR PV
PMT FV 7612.30
OUTPUT
14

Problems

• You wan to buy a house in 3 years. You plan to
  save 5000 in the first year and anticipate that
  the amount you can save will increase by 10 per
  year. If interest rates are 7, how much money
  will you have for a down payment in three years?

15
Whats the PV of 100 due in 3 years if r 10?
Finding PVs is discounting, and its the reverse
of compounding.
0
1
2
3
10
100
PV ?
16
Present value

• PV value today of a future cash flow or series
  of cash flows
• Opportunity cost rate the rate of return on the
  best available alternative investment of equal
  risk
• Equilibrium value price at which investors are
  indifferent between buying and selling a security

17
Solve FVn PV(1 r )t for PV
3
1
ö
æ
(
)

PV

100


100
PVIF
ç
ø
è
r,
t
1.10
(
)


100
0.7513


75.13.
18
Financial Calculator Solution
INPUTS
3 10 0 100 N I/YR PV PMT FV
-75.13
OUTPUT
Either PV or FV must be negative. Here PV
-75.13. Put in 75.13 today, take out 100
after 3 years.
19
Example

• Suppose that you are expecting an investment to
  yield 10,000 five years from now.
• The opportunity cost of similar investments is
  5.
• How much should you pay for this investment
  today?
• What if the opportunity cost was 10?
• What if you got 10,000 in 10 years instead of 5
  years?

20
Example
0
1
5
2
3
4
5
10000
PV ?
5
1
ö
æ
PV



10000.7835
ç

10000
ø
è
1.05


7835
21
Example
INPUTS
5 5 0 10000 N I/YR PV PMT FV
-7835
OUTPUT
22
If sales grow at 20 per year, how long before
sales double?
Solve for t
FVn 1(1 r)t 2 1(1.20)t
Use calculator to solve.
INPUTS
20 -1 0 2 N I/YR PV PMT FV
3.8
OUTPUT
23
Solving for t mathematically

• FVn PV(1r)t
• FVn/PV (1r)t
• ln(FVn/PV) tln(1r)
• ln(FVn/PV)/ln(1r) t
• ln(2)/ln(1.2) .6931/.1823 3.8

24

Examples

• The Rule of 72 says that with discrete
  compounding the time it takes for an investment
  to double in value is roughly 72/r. If the
  annually compounded rate of interest is 10
  estimate how long it will take for money to
  double using the rule of 72 and find the exact
  time that it will take.

25
Solving for r

• Suppose that we can buy a security for 78.35
  that will pay 100 after five years. What is the
  interest rate that we would earn on this
  investment?

26
Solving for r

• Using financial calculator

INPUTS
5 -78.35 0
100 N I/YR PV PMT FV 5

OUTPUT
27
Solving for r

• FV PV(1r)t
• FV/PV (1r)t
• (FV/PV)1/t (1r)
• (FV/PV)1/t 1 r
• (100/78.35)1/5 1 .05

28
Whats the FV of a 3-year annuity of 100 at 10?
0
1
2
3
10
100
100
100
110 121 FV 331
29
Financial Calculator Solution
INPUTS
3 10 0 -100 331.00
I/YR
N
PMT
FV
PV
OUTPUT
Have payments but no lump sum PV, so enter 0 for
present value.
30
Mathematical analysis of the future value of
annuities

• FVAn PMT(FVIFAr,t)

31
Example

• Suppose that I invest 1000 a year in a
  retirement account that pays 7 interest
  annually.
• What will my retirement account be worth in 30
  years? 40 years?
• What if interest rates were 14?

32
Example

• FVIFA7,30 (1.07)30 - 1/.07 94.461
• FVA 100094.461 94,461

INPUTS
30 7 0 -1000 94,461
I/YR
N
PMT
FV
PV
OUTPUT
33
Whats the PV of this annuity?
0
1
2
3
10
100
100
100
90.91
82.64
75.13
248.68 PV
34
INPUTS
3 10 100 0
N
I/YR
PV
PMT
FV
OUTPUT
-248.69
Have payments but no lump sum FV, so enter 0 for
future value.
35
Mathematical analysis of the present value of
annuities

• PVAt PMT(PVIFAr,t)

36
Example

• Suppose that when I retire I want to have 40,000
  a year to live on for 25 years. My investment
  company is offering 5 on such an annuity.
• How much money will I need when I retire to
  purchase this annuity?

37
Example

• PVIFA5,25 1 (1/(1.05)25)/r
• 20 - 5.9061 14.0939
• PVA 40,00014.0930 563,756

INPUTS
25 5 40000 0
N
I/YR
PV
PMT
FV
OUTPUT
-563,756
38
Finding the Number of Payments

• Suppose you borrow 2000 at 5 and you are going
  to make annual payments of 734.42. How long
  before you pay off the loan?

INPUTS
-2,000
5 734.42 0
N
I/YR
PV
PMT
FV
OUTPUT
3
39
Whats the difference between an ordinary annuity
and an annuity due?
Ordinary Annuity
0
1
2
3
r
PMT
PMT
PMT
Annuity Due
0
1
2
3
r
PMT
PMT
PMT
40
Find the FV and PV if theannuity were an annuity
due.
0
1
2
3
10
100
100
100
41
Switch from End to Begin. Then enter
variables to find PVA3 273.55.
INPUTS
3 10 100 0 -273.55

N
I/YR
PV
PMT
FV
OUTPUT
Then enter PV 0 and press FV to find FV
364.10. Or solve as an ordinary annuity and
multiply by (1r)
42

Problems

• A factory costs 800,000. It is expected that
  the factory will produce an inflow after
  operating costs of 170,000 a year for 10 years.
  If the opportunity cost of capital is 14, what
  is the value of this factory today?

43

Example

• A 15 year security has a price of 340.5869. The
  security pays 50 at the end of each of the next
  5 years and then it pays a different cash flow in
  each of the years 6-15. Interest rates are 9.
  What is the annual cash flow in years 6-15?

44
Example

• Suppose that you want to retire in 30 years.
  When you retire, you want to purchase an annuity
  that will pay you 40,000 a year for 20 years
  after you retire. (Assume the payments begin in
  the year after you retire). If you can earn 10
  on all of your investments, how much do you have
  to save each year in order to be able to purchase
  your desired retirement annuity?

45
What is the PV of this uneven cashflow stream?
4
0
1
2
3
10
100
300
300
-50
90.91
247.93
225.39
-34.15
530.08 PV
46
Will the FV of a lump sum be larger or smaller if
we compound more often, holding the stated r
constant? Why?
LARGER! If compounding is more frequent than
once a year--for example, semiannually,
quarterly, or daily--interest is earned on
interest more often.
47
0
1
2
3
10
100
133.10
Annually FV3 100(1.10)3 133.10.
0
1
2
3
0
1
2
3
4
5
6
5
134.01
100
Semiannually FV6 100(1.05)6 134.01.
48
We will deal with 3 different rates APR
nominal, or stated, or quoted, rate per
year. PER periodic rate APR/m. EAR

effective annual rate.
49

• APR is stated in contracts. Periods per year (m)
  must also be given.
• Examples
• 8 Quarterly
• 8, Daily interest (365 days)

50

• Periodic rate PER APR/m, where m is number of
  compounding periods per year. m 4 for
  quarterly, 12 for monthly, and 360 or 365 for
  daily compounding.
• Examples
• 8 quarterly PER 8/4 2.
• 8 daily (365) PER 8/365 0.021918.

51

• Effective Annual Rate (EAR )
• The annual rate which causes PV to grow to the
  same FV as under multi-period compounding.
• Useful for comparisons
• An investment with monthly payments is different
  from one with quarterly payments. Must put on
  EAR basis to compare rates of return.

52
How do we find EFF for a nominal rate of 10,
compounded semiannually?
53
EAR of 10
EARAnnual 10. EARQ (1 0.10/4)4 - 1
10.38. EARM (1 0.10/12)12 - 1
10.47. EARD(360) (1 0.10/360)360 - 1
10.52.
54
Whats the value at the end of Year 3of the
following CF stream if the quoted interest rate
is 10, compounded semiannually?
4
5
0
1
2
3
6 6-mos. periods
5
100
100
100
55

• Payments occur annually, but compounding occurs
  each 6 months.
• So we cant use normal annuity valuation
  techniques.

56
Compound Each CF
0
1
2
3
4
5
6
5
100
100.00
100
110.25
121.55
331.80
FVA3 100(1.05)4 100(1.05)2 100 331.80.
57
Whats the PV of this stream?
0
1
2
3
5
100
100
100
90.70 82.27 74.62 247.59
58
Example

• What is the present value of an ordinary annuity
  that makes 1,000 payments each year and earns
  12 interest compounded semiannually?

59
Example, Retirement Problem Revisited

• Suppose that you want to retire in 30 years.
  When you retire, you want to purchase an annuity
  that will pay you 40,000 a year for 20 years
  after you retire. (Assume the payments begin in
  the year after you retire). If you can earn 10
  compounded monthly on all of your investments,
  how much do you have to save each month for the
  next 30 years in order to be able to purchase
  your desired retirement annuity?

60

Example

• You want to sell on credit, giving customers 3
  months to pay. However, you will have to borrow
  from the bank to carry the accounts payable. The
  bank charges a 15 nominal rate with monthly
  compounding. What nominal rate should you quote
  to your customers to break even?

61

Continuous Compounding

• Effective annual rate using continuous
  compounding
• EAR er - 1
• FVIFi,t using continuous compounding
• FVIFi,t eit
• PVIFi,t using continuous compounding
• PVIFi,t e-it

62

Continuous Compounding
EARAnnual 10. EARQ (1 0.10/4)4 - 1
10.38. EARM (1 0.10/12)12 - 1
10.47. EARD(360) (1 0.10/360)360 - 1
10.515. EARcont e.10 - 1
10.517
63
Amortization
Construct an amortization schedule for a 1,000,
10 annual rate loan with 3 equal payments.
64
Step 1 Find the required payments.
0
1
2
3
10
PMT
PMT
PMT
-1,000
3 10 -1000
0
INPUTS
N
I/YR
PV
FV
PMT
OUTPUT
402.11
65
Step 2 Find interest charge for Year 1.
INTt Beg balt (r) INT1 1,000(0.10) 100.
Step 3 Find repayment of principal in
Year 1.
Repmt PMT - INT 402.11 - 100
302.11.
66
Step 4 Find ending balance after
Year 1.
End bal Beg bal - Repmt 1,000 -
302.11 697.89.
Repeat these steps for Years 2 and 3 to complete
the amortization table.
67
BEG PRIN END YR BAL PMT INT PMT BAL
1 1,000 402 100 302 698 2 698 402 70 332 36
6 3 366 402 37 366 0 TOT 1,206.34 206.34 1,000
Interest declines. Tax implications.
68

• Amortization tables are widely used for home
  mortgages, auto loans, business loans, retirement
  plans, etc. They are very important!
• Financial calculators (and spreadsheets) are
  great for setting up amortization tables.