Mixtures of Substances

1
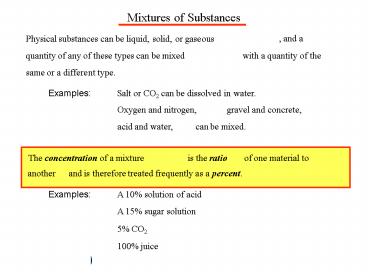
Mixtures of Substances
Physical substances can be liquid, solid, or
gaseous
Examples
Salt or CO2 can be dissolved in water.
Oxygen and nitrogen,
gravel and concrete,
acid and water,
can be mixed.
The concentration of a mixture
is the ratio
and is therefore treated frequently as a percent.
Examples
A 10 solution of acid
A 15 sugar solution
5 CO2
100 juice
2
Mixtures of Merchandise
Nuts, grains,
noodles, nails,
can be mixed.
gasoline.
Examples
Trail mix,
granola,
The price of each of the products mixed is a ratio
(dollars per lb,
dollars per gallon),
as is the price of the mixture that results.
Coins, interest rates, driving speeds,
as products priced in the aggregate.
To calculate values of ingredients or mixtures of
quantities like these, one must decide what and
when to multiply or divide.
3
Questions About Mixtures
Common questions about mixtures that have
concentrations are
1. What is the concentration
of the mixture that results
when mixtures of different concentrations
are combined?
2. How much of one mixture
with a particular concentration?
Common questions about mixtures of merchandise
are
1. What is the price
(per pound, for example)
that have different prices (per pound)?
2. How much of an item at one price
with an item at another price
to create a mixture
at a particular price?
4
Concentration Example
A chemist mixes 100 ml of 15 HCl (hydrochloric
acid) with 150 ml of 20 HCl. What is the
concentration of the mixture?
Note 1,000 ml (milliliters) 1 L (liter)
Use a table to organize known and unknown values.
100 ml
15
15 ml
150 ml
20
30 ml
45 ml
250 ml
?
Amount Concentration Volume
Total volume of the mixture
and total amount of acid in it
are known.
Concentration of the mixture
More than 15. Less than 20.
5
How Much Water to Add
A pharmacy keeps a large stock of 25 benzadryl
on hand.
How much stock and how much water should be mixed
to fill an order for 200 ml of a 2 solution?
Create a table and fill in what is known.
x
25
200 - x
0
0 ml
200 ml
2
Hint
The amount of drug in the final mixture is 0.2
200 ml.
Let x be volume of stock.
Amount of drug in x stock is 0.25 x .
0.25 x 4 ml
Solving
Make 250 ml instead.
Mix 16 ml of stock with 184 ml of water.
6
Mixing Nuts
A grocer charges 5.75 per lb for cashews
Create a table and fill in what is known.
x
5.75
2 - x
1.80
2
3.38
and total prices.
Let x be lbs of cashews.
Fill in cashew,
peanut,
Total price is also 6.75.
Solve for x.
Make 1½ lbs worth 6.65.
6.75 3.95 x 3.6
He should mix 0.8 lbs of cashews with 1.2 lb of
peanuts.
7
PRACTICE
8
(No Transcript)
9
(No Transcript)
10
(No Transcript)
11
(No Transcript)
12
(No Transcript)
13
(No Transcript)
14
(No Transcript)