Other Equilibrium Relationships

1
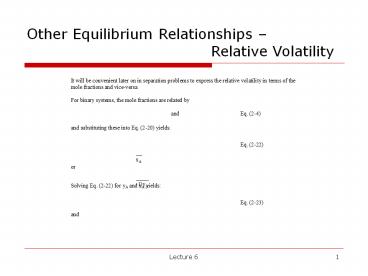
Other Equilibrium Relationships Relative
Volatility
2
Calculation of Bubble-Point and Dew-Point
Temperatures

• The bubble-point temperature is the temperature
  at which a liquid mixture begins to boil.
• The dew-point temperature is the temperature at
  which a vapor mixture first begins to condense.

3
Temperature-Composition Diagram
for Ethanol-Water, P 1 atm
100
95
Two Phase
Superheated Vapor Phase
90
C)
o
T(
85
80
Subcooled Liquid Phase
75
zEtOH
0.0
0.2
0.4
0.6
0.8
1.0
x
or y
EtOH
EtOH
4
Calculation of Bubble-Point Temperatures

• If one is given a liquid mixture, one often needs
  to determine the bubble-point temperature of the
  mixture.
• We have done this to date using equilibrium data
  for binary systems for example, from the
  saturated liquid line on a T vs. x,y plot for a
  given feed composition, zi.
• How do we handle multi-component systems?

5
Calculation of Bubble-Point Temperatures

• If the feed is in the liquid phase, the pressure
  p and the composition, xis, of the liquid phase
  will be given.
• One then needs to determine the bubble-point
  temperature of the mixture.

6
Calculation of Bubble-Point Temperatures
Where do we start?

• Well, we are given the liquid-phase mole
  fractions, and we know that the mole fraction
  relationships for both the liquid and vapor phase
  are given by Eq. (2-13)

7
Calculation of Bubble-Point Temperatures
Equilibrium Relationship

• We are given the liquid-phase mole fractions,
  xis, and we need to link the vapor-phase mole
  fractions, yis, to the liquid-phase mole
  fractions to do this, one can use the
  definition for the equilibrium distribution
  coefficient K, Eq. (2-10) and solve for yi

or
8
Calculation of Bubble-Point Temperatures
Equilibrium Distribution Coefficient Relationship

• Substituting Eq. (2-10) into Eq. (2-13) yields
• K is a function of both temperature and pressure,
  Eq. (2-11)

9
Calculation of Bubble-Point Temperatures
Equilibrium Distribution Coefficient T,P
Relationship

• One needs an expression for K as a function of T
  and P.
• One convenient expression for K as a function of
  T and P for light hydrocarbons is the DePriester
  equation, Eq. (2-12)

10
Calculation of Bubble-Point Temperatures
Analytical Expression

• Substituting the Depriester equation, Eq. (2-12),
  into
• yields the analytical expression for
  bubble-point calculations

11
Calculation of Bubble-Point Temperatures

• One must solve the bubble-point expression for T.
• There are several ways to solve for T
• 1.) If the expression for K is simple enough,
  one may be able to algebraically solve for T
  e.g., if some of the constants in the
  DePriester equation are 0.
• 2.) One may use a trail and error method as
  outlined in Fig. 2-13, Wankat, p. 29.
• 3.) One may solve numerically.

12
(No Transcript)
13
Calculation of Dew-Point Temperatures

• If the feed is in the vapor phase, the pressure p
  and the composition, yis, of the vapor phase
  will be given.
• One then needs to determine the dew-point
  temperature of the mixture.

14
Calculation of Dew-Point Temperatures

• Just as one may derive the bubble-point
  temperature relationship, one can use a similar
  derivation using the equilibrium coefficient
  equation definition, Eq. (2-10), but this time
  solving for xi, since one would be given the
  vapor-phase mole fractions, yis
• or

15
Calculation of Dew-Point Temperatures
Equilibrium Distribution Coefficient Relationship

• Substituting Eq. (2-10) into Eq. (2-13) yields
• K is a function of both temperature and pressure,
  Eq. (2-11)

16
Calculation of Dew-Point Temperatures Analyti
cal Expression

• Substituting the Depriester equation, Eq. (2-12),
  into
• yields the analytical expression for
  dew-point calculations

17
Calculation of Bubble-Point and Dew-Point
Temperatures Numerical Solutions

• One can conveniently use numerical methods for
  these types of problems, using, for example,
  Mathcad.
• Mathcad uses non-linear numerical methods such as
  the Quasi-Newtonian or Levenberg-Marquardt
  algorithms to solve equations.

18
A Final Note!

• While we will solve bubble-point and dew-point
  temperature problems for a given pressure, there
  is no reason why this same methodology cannot be
  applied to determining bubble-point and dew-point
  pressure problems for a given temperature!