Postulates

1
Postulates
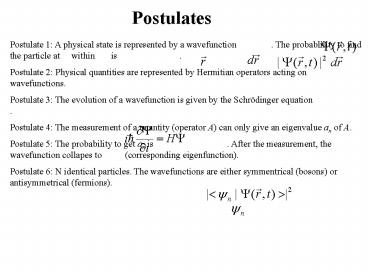
Postulate 1 A physical state is represented by a
wavefunction . The probablility to
find the particle at within is
. Postulate 2 Physical
quantities are represented by Hermitian operators
acting on wavefunctions. Postulate 3 The
evolution of a wavefunction is given by the
Schrödinger equation
. Postulate 4 The measurement of a quantity
(operator A) can only give an eigenvalue an of
A. Postulate 5 The probability to get an is
. After the
measurement, the wavefunction collapes to
(corresponding eigenfunction). Postulate 6 N
identical particles. The wavefunctions are either
symmentrical (bosons) or antisymmetrical
(fermions).
2
Quantum mechanics
If H is time-independent Time-independent
Schrödinger equation H F E F Y(t)F
e-iEt
There exists a common set of orthormal
egenfunctions
A, B, C, ... Commutating Hermitian operators
3
Orbital angular momentum
circ. perm. Commutation relations
Eigenfunctions common to L2, Lz Spherical
harmonics
integers
Orthonormality
Raising, lowering operators
4
One particle in a spherically symmetric potential
H, L2, Lz commute
Eigenfunctions common to H, L2, Lz
Degeneracy
Centrifugal potential
Wavefunctions parity
5
Angular momentum
circ. perm. Commutation relations
Eigenfunctions common to J2, Jz
Integers or half-integers
Angular momentum
Addition of two angular momenta
Triangle rule
L2, Lz ,S2, Sz commute
L2, S2, J2, Jz commute
Clebsch-Gordan coefficients
6
One particle in a spherically symmetric potential
Eigenfunctions common to H, L2,
Lz , S2, Sz
Eigenvalues
Eigenfunctions common to H, L2,
S2, J2, Jz
Eigenvalues
Also eigenfunctions to the spin-orbit interaction
7
Time-independent perturbation theory
known
? Approximation ?
Non-degenerate level
Degenerate level (s times)
First diagonalize H in the subspace
corresponding to the degeneracy
8
Time-dependent perturbation theory
known
System in a at t0 Probability to be in b at time
t?
Constant perturbation switched on at t0
Continuum of final states with an energy
distribution rb(E), width h
Fermis Golden rule
For
9
One particle in an electromagnetic field (I)
Plane wave
b
a
Absorption
Line broadening
Stimulated emission
10
One particle in an electromagnetic field (II)
b
a
Dipole approximation
Absorption
Selection rules
Oscillator strength
11
One particle in a magnetic field
Zeeman effect
Paschen-Back effect
Anomal Zeeman effect
12
One particle in an electric field
Quadratic Stark effect (ground state)
Linear Stark effect
Tunnel ionisation
13
Many-electron atom
P identical particles
y antisymmetrical or symmetrical
/permutation of two electrons
Postulate 6 N identical particles. The
wavefunctions are either symmetrical (bosons) or
antisymmetrical (fermions).
y antisymmetrical
14
Many-electron atom
Hc central field
H1 perturbation
Slater determinant Pauli principle
Electron configuration, periodic system etc..
terms
Wavefunctions common to Hc, L2, Lz, S2, Sz
2S1L
15
Many-electron atom
Hc central field
H1 perturbation
antisymmetrical/ permutation of two
electrons
Slater determinant Pauli principle
Electon configuration, periodic system etc..
Wavefunctions common to Hc, L2, Lz, S2, Sz
2S1L Beyond the central field
approximation
terms
LS coupling
jj coupling