Sn

1
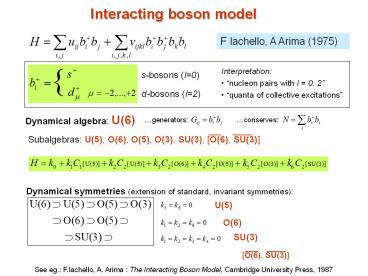
Interacting boson model
F Iachello, A Arima (1975)

• Interpretation
• nucleon pairs with l 0, 2
• quanta of collective excitations

s-bosons (l0)
d-bosons (l2)
Dynamical algebra U(6)
generators
conserves
Subalgebras U(5), O(6), O(5), O(3), SU(3),
O(6), SU(3)
Dynamical symmetries (extension of standard,
invariant symmetries)
U(5)
O(6)
SU(3)
O(6), SU(3)
See eg. F.Iachello, A. Arima The Interacting
Boson Model, Cambridge University Press, 1987
2
triangle(s)
D Warner, Nature 420, 614 (2002).
Parameter space of the model simplest version
(IBM-1) can be imaged as the surface of a
symmetry pyramide.
Corresponding points in various triangles are
connected by similarity transformations
(parameter symmetries), so it is sufficient to
investigate dynamics in one of the triangles.
3
Simplified Hamiltonian
ensures that the thermodynamic
limit exists N?8
d-boson number operator
quadrupole operator
scaling constant h?1 MeV
control parameters ?, ?
symmetry triangle
4
Phase diagram
obtained from
variational procedure based on condensate-type
ground-state wave functions
critical exponent
1st order
2nd order
order parameter ß0 spherical,
ßgt0 prolate, ßlt0 oblate. I
II III
1st order
5
Classical limit of IBM1
d-boson number
The Hamiltonian with s- and d- bosons
quadrupole operator
scaling constant a N/10 MeV
Classical Limit obtained by Glauber coherent
states
If restricted to L0 states, Hamiltonian is
solely a function of quadrupole deformation
parameters ß ? (in the intrinsic frame) -gt 2D
system
6
Classical potential in case of prolate deformation
x
?
y
Particularly important values of energy
y
section through the plane y 0
x
7
Chaos within the Triangle

• Standard classical measures of chaos
• Lyapounov exponents ?
  D(t) ... separation of two
  neighbouring trajectories at time t
• Fraction of chaotic phase space volume s

New highly regular arc discovered
Alhassid,Whelan PRL 67 (1991) 816 using both
measures chaotic volume s and average maximal
Lyapounov exponent ?.
Fraction of chaotic phase space s at two values
of angular momentum. The arc is clearly visible
in both pictures. adapted from Alhassid,Whelan
PRL 67 (1991) 816
8
Chaos within the Triangle

• Standard quantum measures of chaos

• Brody parameter ? (distribution of nearest
  neighbor level separation S)
• Distribution of B(E2) strengths (Porter-Thomas
  distribution) (Alhassid,Whelan)
• ?3 statistics (long range spectral correlations)
  (Alhassid,Whelan)
• Wave function entropy (localisation in
  dynamical-symmetry bases) (Cejnar,Jolie)

interpolates between Poisson (?0, regular
dynamics) and Wigner distribution (?1, chaos)