Buoyant force

1
Buoyant force
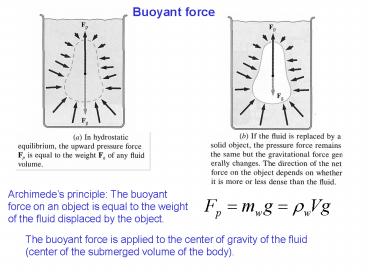
Archimedes principle The buoyant force on an
object is equal to the weight of the fluid
displaced by the object.
The buoyant force is applied to the center of
gravity of the fluid (center of the submerged
volume of the body).
2
Equal Volumes Feel Equal Buoyant Forces Suppose
you had equal sized balls of cork, aluminum and
lead, with respective densities of 0.2, 2.7, and
11.3 times the density of water. If the volume of
each is 10 cubic centimeters then their masses
are 2, 27, and 113 gram.
Each would displace 10 grams of water, yielding
apparent masses of -8 (the cork would accelerate
upward), 17 and 103 grams respectively (and
weights of -0.08, 0.17 and 1.03 N). Apparent
mass can be defined as apparent weight divided by
the gravitational acceleration, g.
3
Archimedes principleThe buoyant force on an
object is equal to the weight of the fluid
displaced by the object.
Ship empty.
Ship loaded with 50 ton of iron.
Ship loaded with 50 ton of styrofoam.
Volume of the submerged part of the ship (or any
other floating object) is equal to the mass of
the ship divided by the density of water
weight of the ship
buoyancy force,balancing the weight
4
Ice cube in a glass of water
Water level, h?
After the piece of ice melts
5
Center of gravity vs. center of buoyancy
Gravitational force is applied at the center of
gravity.Buoyancy force is applied to the center
of submerged part the center of
buoyancy.Center of gravity should be below
center of buoyancy for stable equilibrium.
Is that a necessary condition of equilibrium?
There is something wrong with the picture on the
left What?
Center of buoyancy is the center of volume of the
submerged part of the boat. It cannot possibly be
at or above the water-line!
6
What about a raft?
Is its center of gravity situated below the
center of buoyancy?
How come, those people are so careless and are
not afraid to turn over?
7
Steady flow in a river.
Velocity in each point is shown by a vector with
the length proportional to the velocity. Velocity
gets higher, where the river gets narrower.
Flow represented by streamlines, that are
everywhere tangent to flow direction. Higher
density of the streamlines corresponds to higher
flow velocity.
In a steady flow there are no variations in
velocity and pattern of flow in time.
Nevertheless, the actual fluid elements flowing
past any particular point at different times are
always different. The fluid elements also get
accelerated and decelerated as they move along
the streamlines.
8
Motion of fluids obeys the standard laws of
mechanics.
Newtons second law
Becomes Navier-Stokes equation
Any way to make our life easier?!Lets try to
use the laws of conservation!!
9
Motion of fluids obeys the standard laws of
mechanics.
Conservation of mass
Conservation of momentum
Conservation of energy
Using the laws of conservation means doing
appropriate bookkeeping and doing algebra instead
of solving differential equations!
10
Flow tube -
Steady flow
A small tubelike region bounded on its sides by a
continuous set of streamlinesand on its ends by
small areas at right angles to the streamlines.
Cross-section areas on the left and right ends
areA1 and A2.Densities and velocities arer1,
r2 and v1, v2
11
Mass of fluid entering the tube from the left
over the time interval Dt
Steady flow
By mass conservation, over the time interval Dt,
the same mass is exiting the tube from the right
Therefore
everywhere along a flow tube
If the fluid is incompressible and its density,
r, is constant, we have
12
Does it work for traffic?
Once you pass the spot of accident there are more
lanes available (larger A) and the traffic speeds
up (higher v).What is the matter?
Traffic is highly compressible.You have got to
use
13
How does the total energy of a small fluid
element change, as it moves inside the flow tube
from cross-section 1 to cross-section 2?
Kinetic energy
Potential energy
14
How does this change in the total energy become
possible? There are external forces originating
from pressure of the liquid outside the tube,
which do work on the fluid element!
Positive work as it enters from the left
Negative work as it exits from the right
The total energy balance
15
The total energy balance
Incompressible fluids constant density and
volume
Bernoullis equation