Producing the Product Rule

1
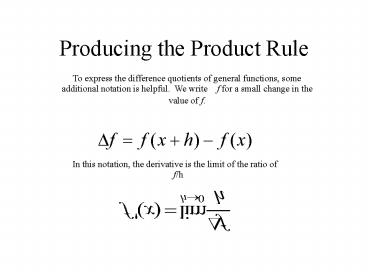
Producing the Product Rule
To express the difference quotients of general
functions, some additional notation is helpful.
We write ?f for a small change in the value of f.
In this notation, the derivative is the limit of
the ratio of ?f/h
2
Producing the Product Rule
(Area of whole rectangle)-(unshaded area)
Area of the three shaded rectangles
g(x)
g(xh)
3
Producing the Product Rule
Now we divide both sides by h
To evaluate the limit as h approaches 0, lets
examine the three terms on the right separately
and
4
Producing the Product Rule
In the third term, we multiply the top and bottom
by h to get
Then by taking the limit of each part of this, as
h approaches 0, we get
So.
5
The Product Rule
or
6
Another Way of Saying it
If uf(x) and vg(x), then
In other words, the derivative of a product of
two functions is the derivative of the first
times the second, plus the first times the
derivative of the second.
7
Practicing the product rule
Differentiate each of the following
8
The Quotient Rule
This is not as rigorous as finding the product
rule, but we use the product rule in it to find
the rule.
Our Mission differentiate
(where Q(x) is differentiable)
9
Finding the Quotient Rule
So, solving for Q(x) we get
10
Finding the Quotient Rule
And by multiplying the top and bottom by g(x) to
simplify we get
To simplify
11
The Quotient rule

• Using the lyrics from an old Cab Calloway song,
  with Ho meaning Low and Hi being High, it goes
  like this
• Ho-de-hi minus Hi-de-ho over Ho-Ho

12
Practicing the Quotient RuleFind the derivative
of each of the following