Quantum Mechanics of Atoms

1
Chapter 28 Quantum Mechanics of Atoms
2
Units of Chapter 28

• Quantum Mechanics A New Theory
• The Wave Function and Its Interpretation the
  Double-Slit Experiment
• The Heisenberg Uncertainty Principle
• Philosophic Implications Probability versus
  Determinism
• Quantum-Mechanical View of Atoms
• Quantum Mechanics of the Hydrogen Atom Quantum
  Numbers

3
Units of Chapter 28

• Complex Atoms the Exclusion Principle
• The Periodic Table of Elements
• X-Ray Spectra and Atomic Number
• Fluorescence and Phosphorescence
• Lasers
• Holography

4
28.1 Quantum Mechanics A New Theory
Quantum mechanics incorporates wave-particle
duality, and successfully explains energy states
in complex atoms and molecules, the relative
brightness of spectral lines, and many other
phenomena. It is widely accepted as being the
fundamental theory underlying all physical
processes.
5
28.1 Quantum Mechanics A New Theory
Quantum mechanics is essential to understanding
atoms and molecules, but can also have effects on
larger scales.
6
28.2 The Wave Function and Its Interpretation
the Double-Slit Experiment
An electromagnetic wave has oscillating electric
and magnetic fields. What is oscillating in a
matter wave?
7
28.2 The Wave Function and Its Interpretation
the Double-Slit Experiment
This role is played by the wave function, ?. The
square of the wave function at any point is
proportional to the number of electrons expected
to be found there. For a single electron, the
wave function is the probability of finding the
electron at that point.
8
28.2 The Wave Function and Its Interpretation
the Double-Slit Experiment
For example the interference pattern is observed
after many electrons have gone through the slits.
If we send the electrons through one at a time,
we cannot predict the path any
single electron will take, but we can predict the
overall distribution.
9
28.3 The Heisenberg Uncertainty Principle
Quantum mechanics tells us there are limits to
measurement not because of the limits of our
instruments, but inherently.
10
28.3 The Heisenberg Uncertainty Principle
This is due to the wave-particle duality, and to
interaction between the observing equipment and
the object being observed.
11
28.3 The Heisenberg Uncertainty Principle
Imagine trying to see an electron with a powerful
microscope. At least one photon must scatter off
the electron and enter the microscope, but in
doing so it will transfer some of its momentum to
the electron.
12
28.3 The Heisenberg Uncertainty Principle
The uncertainty in the momentum of the electron
is taken to be the momentum of the photon it
could transfer anywhere from none to all of its
momentum. In addition, the position can only be
measured to about one wavelength of the photon.
13
28.3 The Heisenberg Uncertainty Principle
Combining, we find the combination of
uncertainties
This is called the Heisenberg uncertainty
principle. It tells us that the position and
momentum cannot simultaneously be measured with
precision.
14
28.3 The Heisenberg Uncertainty Principle
This relation can also be written as a relation
between the uncertainty in time and the
uncertainty in energy
This says that if an energy state only lasts for
a limited time, its energy will be uncertain. It
also says that conservation of energy can be
violated if the time is short enough.
15
28.4 Philosophic Implications Probability versus
Determinism
The world of Newtonian mechanics is a
deterministic one. If you know the forces on an
object and its initial velocity, you can predict
where it will go.
16
28.4 Philosophic Implications Probability versus
Determinism
Quantum mechanics is very different you can
predict what masses of electrons will do, but
have no idea what any individual one will.
17
28.5 Quantum-Mechanical View of Atoms
Since we cannot say exactly where an electron is,
the Bohr picture of the atom, with electrons in
neat orbits, cannot be correct.
Quantum theory describes an electron probability
distribution this figure shows the distribution
for the ground state of hydrogen
18
28.6 Quantum Mechanics of the Hydrogen Atom
Quantum Numbers

• There are four different quantum numbers needed
  to specify the state of an electron in an atom.
• Principal quantum number n gives the total
  energy

19
28.6 Quantum Mechanics of the Hydrogen Atom
Quantum Numbers
2. Orbital quantum number l gives the angular
momentum l can take on integer values from 0 to
n - 1.
(28-3)
3. The magnetic quantum number, ml, gives the
direction of the electrons angular momentum, and
can take on integer values from l to l.
20
28.6 Quantum Mechanics of the Hydrogen Atom
Quantum Numbers
This plot indicates the quantization of angular
momentum direction for l 2. The other two
components of the angular momentum are undefined.
21
28.6 Quantum Mechanics of the Hydrogen Atom
Quantum Numbers
4. The spin quantum number, ms, which for an
electron can take on the values ½ and -½. The
need for this quantum number was found by
experiment spin is an intrinsically quantum
mechanical quantity, although it mathematically
behaves as a form of angular momentum.
22
28.6 Quantum Mechanics of the Hydrogen Atom
Quantum Numbers
This table summarizes the four quantum numbers.
23
28.6 Quantum Mechanics of the Hydrogen Atom
Quantum Numbers
The angular momentum quantum numbers do not
affect the energy level much, but they do change
the spatial distribution of the electron cloud.
24
28.6 Quantum Mechanics of the Hydrogen Atom
Quantum Numbers
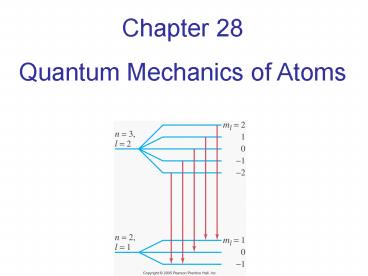
Allowed transitions between energy levels occur
between states whose value of l differ by one
Other, forbidden, transitions also occur but
with much lower probability.
25
28.7 Complex Atoms the Exclusion Principle
Complex atoms contain more than one electron, so
the interaction between electrons must be
accounted for in the energy levels. This means
that the energy depends on both n and l. A
neutral atom has Z electrons, as well as Z
protons in its nucleus. Z is called the atomic
number.
26
28.7 Complex Atoms the Exclusion Principle
In order to understand the electron distributions
in atoms, another principle is needed. This is
the Pauli exclusion principle No two electrons
in an atom can occupy the same quantum state. The
quantum state is specified by the four quantum
numbers no two electrons can have the same set.
27
28.7 Complex Atoms the Exclusion Principle
This chart shows the occupied and some
unoccupied states in He, Li, and Na.
28
28.8 The Periodic Table of the Elements
We can now understand the organization of the
periodic table. Electrons with the same n are in
the same shell. Electrons with the same n and l
are in the same subshell. The exclusion
principle limits the maximum number of electrons
in each subshell to 2(2l 1).
29
28.8 The Periodic Table of the Elements
Each value of l is given its own letter symbol.
30
28.8 The Periodic Table of the Elements
Electron configurations are written by giving the
value for n, the letter code for l, and the
number of electrons in the subshell as a
superscript. For example, here is the
ground-state configuration of sodium
31
28.8 The Periodic Table of the Elements
This table shows the configuration of the outer
electrons only.
32
28.8 The Periodic Table of the Elements
Atoms with the same number of electrons in their
outer shells have similar chemical behavior. They
appear in the same column of the periodic table.
33
28.8 The Periodic Table of the Elements
The outer columns those with full, almost full,
or almost empty outer shells are the most
distinctive. The inner columns, with partly
filled shells, have more similar chemical
properties.
34
28.9 X-Ray Spectra and Atomic Number
The effective charge that an electron sees is
the charge on the nucleus shielded by inner
electrons. Only the electrons in the first level
see the entire nuclear charge.
35
28.9 X-Ray Spectra and Atomic Number
The energy of a level is proportional to Z2, so
the wavelengths corresponding to transitions to
the n 1 state in high-Z atoms are in the X-ray
range.
36
28.9 X-Ray Spectra and Atomic Number
Inner electrons can be ejected by high-energy
electrons. The resulting X-ray spectrum is
characteristic of the element.
This example is for molybdenum.
37
28.9 X-Ray Spectra and Atomic Number
Measurement of these spectra allows determination
of inner energy levels, as well as Z, as the
wavelength of the shortest X-rays is inversely
proportional to Z2.
38
28.9 X-Ray Spectra and Atomic Number
The continuous part of the X-ray spectrum comes
from electrons that are decelerated by
interactions within the material, and therefore
emit photons. This radiation is called
bremsstrahlung (braking radiation).
39
28.10 Fluorescence and Phosphorescence
If an electron is excited to a higher energy
state, it may emit two or more photons of longer
wavelength as it returns to the lower level.
40
28.10 Fluorescence and Phosphorescence
Fluorescence occurs when the absorbed photon is
ultraviolet and the emitted photons are in the
visible range.
41
28.10 Fluorescence and Phosphorescence
Phosphorescence occurs when the electron is
excited to a metastable state it can take
seconds or more to return to the lower state.
Meanwhile, the material glows.
42
28.11 Lasers
A laser produces a narrow, intense beam of
coherent light. This coherence means that, at a
given cross section, all parts of the beam have
the same phase.
The top figure shows absorption of a photon. The
bottom picture shows stimulated emission if the
atom is already in the excited state, the
presence of another photon of the same frequency
can stimulate the atom to make the transition to
the lower state sooner. These photons are in
phase.
43
28.11 Lasers

• To obtain coherent light from stimulated
  emission, two conditions must be met
• Most of the atoms must be in the excited state
  this is called an inverted population.

44
28.11 Lasers
2. The higher state must be a metastable state,
so that once the population is inverted, it stays
that way. This means that transitions occur
through stimulated emission rather than
spontaneously.
45
28.11 Lasers
The laser beam is narrow, only spreading due to
diffraction, which is determined by the size of
the end mirror.
An inverted population can be created by exciting
electrons to a state from which they decay to a
metastable state. This is called optical pumping.
46
28.11 Lasers
A metastable state can also be created through
interactions between two sets of atoms, such as
in a helium-neon laser.
47
28.11 Lasers
Lasers are used for a wide variety of
applications surgery, machining, surveying,
reading bar codes, CDs, and DVDs, and so on. This
diagram shows how a CD is read.
48
28.12 Holography
Holograms are created using the coherent light of
a laser. The beam is split, allowing the film to
record both the intensity and the relative phase
of the light. The resulting image, when
illuminated by a laser, is three-dimensional.
49
28.12 Holography
White-light holograms are made with a laser but
can be viewed in ordinary light. The emulsion is
thick, and contains interference patterns that
make the image somewhat three-dimensional.
50
Summary of Chapter 28

• Quantum mechanics is the basic theory at the
  atomic level it is statistical rather than
  deterministic
• Heisenberg uncertainty principle

• Electron state in atom is specified by four
  numbers, n, l, ml, and ms

51
Summary of Chapter 28

• n, the principal quantum number, can have any
  integer value, and gives the energy of the level
• l, the orbital quantum number, can have values
  from 0 to n 1
• ml, the magnetic quantum number, can have values
  from l to l
• ms, the spin quantum number, can be ½ or -½
• Energy levels depend on n and l, except in
  hydrogen. The other quantum numbers also result
  in small energy differences.

52
Summary of Chapter 28

• Pauli exclusion principle no two electrons in
  the same atom can be in the same quantum state
• Electrons are grouped into shells and subshells
• Periodic table reflects shell structure
• X-ray spectrum can give information about inner
  levels and Z of high-Z atoms