Chapter 5 Discrete and Continuous Probability Distributions

1
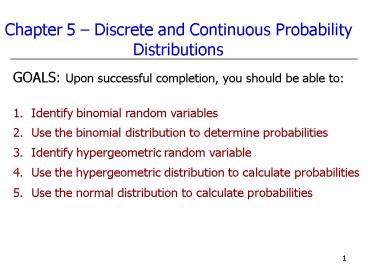
Chapter 5 Discrete and Continuous Probability
Distributions

• GOALS Upon successful completion, you should be
  able to
• Identify binomial random variables
• Use the binomial distribution to determine
  probabilities
• Identify hypergeometric random variable
• Use the hypergeometric distribution to calculate
  probabilities
• Use the normal distribution to calculate
  probabilities

2
The Binomial Probability Distribution

• Properties of a Binomial Experiment
• Sequence of n identical trials.
• Two outcomes, success and failure, are possible
  on each trial.
• The probability of a success, denoted by p, does
  not change from trial to trial.
• The trials are independent.

3
Example Company Insurance

• Company records indicate that 90 of the
  employees annually use the companys health
  insurance plan.
• Suppose 3 employees are randomly selected and
• x the number using the insurance.
• Is x a binomial random variable?

4
Example Company Insurance

• Binomial Probability Properties Apply?
• 3 identical trials n3
• Two outcomes, success use insurance Y and
  failure do not use insurance N, are possible
  on each trial.
• The probability of a success, denoted by p .90,
  does not change from trial to trial.
• The trials are independent?

5
Example Company Insurance

• So, what is the probability that just 1 of the 3
  employees will use the company insurance plan?
• That is,
• P(x1 n3, p.90) ?

6
Example Company Insurance Tree Diagram
7
Example Company Insurance

• P( N1N2Y3 N1Y2N3 Y1N2N3 )
• (.1)(.1)(.9)
• (.1)(.9)(.1)
• (.9)(.1)(.1) .027

8
The Binomial Probability Function

• where
• P(x) the probability of x successes in n
  trials
• n the number of trials
• p the probability of success on any one
  trial
• q 1 - p

9
Example Company Insurance

• Using the Binomial Probability Function
• (3)(0.90)(0.01)
• .027

10
Example Company Insurance

• Using the Tables of Binomial Probabilities
• see page 731

11
The Binomial Probability Distribution

• Expected Value E(x) ? n p
• Variance Var(x) ? 2 n p q
• Standard Deviation

12
Example Company Insurance

• Expected Value
• E(x) ? 3(.9) n p 2.7 employees
• Variance
• ? 2 n p q 3(.9)(.1) .27
• Standard Deviation

13
The Hypergeometric Probability Distribution

• Closely related to the binomial distribution.
• Trials are not independent, and the probability
  of success changes from trial to trial.

14
The Hypergeometric Probability Function

• for 0
• where
• f(x) probability of x successes in n
  trials
• n number of trials
• N number of elements in the population
• r number of elements in the
  population labeled success

15
Florida Lotto Ticket - Back
16
Florida Lotto 5 of 6
17
Normal Distribution Characteristics

• Symmetric and Bell-shaped
• Location determined by the mean, m, which can be
  any numerical value -, 0,
• Spread determined by the standard deviation, s
• Mean, median and mode at the center
• Area under curve equivalent to probability
• Total area 1 .5 on each side

18
Normal Probability Distribution

• where
• ? mean
• ? standard deviation
• ? 3.14159
• e 2.71828

19
Normal Curves Different ?, Same ?
s 0.5
s 0.5
? 12
? 20
20
Normal Curves Same ?, Different ?
s 0.5
s 1.0
? 20
21
Recall the Empirical Rule

• µ 1s ? About 68 of the data
• µ 2s ? About 95 of the data
• µ 3s ? About 99.7 of the data

22
Empirical Rule

• So, if µ 10 and s 2,
• then 68 of the data will be between 8 and 12
• then 95 of the data will be between 6 and 14
• then 99.7 of the data will be between 4 and 16

23
What about other probabilities?

• If µ 10 and s 2,what percentage of the data
  will be between 7 and 13?

24
What about other probabilities?

• If µ 10 and s 2, what percentage of the data
  will be between 7 and 13?
• Solution, integrate
• from 7 to 13.

25
Calculating Z-scores
26
Standard Normal Distribution

• Normal Distribution with
• µ 0 and s 1
• Also called the z-distribution.

27
Standard Normal Distribution

• Integrate the z-distribution ? table of
  probabilities
• Convert other normal distributions to the
  z-distribution to determine probabilities.

28
Standard Normal Table

• Table of probabilities from the mean to some
  positive z-value page 743

29
Calculating Normal Probability

• GE fluorescent light bulbs have an average life
  of 1000 hours with a standard deviation of 50
  hours.
• What percentage of light bulbs last between 1000
  hours and 1080 hours?

1000 1080
30
Calculating Normal Probability
1000 1080
X
31
Calculating Normal Probability
What percentage of light bulbs last more than
1080 hours?
.5000 - .4452 .0548
32
Calculating Normal Probability
What percentage of light bulbs last less than
1080 hours?
.5000 .4452 .9452
33
Calculating Normal Probability
What percentage of light bulbs last between than
1080 and 1100 hours?
.4772 - .4452 .0320
34
Finding a Percentile

• DieHard makes a car battery with an average life
  of 96 months and a standard deviation of 6
  months.
• What is the value that separates the 5 of the
  batteries that have the shortest lifespan?
• What is the 5th percentile?

35
Finding a Percentile

• Here we know µ and s. We are looking for the
  value of x that corresponds to the 5th
  percentile.

36
Finding a Percentile

• Find the 80th percentile.

X
37

• End
• of
• Chapter 5