Electromagnetic Devices and Optics - PHY743 -

1
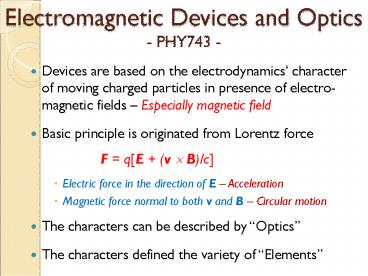
Electromagnetic Devices and Optics- PHY743 -

• Devices are based on the electrodynamics'
  character of moving charged particles in presence
  of electro-magnetic fields Especially magnetic
  field
• Basic principle is originated from Lorentz force
• F qE (v ? B)/c
• Electric force in the direction of E
  Acceleration
• Magnetic force normal to both v and B Circular
  motion
• The characters can be described by Optics
• The characters defined the variety of Elements

2
Magnetic Dipole

• Circular motion of charged particle in uniform B
  field

Circular Motion

• Radius in meter
• P Momentum in GeV/c
• B Field in Tesla (kGauss)

?
?
? is a function of momentum p
3
Magnetic Dipole Cont.

• Momentum Dispersion by Magnetic Dipole
• Function of Magnetic Dipole
• Change charged particles trajectory orientation
• Disperse trajectory orientation according to
  momentum

Magnetic Dipole
Optics Prism
Wavelength Dispersion
Momentum Dispersion
4
Magnetic Dipole Cont.

• Basic Structure of a Dipole

H Dipole

• Small uniform field area but small size
• Suitable for small particle trajectory
• profile Beam Line Element or
• Special application

• Large uniform field area
• Suitable for large particle trajectory
• profile - Spectrometer

5
Magnetic Dipole Cont.

• Effective Field Boundary (EFB)

Bx and Bz are mot zero in fringe field region
I ?BydZ
Boundary shaping outlined by EFB line and
detailed F.F.D. are important parameters for
design and optical description of a dipole
6
Magnetic Dipole Cont.

• Important Optical Parameters for a Dipole
• B0 and L (path length)
• ? and ?
• These are first order parameters
• ? and ?
• Shaping of EFBs
• Fringe field description
• These are second and higher order parameters

7
Magnetic Quadrupole

• Basic Structure of a Quadrupole
• York iron with 4 inner circular
• symmetric poles
• Four sets of connected coils
• Field flux flows oppositely
• Up-Down and Left-Right
• B 0 at r 0, Bmax at r R

R
8
Magnetic Quadrupole Cont.

• It works just like an optical lens
• Quadrupole magnet Magnetic Lens

Quadrupole focuses the charged particles.
Multipoles and quadrupoles are needed to focus
the particles in full phase space
9
Magnetic Multipoles

• Magnetic Multipoles have the same concept as
  Quadrupole except number of poles
• They are
• Hexapole (Axial Symmetry 2nd order in optics)
• Octapole (Point Symmetry 3rd order in optics)
• Decapole (Axial Symmetry 4th order in optics)
• Dodecapole (Point Symmetry 5th order in optics)
• Hardware Hexapole

Others defects Combined asymmetries, imperfect
individual pole location and rotation, and
imperfect pole face curvatures. These are
unavoidable.
10
Magnetic Multipoles Cont.

• Quadrupoles are used for beam line and
  spectrometer to confine or focus the beam profile
  since Dipole changes the profile size due to
  incident angle and momentum spreads
• Hexapoles are used commonly in beam line to
  control the beam profile at hardware level
• Multipole Fields from spectrometer Quadrupoles
  are commonly described or corrected in the
  Optics description
• Optical Parameters for Quadrupole and Multipoles
• Tip field strength Bmax, radius R, and
  effective length L (1st order)
• Strength of Multipole field contents (2nd and
  higher orders)
• Fringe field distribution description (2nd and
  higher orders)

11
Electric Separator Velocity Separator

• Used to separate particles w/ the same momentum,
  i.e. purify the secondary beam content
• Basic Structure
• Location and size of the slit selects the
  particles
• Optical Parameters Effective path length L
  and Ex (first order)
• Gap and width of electrodes and fringe
  field
• (Higher orders)

12
Solenoids

• Commonly used for collision physics or large
  acceptance reactional or decay physics
• Basics structure (Assuming for reactional or
  decay physics)
• Optical parameters
• Length of solenoid
• Diameter of solenoid
• Asymptotic magnetic field of solenoid, i.e. B
  0.4?IN/L

13
Example The Hadron Hall at J-PARCPut All The
Elements Together for Hadronic Beam Lines
Secondary lines for ?, K, or p beam
14
Example Continuous Electron Beam Accelerator
Facility (CEBAF)
15
Example Continuous Electron Beam Accelerator
Facility (CEBAF)

• ARCs and Hall A/C lines require a series of beam
  line dipoles to separate passes and reorient the
  beam direction
• Many quadrupoles and multipoles are required to
  confine the beam profile, remittance, achromatic
  in momentum at target

16
Example Hall C at Jlab (CEBAF)
HMS
SOS
They form specialized magnetic optical
instruments that analyze the momentum of the
scattered charged particles from the experimental
target
17
Matrix Representation of Magnetic OpticsUsing
Spectrometer at CEBAF as Example

• Coordinate Matrices
• At target Xt (xt, xt, yt, yt, 0, ?p), xt
  yt 0 for point target
• At focal plane Xfp (xfp, xfp, yfp, yfp, L,
  ?p), measured at focal plane
• x and y are the angles in dispersion and
  non-dispersion planes
• ?p is momentum in with respect to the central
  momentum
• Transportation Matrices Representing the
  Optical Character of the Spectrometer System
• M Forward optical matrix from target to focal
  plane
• M-1 Backward optical matrix from focal plane to
  target
• Matrix Representation of Optical Transportation
  and Reconstruction
• Forward Xfp M Xt Backward Xt M-1
  Xfp
• ?p can be found when M (or M-1) and the rest
  elements in Xt, and Xfp matrices are known, i.e.

?p F(known coordinates) where F is also
written in matrix
At CEBAF xt F(known coordinates and ?p)
yt F(known coordinates and ?p)
Reconstruction matrices, F, F, and F, are all
derived from M or M-1
18
Matrix Representation of Magnetic Optics Cont.

• By Polynomial expansion, M is written in series
  of orders in which the 1st order matrix
  represents the basic optical nature of a
  specifically designed spectrometer.
• 1st order matrix M(6x6) Using 1,2,3,4,5,6 for
  x, x, y, y, L, ?p
• Each element represents an Amplification or a
  Correlation from individual Xt to Xfp
  coordinates

19
Matrix Representation of Magnetic Optics Cont.

• Example
• R11 and R33 are xfp/xt and yfp/yt ratios, i.e.
  image (or spot size) Amplifications

20
Matrix Representation of Magnetic Optics Cont.

• Example Cont.
• R12 and R34 are xfp/xt and yfp/yt, i.e.
  Correlation dependence of image or spot size at
  FP to the incident angular acceptance xt and
  yt.

21
Matrix Representation of Magnetic Optics Cont.

• Example Cont.
• Element R16 (?p/xt) represents the enlarged image
  size due to momentum accaptance or bite.
• D/M R11/R16 defines an important character for
  a spectrometer Momentum Dispersion in unit of
  cm/. In principle, the larger D/M the better
  momentum resolution for a spectrometer.

22
Matrix Representation of Magnetic Optics Cont.

• General considerations of a specific optical
  system
• Optimize all first order parameters, including
  all drift spaces to achieve specific optical
  features for a system
• D/M for required momentum resolution of a
  spectrometer
• To achieve Point-to-point focusing, minimize R12
  and R34, i.e. no angular and size correlations
  Better momentum resolution.
• To achieve Point to Parallel focusing, minimize
  R22 and R44, i.e. no angular and angular
  correlations Better angular acceptance but poor
  1st order focusing.

23
Matrix Representation of Magnetic Optics Cont.

• General considerations of a specific optical
  system Cont.
• Mixed Point-to-Point in x but Point-to-Parallel
  in y. Enhance resolution by good D/M and x
  focusing but increase angular acceptance from y.
• Achromatic optics for beam line R16 ? 0 (or
  D/M ? 0)
• To minimize the beam size and dispersion to
  connect optical systems or
• send beam on experimental target.
• Issues to be considered for a spectrometer
• Momentum resolution
• Momentum and angular acceptances
• Total path length
• Focal plane size
• Total spin precession for polarized particle

R44 ? 0
24
Higher Order of Electro-magnetic Optics

• First order optics defines the intrinsic and
  general features of an optical system (a
  spectrometer or a sub-section of beam line). It
  is an ideal approximation that analogs to the
  small lens approximation of optics.
• Higher order optics come from non-ideal features
  of a system, thus represent the realities.
  Inclusion of higher order matrices in M is to
  reproduce the Real Optics of a Realistic
  system. Therefore, it is extremely important and
  crucial to evaluate and obtain the realistic
  higher order optics in order for the system to
  work or achieve the design goal.
• The sources contributed to higher order optics
• Fringe field effect from each electro-magnetic
  element
• Dipole EFB boundary shape and non-parallel of
  dipoles
• Asymmetries from symmetric elements
• Alignment errors and relative rotations between
  elements
• Precision of field setting
• Field interference between elements

25
Higher Order of Electro-magnetic Optics Cont.

• Higher order matrix elements
• 2nd order Rijk, i, j, k 1 6, e.g.
  RxxyR224
• Total of 63/2 elements
• 3rd order Rijkl, i, j, k, l 1 6, e.g.
  Rxxyy R2234
• Total of 64/22 elements
• 4th order Total of 65/23 elements
• Number of orders needed 6 10 for accuracy
• Number of elements Often more than thousand

26
Summary of Magnetic Optics

• Magnetic devices and systems are similar as
  optical components and systems, such as
• Quadra-poles ? Lens and Dipole ? Prism,
• Magnetic devices and systems can be designed and
  used based on magnetic optics. Commonly used
  optics software are
• Transport Up to third orders, used for basic
  design, obtain matrix
• Turtle Use matrix to evaluate profiles to
  optimize acceptance
• Raytrace Describe field up to fifth orders,
  use field map to evaluate realistic optics
• COSY Combined all above, include higher orders
  and obtain matrix
• Accurate optical matrix is essential for
  designing and using the magnetic systems beam
  line and spectrometer