ELECTRICITY AND MAGNETISM - PowerPoint PPT Presentation
1 / 172
Title:
ELECTRICITY AND MAGNETISM
Description:
No work is done in moving a test charge between any two points on an equipotential surface. ... If two point charges are separated by a distance r12, the ... – PowerPoint PPT presentation
Number of Views:396
Avg rating:3.0/5.0
Title: ELECTRICITY AND MAGNETISM
1
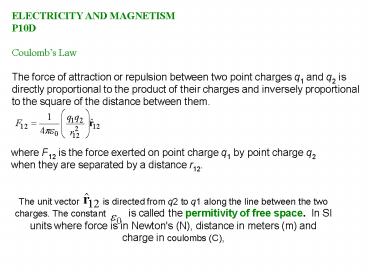
ELECTRICITY AND MAGNETISM P10D Coulombs
Law The force of attraction or repulsion
between two point charges q1 and q2 is directly
proportional to the product of their charges and
inversely proportional to the square of the
distance between them.
where F12 is the force exerted on point charge q1
by point charge q2 when they are separated by a
distance r12.
The unit vector is directed from q2 to q1
along the line between the two charges. The
constant is called the permitivity of
free space. In SI units where force is in
Newton's (N), distance in meters (m) and charge
in coulombs (C),
2
Topic Outline
Potential difference and Electric
Potential Potential Difference in Uniform
Electric Field Electric Potential and Potential
Energy due to a Point Charge Obtaining the value
of the electric field from electric potential.
Electric Potential Due to Continuous Charge
Distribution Electric Potential Due to a Charged
Conductor The Millikan Oil - Drop
Experiment Applications of Electrostatics
3
Electric Potential
- Electrostatic Force given by Coulombs law is
conservative ? can use the idea of electric
potential - Scalar quantity
- Can use it to describe electrostatic phenomenon
more simply than electric field and electric
force
4
Electric Potential Energy
- Test charge qo placed in an electric field E
created by some other charged object. We get
electric force qoE. - Coulombs force is a conservative force.
- External agent displaces the charge, then work
done by the field is equal to work done by
external agent causing the displacement. - (REMEMBER Work force x displacement)
- For an infinitesimal displacement ds, the work
done, dW, by the electric field on the charge is
F . ds qoE . ds.
5
Electric Potential Energy
- The potential energy of the charge-field system
is decreased by an amount dU ? qoE . ds -
dW - For a finite displacement of the charge from a
point A to a point B, the change in potential
energy of the system DU UB ? UA is
Integration performed along the path qo follows
as it moves from A to B (called path integral or
line integral). Force is conservative, this line
integral does not depend on the path taken
from A to B.
6
Conservative Force
E
B
qo
A
If the path between A to B does not make any
difference, why dont we just use the expression
DU -qoEd where d is the straight-line distance
between A and B ?
7
Electric Potential
- The Potential Energy per unit charge U/qo is
independent of the value of qo and has a unique
value at every point in an electric field. This
quantity is called the electric potential (or
simply the potential) V.
V U/qo
- Potential difference DV VB ? VA between any
two points A and B is
8
Electric Potential at an arbitrary point
- Electric potential at an arbitrary point in an
electric field equals the work required per unit
charge to bring a positive test charge from
infinity (where V 0) to that point.
- Electric potential at any point P is
S.I. unit J/C defined as a volt (V) and 1 V/m
1 N/C
Note that Vp represents the potential difference
DV between the point P and a point at infinity.
9
Potential Differences in Uniform E field
Example Uniform field along y axis (E parallel
to ds)
VB ? VA DV ?
E . ds
?
E ds
DV ?
E ds ? E d
- When the electric field E is directed downward,
point B is at a lower electric potential than
point A. A positive test charge that moves from
point A to point B loses electric potential
energy. - Electric field lines always point in the
direction of decreasing electric potential.
Pictures from Serway Beichner
10
Potential Energy in Uniform E field
Example Uniform field along y axis (E parallel
to ds) And suppose a test charge qo moves from A
to B. We have
DU qo DV ? qo E d
If qo is positive then DU is negative. i.e. a
positive charge loses electric potential energy
when it moves in the direction of the electric
field. This means electric field does work on a
positive charge when the charge moves in the
direction of the electric field. Release the
test charge at rest, it will accelerate downward,
gaining kinetic energy. As the charged particle
gains kinetic energy, it loses an equal amount of
potential energy.
Pictures from Serway Beichner
11
Potential Energy in Uniform E field
Example Uniform field along y axis (E parallel
to ds) And suppose a test charge qo moves from A
to B. We have
DU qo DV ? qo E d
If qo is negative then DU is positive and the
situation is reversed a negative charge gains
electric potential energy when it moves in the
direction of the electric field. The external
agent has to do work to cause this to happen.
Release the test charge at rest, it will
accelerate upward in the direction opposite to
electric field.
Pictures from Serway Beichner
12
Example (Potential Energy in Uniform Field)
S B 25.2 An ion accelerated through a potential
difference of 115 V experiences an increase in
kinetic energy of 7.37 x 10 17 J. Calculate the
charge on the ion.
qV 7.37x10-17 J , V115 V q 6.41x10-19 C
13
Potential Diff. In Uniform E field
Charged particle moves from A to B in uniform E
field.
q
DU qo DV - qo E . s ? qo Es cos q
Show that the potential diff. between path (1)
and (2) are the same as expected for a
conservative force field.
Pictures from Serway Beichner
14
Potential Diff. In Uniform E field (Path
independence)
Show that the potential difference between path
(1) and (2) are the same as expected for a
conservative force field.
path (1)
DV -
E . ds ? Es cos q
path (1)
path (2)
0 since E ds
DV -
E . ds - E . ds
path (2)
Same
DV ?
E . ds ? E h ? E s cos q
Pictures from Serway Beichner
15
Equipotential
VC VB ( same potential)
In fact, points along this line has the same
potential. We have an equipotential line.
Pictures from Serway Beichner
16
Equipotential Surfaces
The name equipotential surface is given to any
surface consisting of a continuous distribution
of points having the same electric potential.
No work is done in moving a test charge between
any two points on an equipotential surface. The
equipotential surfaces of a uniform electric
field consist of a family of planes that are all
perpendicular to the field.
17
Equipotential Surface
Equipotential Surfaces (dashed blue lines) and
electric field lines (orange lines) for (a) a
uniform electric field produced by infinite sheet
of charge, (b) a point charge, and (c) an
electric dipole. In all cases, the equipotential
surfaces are perpendicular to the electric field
lines at every point.
Pictures from Serway Beichner
18
Equipotential Surfaces
Rank the work done by the E field on a positively
charged particle that moves from (i) A to B (ii)
B to C (iii) C to D (iv) D to E.
Pictures from Serway Beichner
19
E-field between two parallel plates
Assume uniform field, and 3 mm plate separation E
VB VA / d 12 / 3.0x10-3 4000 V/m E
directed from A (ve) to B (?ve) A(ve) plate is
at higher potential than ve plate. Potential
difference between plates potential difference
between battery terminals because all points on a
conductor in equilibrium are at the same electric
potential no potential difference exists between
a terminal and any portion of the plate to which
it is connected.
Pictures from Serway Beichner
20
Motion of a proton in a uniform E field
- A proton is released from rest in a uniform E
field that has a magnitude of 8 x 104 V/m and is
directed along the positive x-axis. The proton
undergoes a displacement of 0.50 m in the
direction of E. - Find the change in electric potential between
points A and B. - Find the change in potential energy of the proton
for this displacement.
- DV ?Ed ? (8.0x104 V/m) (0.50m) ? 4.0x104 V
- DU q DV (1.6 x 10-19 C) (?4 .0x104 V) ?6.4
x 10-15 J - What is the significance of the negative sign?
- What is the speed of the proton?
Pictures from Serway Beichner
21
Example (Motion in Uniform Field)
S B 25.8 Suppose an electron is released from
rest in a uniform electric field whose magnitude
is 5.90 x 103 V/m. (a) Through what potential
difference will it have passed after moving 1.00
cm? (b) How fast will the electron be moving
after it has traveled 1.00 cm?
- (a) DV Ed (5.90 x 103 V/m)(0.0100 m) 59.0
V - (b) q DV mv2/2 ? v 4.55x106 m/s
22
Example (Motion in Uniform Field)
S B 25.13 On planet Tehar, the acceleration due
to gravity is the same as that on Earth but there
is also strong downward electric field with the
field being uniform close to the planets
surface. A 2.00 kg ball having a charge of 5.00
mC is thrown upward at a speed of 20.1 m/s and it
hits the ground after an interval of 4.10 s. What
is the potential difference between the starting
point and the top point of the trajectory?
(Tutorial)
23
Example (Motion in Uniform Field)
S B 25.14 An insulating rod having linear
charge density l 40.0 ?C/m and linear mass
density ? 0.100 kg/m is released from rest in a
uniform electric field E 100 V/m directed
perpendicular to the rod as shown. (a) Determine
the speed of the rod after it has traveled 2.00
m. (b) How does you answer to part (a) change if
the electric field is not perpendicular to the
rod ? (Ignore gravity)
Arbitrarily take V 0 at the initial point, so
at distance d downfield, where L is the rod
length
0.4 m/s
(b) same
Pictures from Serway Beichner
24
Electric Potential and Potential Energy due to
point charges
Consider isolated positive point charge q.
(i.e. E directed radially outward from the
charge) To find electric potential at a point
located at a distance r from the charge, start
with the general expression for potential
difference
Where A and B are two arbitrary points as shown.
E kq/r2 r, where r is a unit vector directed
from the charge toward the field point.
Pictures from Serway Beichner
25
Electric Potential and Potential Energy due to
point charges
We can express E . ds as
E . ds kq/r2 r . ds
The magnitude of r is 1, dot product r . ds ds
cos q, where q is the angle between r and ds .
ds cos q is the projection of ds onto r , thus
ds cos q dr.
VB-VA -
E . ds
-
kq/r2 dr
Pictures from Serway Beichner
26
Electric Potential and Potential Energy due to
point charges
VB-VA -
-
Er dr
kq/r2 dr
rB
kq
VB-VA
r
rA
1
1
VB-VA kq
rB
rA
Depends only on the coordinates and not on the
path.
Pictures from Serway Beichner
27
Electric Potential and Potential Energy due to
point charges
rA infinity (and VA 0), we have electric
potential created by a point charge at a distance
r from the charge given by
Points at same distance r from q have the same
potential V, i.e. the equipotential surfaces are
spherical and centered on the charge.
Pictures from Serway Beichner
28
Potential due to two or more charges
Superposition
q1
r1
q2
q5
r2
r5
P
r3
r4
q3
q4
where potential is taken to be zero at infinity
and ri is the distance from the point P to the
charge qi. Note that this is a scalar sum rather
than a vector sum.
29
Example
SB 25.18 A charge q is at the origin. A charge
2q is at x 2.00 m on the x axis. For what
finite value(s) of x is (a) the electric field
zero ? (b) the electric potential zero ?
x2 4.00x 4.00 0 (x4.83)(x?0.83)0
- x - 4.83 m
- (other root is not physically valid)
?x 0.667 m and x -2.00 m
30
Potential Energy of a system of two charges
V1 potential at a point P due to q1, external
agent must do work to bring a second charge q2
from infinity to P and this work q2V1.
Definition This work done is equal to the
potential energy U of the two-particle
system.
P
If two point charges are separated by a distance
r12, the potential energy of the pair of charges
is given by
Pictures from Serway Beichner
31
Potential Energy
Three point charges are fixed at the positions
shown. The potential energy of this system of
charges is given by
Pictures from Serway Beichner
32
Example (Potential Energy)
SB 25.23 What is the amount of work required to
assemble four identical point charges of
magnitude Q at the corners of a square of side s?
U 0 U12 (U13 U23) (U14 U24 U34)
s
s
s
s
Pictures from Serway Beichner
33
Example (Potential Energy)
SB 25.55 The charge distribution as shown is
referred to as a linear quadrupole. (a) What is
the potential at a point on the axis where x gt a?
(b) What happens when x gtgt a?
Pictures from Serway Beichner
34
Example (Potential Energy)
V ke Q
Pictures from Serway Beichner
35
Electric Potential and Potential Energy due to
point charges
Homework create an array of 2,3,4,5 charges and
construct the corresponding equipotential
contours and electric field lines distribution.
You may want to verify your results with results
from following webpage.
Click here to get to website that generate field
lines for your chosen charge distribution
36
Electric Field from Electric Potential
Electric field related to electric potential by
This means
dV ? E . ds
If electric field has only one component Ex, then
E . ds Ex dx. We have dV ? Ex dx or
37
Electric Field from Electric Potential
- Magnitude of E field in the direction of some
coordinate is equal to negative of the
derivative of the electric potential w.r.t. that
coordinate. - If the charge distribution creating the E-field
has spherical symmetry such that the volume
charge density depends only on the radial
distance r, then the electric field is radial.
In this case, and E . ds Er dr
38
Electric Field from Electric Potential
- For point charge V kq/r and we have E
kq/r2 - The potential changes only in the radial
direction, not in any direction perpendicular to
r. Thus V is a function only of r.
- Equipotential surfaces are a family of spheres
concentric with the spherically symmetric charge
distribution. - Equipotential surface perpendicular to field
lines.
Pictures from Serway Beichner
39
Electric Field from Electric Potential
- More general expression (in cartesian coordinate)
- More general expression (in spherical coordinate)
z
q
r
y
f
x
40
From general expression to simplified expression
- More general expression (in spherical coordinate)
If the potential V does no depends on the
coordinates q and f, then dV/dq 0 and dV/df
0, we have
41
Example Electric Potential due to a dipole
An electric dipole consists of two charges of
equal magnitude and opposite sign separated by a
distance 2a. The dipole is along the x-axis and
is centered at the origin. (a) Calculate the
electric potential at point P. (b) Calculate V
and Ex at a point far from the dipole. (c)
Calculate V and Ex if P is located anywhere
between the two charges.
(a)
q
q
2kqa
k
x- a
x a
x2 - a2
- x gtgt a, V 2kqa/x2 Ex - dV/dx 4kqa/x3
q
q
2kqx
(c)
k
a - x
x a
x2 - a2
-x2-a2
dV
d
2kq
Ex
x2 - a2 2
dx
dx
Pictures from Serway Beichner
42
Example (E -dV/dx)
SB 25.37 Over a certain region of space, the
electric potential is V 5x 3x2y 2yz2. Find
the expression for the x,y,z components of the
electric field over this region. What is the
magnitude of the field at the point P, which has
coordinates (1, 0, -2)m?
Ex - dV/dx
Ey - dV/dy
Ez - dV/dz
43
Electric Potential due to continuous charge
distribution
Calculate electric potential due to a continuous
charge distribution in two ways.
(1) Consider potential due to small charge
element dq, treating this element as a point
charge. The electric potential dV at some point
P due to dq is
dq
dV k
r
Summing up all elements ?
Pictures from Serway Beichner
44
Electric Potential due to continuous charge
distribution
Calculate electric potential due to a continuous
charge distribution in two ways.
(2) If electric field is already known from other
considerations (Gausss Law), we can calculate
the electric potential due to a continuous charge
distribution using
First determine DV between any two points and
then choose the electric potential V to be zero
at some convenient point.
45
Electric Potential due to a uniformly charged ring
(a) Find an expression for the electric potential
at point P located on the perpendicular central
axis of a uniformly charged ring of radius a and
total charge Q. (b) Find an expression for the
magnitude of the electric field at point P.
(a) Charge element dq is at a distance x2a2
from point P.
dq
dq
V k
k
r
x2 a2
And each element dq is at the same distance from
P, i.e.
k
kQ
dq
V
x2 a2
x2 a2
Pictures from Serway Beichner
46
Electric Potential due to a uniformly charged ring
(b) Use Ex - dV/dx
dV
d
-1/2
Ex -
(x2 a2 )
- kQ
dx
dx
-3/2
- kQ (- ½)
2x
(x2 a2 )
kQx
Ex
3/2
(x2 a2 )
What about Ey and Ez ? What is the electric
potential at the center of the ring? What is the
electric field at the center of the ring?
Pictures from Serway Beichner
47
Electric Potential due to a uniformly charged ring
kQx
Ex
3/2
(x2 a2 )
Flashback from topic l
Pictures from Serway Beichner
48
Electric Potential due to uniformly charged ring
Example 25 Q41 here Consider a ring of radius R
with the total charge Q spread uniformly over its
perimeter. What is the potential difference
between the point at the center of the ring and a
point on its axis a distance 2R from the center?
49
Electric Potential due to a uniformly charged disk
(a) Find an expression for the electric potential
at point P located on the perpendicular central
axis of a uniformly charged disk of radius a and
surface charge density s. (b) Find an expression
for the magnitude of the electric field at point
P.
(a) Divide into rings radius r and width dr and
surface area dA2pr dr
Pictures from Serway Beichner
50
Electric Potential due to a uniformly charged disk
(a) To find the potential, sum over all rings.
Integrate dV from r 0 to r a
a
2r dr
pks
V
x2 r2
0
1/2
2pks
(x2 a2 )
- x
V
Pictures from Serway Beichner
51
Electric Potential due to a uniformly charged disk
(b) Ex - dV/dx
Pictures from Serway Beichner
52
Electric Potential due to a uniformly charged disk
When you are really close to this disk, then it
is as if you are looking at an infinite plane of
charge, use above equation to deduce the electric
field. Is the result consistent with the result
obtained from our discussion using Gausss law ?
Pictures from Serway Beichner
53
Electric Potential due to uniformly charged
annulus
Calculate the electric potential at point P on
the axis of an annulus, which has uniform charge
density s.
V 2psk (x2b2)1/2 - (x2a2)1/2
Pictures from Serway Beichner
54
Electric Potential due to non-uniformly charged
disk
A disk of radius R has a non-uniform surface
charge density sCr where C is constant and r is
distance from the center of the disk as shown.
Find the potential at P.
dq sdA Cr(2prdr) and
Standard integral
V C(2pk) R(R2x2)1/2 x2ln(x/R (R2x2)1/2)
Pictures from Serway Beichner
55
Electric Potential due to a finite line of charge
A rod of length l located along the x axis has a
total charge Q and a uniform linear charge
density lQ/ l . Find the electric potential at a
point P located on the y axis at distance a
from the origin.
Length element dx with charge dq l dx
ldx
dV
k
k
x2 a2
l
Integrate dV over limits x0 to x l
Pictures from Serway Beichner
56
Electric Potential due to a finite line of charge
Integrate dV over limits x0 to x l
l
dx
V kl
x2 a2
0
Note l Q/ l and
Natural Log
l
dx
ln (x x2a2 )
x2 a2
We have
Pictures from Serway Beichner
57
Electric Potential due to a finite line of charge
A rod of length 2 l located along the x axis has
a total charge Q and a uniform linear charge
density lQ/2l . Find the electric potential at a
point P located on the y axis a distance a from
the origin.
How is this result consistent with the E field
for infinite line of charge obtained using
Gausss Law? (Homework)
Pictures from Serway Beichner
58
Electric Potential due to a finite line of charge
A rod of length L as shown lies along the x axis
with its left end at the origin and has a
non-uniform charge density l ax (where a is a
positive constant). (a) What are the units
of a? (b)
Calculate the electric potential at point A.
(c) Calculate the electric potential at
point B.
- C/m2
(b)
k? L d ln( 1 L/d)
(c) Homework for the brave.
Pictures from Serway Beichner
59
Electric Potential due to a uniformly charged
sphere
- An insulating solid sphere of radius R has a
uniform positive volume charge density and total
charge Q. - Find the electric potential at a point outside
the sphere, that is, r gt R. Take the potential
to be zero at r ?. - Find the potential of a point inside the sphere,
(r lt R).
In this case, it is easier to use electric field
obtained in our previous discussions and
determine the electric potential.
Pictures from Serway Beichner
60
Electric Potential due to a uniformly charged
sphere
Outside the sphere, we have
k Q
For r gt R
Er
r2
To obtain potential at B, we use
r
r
dr
VB -
Er dr - kQ
r2
?
Potential must be continuous at r R, gt
potential at surface
Pictures from Serway Beichner
61
Electric Potential due to a uniformly charged
sphere
Inside the sphere, we have
k Q
r
For r lt R
Er
R3
To obtain the potential difference at D, we use
r
r
k Q
k Q
VD - VC -
r dr
( R2 r2 )
Er dr -
2R3
R3
R
R
Since
To obtain the absolute value of the potential at
D, we add the potential at C to the potential
difference VD - VC
For r lt R
Check V for r R
Pictures from Serway Beichner
62
Electric Potential due to a uniformly charged
sphere
What are the magnitude of the electric field and
the electric potential at the center of the
sphere?
A plot of electric potential V versus distance r
from the center of a uniformly charged insulating
spheres of radius R. The curve for VD inside the
sphere is parabolic and joined smoothly with the
curve for VB outside the sphere, which is a
hyperbola. The potential has a maximum value Vo
at the center of the sphere. What are the
differences if the sphere is a conducting sphere?
Pictures from Serway Beichner
63
Electric Potential due to a charged conductor
Every point on the surface of a charged conductor
in equilibrium is at the same electric potential.
Consider two points A and B on the surface of a
charged conductor as shown. Along a surface path
connecting these points, E is always
perpendicular to the displacement ds therefore
E.ds 0. Using this result and
B
VB - VA -
E . ds 0
A
We find potential difference between A and B is
zero. This result applies to any two points on
the surface
Pictures from Serway Beichner
64
Electric Potential due to a charged conductor
What about inside the conductor?
Because the electric field is zero inside the
conductor, we conclude from the relationship E
?dV/dr that the electric potential is constant
everywhere inside the conductor and equal to its
value at the surface.
What is the work done in moving a positive charge
from the interior of a charged conductor to its
surface?
Pictures from Serway Beichner
65
Electric Potential due to a charged conductor
An arbitrarily shaped conductor carrying a
positive charge. When the conductor is in
electrostatic equilibrium, all of the charge
resides at the surface, E 0 inside the
conductor, and the direction of E just outside
the conductor is perpendicular to the surface.
The electric potential is constant inside the
conductor and is equal to the potential at the
surface.
Note from the spacing of the plus () signs that
the surface charge density is non-uniform.
Surface charge density is high where the radius
of curvature is small and the surface is convex.
And vice-versa. Because E field just outside the
conductor is proportional to the surface charge
density, we see that the electric field is large
near convex points having small radii of
curvature and reaches very high values at sharp
points. Can
you explain the shape of a lightning rod?
Pictures from Serway Beichner
66
Electric Potential due to Spherical Charged
Conductor
Outside the sphere, we have
k Q
For r gt R
Er
r2
To obtain potential at B, we use
r
r
dr
VB -
Er dr - kQ
r2
?
Pictures from Serway Beichner
67
Electric Potential due to a charged conductor
- The excess charge on a conducting sphere of
radius R is uniformly distributed on its surface.
- (b) Electric potential versus distance r from the
center of the charged conducting sphere. - (c) Electric field magnitude versus distance r
from the center of the charged conducting sphere.
Pictures from Serway Beichner
68
Example
25 Q 47 How many electrons should be removed
from an initially uncharged spherical conductor
of radius 0.300 m to produce a potential of 7.5
kV at the surface?
Substituting given values into V 7.50 x
103 V
1.56 x 1012 electrons
Pictures from Serway Beichner
69
Electric Potential due to a charged conductor
The electric field lines (in orange) around two
spherical conductors. The smaller sphere has a
net charge Q, and the larger one has zero net
charge. The blue curves are cross sections of
equipotential surfaces.
Note that the surface charge density is not
uniform
Pictures from Serway Beichner
70
Example Two connected Charged Conducting Spheres
Two spherical conductors of radii r1 and r2 are
separated by a distance much greater than the
radius of either sphere. The spheres are
connected by a conducting wire. The charges on
the spheres in equilibrium (the spheres are at
the SAME electric potential V) are q1 and q2
respectively, the they are uniformly charged.
Find the ratio of the magnitudes of the electric
fields at the surfaces of the spheres.
Connected ? both must have the same electric
potential.
V kq1/r1 kq2/r2 ? q1/r1 q2/r2
Sphere far apart ? charge uniform ? magnitude of
surface E given by
E1 kq1/r12 and E2 kq2/r22 we have E1/E2
r2/r1
Pictures from Serway Beichner
71
The Millikan Oil-Drop Experiment
At equilibrium qE mg gives q
http//www.ccmr.cornell.edu/muchomas/8.04/Lecs/le
c_Millikan/
http//www.mdclearhills.ab.ca/millikan/experiment.
html
Pictures from Serway Beichner
72
(No Transcript)
73
Coulombs law and the Principle of Superposition
constitute the physical input for
electrostatics. The force on any one charge due
to a collection of other charges is the vector
sum of the forces due to each individual charge.
74
- Problem Solving Strategies
- 1.Draw a clear diagram of the situation.
Be sure - to distinguish between the fixed external charges
and - the charges which the forces must be found. The
- Diagram should contain the coordinate axes for
- reference.
- 2.Electric force is a vector quantity
when many - forces are present the net force is a vector sum.
- 3.Search for symmetries in the
distribution of - charges that give rise to the electric force.
When - Symmetries are present, the net force along
certain - directions will be zero.
- Example Consider three point charges
- q1 q2 2.0 ?C and q3 -3 ?C which are placed
as - shown. Calculate the net force on q1 and q3.
75
(No Transcript)
76
The force on q1 is F1 F12 F13.
77
Similarly, F3 F31 F32
78
Electric Field. Electric field is defined as the
electric force per unit charge. The direction of
the field is taken to be the direction of the
force it would exert on a positive test charge.
The electric field is radically outward from a
positive charge and radically in toward a
negative point charge. The electric field can be
defined by measuring the magnitude and direction
of the electric force F on a test charge q0. A
small test charge is used so as not to interfere
with the field distribution of the other charges.
Thus we define the electric field as
The SI unit of electric field is NC-1.
79
Field Line Diagrams A convenient way to
visualize the electric field due to any
charge distribution is to draw a field line
diagram. At any point the field line has the
same direction as the electric field vector.
Electric field lines diverge from positive
charges and converge into negative charges.
Rules for constructing filed lines 1.
Field lines begin at positive charge and end
at negative
charge 2. The number of field
lines shown diverging from or
converging into a point is proportional to the
magnitude of the
charge. 3. Field lines are
spherically symmetric near a point
charge
80
4. 4. If the system has a net
charge, the field lines are
spherically symmetric at great distances 5.
5.Field lines never cross each other.
81
The Electric Dipole and their Electric Fields An
electric dipole consists of two charges q and
q, of equal magnitude but opposite sign, that
are separated by a distance L. From the
diagram, E Ex i (E1x E2x)i 2E1x i where
. But Hence I
I Define the product p qL as the
electric dipole moment. We make p qL a vector
by defining L to be directed from q to q. The
vector p points from the negative charge to the
positive charge.
I
82
The electric field decreases with r as 1/r3.
Finally,
.If r gtgtL, then
And Electric Dipole Field
83
Charge Distributions The simplest kind of charge
distribution is an isolated point charge (i.e.,
an amount of charge covering such a small region
of space that we need not be concerned about its
dimensions). When the finite size of the space
occupied by a collection of charges must be
considered, it is useful to consider the density
of charge. The word density is used is used in
three different ways. (rho) for the charge
per unit volume, the volume density (sigma)
for the charge per unit area, the area density
(lambda) for the charge per unit length, the
linear density Thus we can write
84
(No Transcript)
85
Find the electric field a distance z above the
midpoint of a straight line segment of length 2L
which carries a uniform line charge ?. (Model of
a transmission line). It is advantageous to chop
the line up into symmetrically placed pairs (at
? x). Here where q is the
total charge on the rod The horizontal
components of the two fields cancel. The net
field of the pair is Here ,
and x runs from 0 to L
86
How to evaluate this integral
87
Consider
and
Let
then
Substitute these into the integral
From diagram,
88
Hence
Hence Substitute the limits x 0 and x L to
get the desired answer.
For points far from the line ( z gtgt L), this
result simplifies to
89
Find the electric field a distance z above one
end of a straight line segment of length L,
which carries a uniform line charge ?. (Model of
a transmission line)
90
(No Transcript)
91
Net electric field E Ex Ez
For z gtgt L and
Find the electric field a distance z above the
center of a circular loop of
92
(No Transcript)
93
radius R, which carries a uniform line charge ?.
94
Here, where q is the total charge of the
loop Horizontal components cancel, leaving
(both constants)
But
95
Find the electric field a distance z above the
center of a flat circular disk of radius a,
which carries a uniform surface density,
. (Models an electrostatic microphone) A
typical element is a ring of radius r and
thickness dr, which has an area .
96
The charge density on the disk is
The charge on the element is
The electric field at P produced by this ring is
Since we have expressed a positive ring thickness
as dr, we sum the rings from the center toward
the edge. That is, the radius ranges from 0 to
a.
97
then du 2r dr, and
Let
98
So
When z ltlt a,
When z ltlt a,
which is the expected result for a point charge
located at the origin.
99
Gauss Law The net flux through any closed
surface is proportional to the net charge
enclosed by that surface, i.e.,
The total of the electric flux out of a closed
surface is equal to the charge enclosed divided
by the permittivity.
100
The electric flux through an area is defined as
the electric field multiplied by the area of the
surface projected in a plane perpendicular to
the field.
Gauss's Law is a general law applying to any
closed surface. It is an important tool since it
permits the assessment of the amount of enclosed
charge by mapping the field on a surface outside
the charge distribution. For geometries of
sufficient symmetry, it simplifies the
calculation of the electric field.
101
Gauss' Law, Integral Form
The area integral of the electric field over any
closed surface is equal to the net charge
enclosed in the surface divided by the
permittivity of space. Gauss' law is a form of
one of Maxwell's equations, the four fundamental
equations for electricity and magnetism.
Gauss' law permits the evaluation of the
electric field in many practical situations by
forming a symmetric Gaussian surface surrounding
a charge distribution and evaluating the electric
flux through that surface.
102
.
Flux and Gauss's Law Example Water Flow Suppose
water flows through a square surface of area A
with a uniform velocity
103
We want to define flux as the amount of water
that flows through the surface per second. First,
notice that the flux depends on the direction of
the velocity vector
relative to the surface.
104
Questions
Compare the water flux in the following three
situations. Rank the flux in cases (a), (b), and
(c), from largest to smallest
(a)
is perpendicular to the surface
105
(b)
is at a 45 degree angle to the surface
(c)
is parallel to the surface
106
If the biggest flux is 1, what is the relative
number corresponding to the flux in the other
two cases? Answer (a) 1 (b)
(c) 0
107
ELECTRICITY AND MAGNETISM P10D Coulombs
Law The force of attraction or repulsion
between two point charges q1 and q2 is directly
proportional to the product of their charges and
inversely proportional to the square of the
distance between them.
where F12 is the force exerted on point charge q1
by point charge q2 when they are separated by a
distance r12.
The unit vector is directed from q2 to q1
along the line between the two charges. The
constant is called the permitivity of
free space. In SI units where force is in
Newton's (N), distance in meters (m) and charge
in coulombs (C),
108
(No Transcript)
109
Coulombs law and the Principle of Superposition
constitute the physical input for
electrostatics. The force on any one charge due
to a collection of other charges is the vector
sum of the forces due to each individual charge.
110
- Problem Solving Strategies
- 1.Draw a clear diagram of the situation.
Be sure - to distinguish between the fixed external charges
and - the charges which the forces must be found. The
- Diagram should contain the coordinate axes for
- reference.
- 2.Electric force is a vector quantity
when many - forces are present the net force is a vector sum.
- 3.Search for symmetries in the
distribution of - charges that give rise to the electric force.
When - Symmetries are present, the net force along
certain - directions will be zero.
- Example Consider three point charges
- q1 q2 2.0 ?C and q3 -3 ?C which are placed
as - shown. Calculate the net force on q1 and q3.
111
(No Transcript)
112
The force on q1 is F1 F12 F13.
113
Similarly, F3 F31 F32
114
Electric Field. Electric field is defined as the
electric force per unit charge. The direction of
the field is taken to be the direction of the
force it would exert on a positive test charge.
The electric field is radically outward from a
positive charge and radically in toward a
negative point charge. The electric field can be
defined by measuring the magnitude and direction
of the electric force F on a test charge q0. A
small test charge is used so as not to interfere
with the field distribution of the other charges.
Thus we define the electric field as
The SI unit of electric field is NC-1.
115
Field Line Diagrams A convenient way to
visualize the electric field due to any
charge distribution is to draw a field line
diagram. At any point the field line has the
same direction as the electric field vector.
Electric field lines diverge from positive
charges and converge into negative charges.
Rules for constructing filed lines 1.
Field lines begin at positive charge and end
at negative
charge 2. The number of field
lines shown diverging from or
converging into a point is proportional to the
magnitude of the
charge. 3. Field lines are
spherically symmetric near a point
charge
116
4. 4. If the system has a net
charge, the field lines are
spherically symmetric at great distances 5.
5.Field lines never cross each other.
117
The Electric Dipole and their Electric Fields An
electric dipole consists of two charges q and
q, of equal magnitude but opposite sign, that
are separated by a distance L. From the
diagram, E Ex i (E1x E2x)i 2E1x i where
. But Hence I
I Define the product p qL as the
electric dipole moment. We make p qL a vector
by defining L to be directed from q to q. The
vector p points from the negative charge to the
positive charge.
I
118
The electric field decreases with r as 1/r3.
Finally,
.If r gtgtL, then
And Electric Dipole Field
119
Charge Distributions The simplest kind of charge
distribution is an isolated point charge (i.e.,
an amount of charge covering such a small region
of space that we need not be concerned about its
dimensions). When the finite size of the space
occupied by a collection of charges must be
considered, it is useful to consider the density
of charge. The word density is used is used in
three different ways. (rho) for the charge
per unit volume, the volume density (sigma)
for the charge per unit area, the area density
(lambda) for the charge per unit length, the
linear density Thus we can write
120
(No Transcript)
121
Find the electric field a distance z above the
midpoint of a straight line segment of length 2L
which carries a uniform line charge ?. (Model of
a transmission line). It is advantageous to chop
the line up into symmetrically placed pairs (at
? x). Here where q is the
total charge on the rod The horizontal
components of the two fields cancel. The net
field of the pair is Here ,
and x runs from 0 to L
122
How to evaluate this integral
123
Consider
and
Let
then
Substitute these into the integral
From diagram,
124
Hence
Hence Substitute the limits x 0 and x L to
get the desired answer.
For points far from the line ( z gtgt L), this
result simplifies to
125
Find the electric field a distance z above one
end of a straight line segment of length L,
which carries a uniform line charge ?. (Model of
a transmission line)
126
(No Transcript)
127
Net electric field E Ex Ez
For z gtgt L and
Find the electric field a distance z above the
center of a circular loop of
128
(No Transcript)
129
radius R, which carries a uniform line charge ?.
130
Here, where q is the total charge of the
loop Horizontal components cancel, leaving
(both constants)
But
131
Find the electric field a distance z above the
center of a flat circular disk of radius a,
which carries a uniform surface density,
. (Models an electrostatic microphone) A
typical element is a ring of radius r and
thickness dr, which has an area .
132
The charge density on the disk is
The charge on the element is
The electric field at P produced by this ring is
Since we have expressed a positive ring thickness
as dr, we sum the rings from the center toward
the edge. That is, the radius ranges from 0 to
a.
133
then du 2r dr, and
Let
134
So
When z ltlt a,
When z ltlt a,
135
Electric Flux The concept of electric flux is
useful in association with Gauss ' law. The
electric flux through a planar area is defined as
the electric field times the component of the
area perpendicular to The field. If the area is
not planar, then the evaluation of the
flux generally requires an area integral since
the angle will be continually changing.
136
When the area A is used in a vector operation
like this, it is understood that the magnitude
of the vector is equal to the area and the
direction of the vector is perpendicular to the
area. Gaussian Surface Part of the power of
Gauss' law in evaluating electric fields is
that it applies to any surface. It is often
convenient to construct an imaginary surface
called a Gaussian surface to take advantage of
the symmetry of the physical situation.
137
(No Transcript)
138
Conductor at Equilibrium
- The net electric charge of a conductor resides
entirely on - its surface. (The mutual repulsion of like
charges from - Coulomb's Law demands that the charges be as
far apart as - possible, hence on the surface of the
conductor.) - 2. The electric field inside the conductor is
zero. (Any net - electric field in the conductor would cause
charge to move - since it is abundant and mobile. This
violates the condition - of equilibrium net force 0.)
139
The external electric field at the surface of the
conductor is perpendicular to that surface. (If
there were a field component parallel to the
surface, it would cause mobile charge to move
along the surface, in violation of the assumption
of equilibrium.)
Electric Field Conductor Surface
140
The fact that the conductor is at equilibrium is
an important constraint in this problem. It
tells us that the field is perpendicular to the
surface, because otherwise it would exert a
force parallel to the surface and produce charge
motion. Likewise it tells us that the field in
the interior of the conductor is zero, since
otherwise charge would be moving and not at
equilibrium.
141
Examining the nature of the electric field near a
conducting surface is an important application of
Gauss' law. Considering a cylindrical Gaussian
surface oriented perpendicular to the surface, it
can be seen that the only contribution to the
electric flux is through the top of the Gaussian
surface. The flux is given by
and the electric field is simply
While strictly true only for an infinite
conductor, it tells us the limiting value as we
approach any conductor at equilibrium.
142
Gauss's Law for a Single Point Charge Gauss's Law
applies to any charge contribution, but let us
apply it now to the simplest case, that of a
single point charge q.
Construct a spherical Gaussian surface of radius
r around the charge q. Take a small area on the
Gaussian surface. The area vector dA points
radially outward, as does the electric field
vector at this point. Therefore, the electric
flux through this small area is
143
From the spherical symmetry, all of such small
area elements contribute equally to the total.
According to Gauss's Law,
because
q. Solving for the electric field gives
which is just Coulomb's Law!
144
A Uniformly Charged Solid Sphere An insulating
sphere of radius R has a total charge q uniformly
distributed throughout its volume. We want to
find the electric field everywhere, that is, at
an inside point r lt R, and also at an outside
point r gt R.
145
For the case where r lt R We construct a
spherical Gaussiansurface of radius r and apply
Gauss's Law.
We already know that for a spherically symmetric
case,
We have to be careful about qenc, though. qenc is
the net charge within the Gaussian surface, not
the total charge q of the entire sphere. For this
reason, we need to multiply the total charge by
the ratio of the volume of the Gaussian surface
to the volume of the entire sphere
146
Therefore,
147
The graph below is of the magnitude of the
electric field due to a uniformly charged sphere,
plotted as a function of distance from the
center of the sphere.
148
Electric Field for a Charged Sheet What is the
electric field due to a large, thin charged
sheet made of some no conducting material?
We construct a cylindrical Gaussian surface, and
place it symmetrically in the charged sheet as
shown in the above figure. Let the area of each
top surface of the \ cylinder be A, and the
surface charge density on the sheet be ?.
149
Applying Gauss's Law to this cylindrical Gaussian
surface gives
and we note qenc ?A.Therefore,
Electric Field between Capacitor Plates
Consider two large, parallel conducting plates,
one with a charge density ? and the other with
charge density - ?.
150
The two opposite charges are attracted to the
inside of the plates, as shown. We construct a
cylindrical Gaussian surface, with one base area
within a conducting plate and another base
between the plates. If the area of each base is
A, then we have
where E is the electric field at the base located
between the parallel plates, since E 0 on the
base inside the conductor plate. Then, we have
151
Electric Field Due to a Line Charge
Consider an infinitely long straight line charge
with linear charge density ?, which is in units
of Coulombs per meter.
Choose a cylinder because it mimics the symmetry
of the wire. We construct a cylindrical Gaussian
surface of radius r and length L. The electric
flux about this Gaussian surface is
152
Therefore,
Question
Find the electric field at all points due to a
long, solid cylinder of radius R and uniform
charge density ?.
Answer B
Begin by choosing an appropriate Gaussian
surface one that mimics the symmetry of the
case. Therefore, since we are concerned with a
long cylinder, we choose a Gaussian surface that
is also a long cylinder.To find the field
everywhere, we need to examine two cases when
the radius r of the Gaussian surface is less than
R, and when r gt R.
153
Case I r lt R We note that the electric field
will be constant everywhere on the cylindrical
Gaussian surface we also note that this surface
has a surface area of 2 ?rL. Thus, Gauss's Law
becomes
The uniform charge density of the cylinder is ?.
Therefore, the enclosed charge qenc is simply ?
times the volume enclosed by the Gaussian
surface, ?r2L. This gives us
Therefore, for r lt R,
154
Case II r gt R
Again, the electric field will be constant
everywhere on the cylindrical Gaussian surface,
and the surface area of that surface is still 2
?rL. With r gt R, the entire cylinder is enclosed,
so qenc is equal to the uniform charge density ?
times the volume of the charged cylinder, ?R2L.
This gives us
Therefore, for r gt R,
To summarize, here is a plot of the electric
field
versus the radius r of the Gaussian surface for
this problem
155
(No Transcript)
156
A Uniformly Charged Sphere Question
Use Gauss's Law to find the electric field
everywhere due to a uniformly charged insulator
shell, like the one shown below. The shell has a
total charge Q, which is uniformly distributed
throughout its volume.
157
(a) What is the charge on the inner surface of
the conductor?(b) What is the charge on the
outer surface of the conductor?(c) Use Gauss's
Law to find the electric field for radius r lt
a.(d) Use Gauss's Law to find the electric field
for radius a lt r lt b.(e) Use Gauss's Law to find
the electric field for radius r gt b.
Answer
We need to look at this problem in three parts
one, for when
the radius for when a lt r lt b, and
for when
.
No charge is enclosed in this case that is, qenc
0. Therefore,
the flux through the Gaussian surface must be
zero, and so the electric field
0 everywhere in this region.
158
Here, the charge enclosed is found by multiplying
the total charge Q by the ratio of the volume of
charge enclosed by the Gaussian surface to the
volume of the entire charged shell
We recall that
and as the electric field will be constant
everywhere on the spherical Gaussian surface, we
can substitute as follows
159
Therefore,
III.
Here, qenc is Q, so we have
Again, since the electric field will be constant
at every point on the spherical Gaussian
surface, we have
160
Which becomes
To summarize our findings, here is a plot of the
electric field as a function of the radius of the
Gaussian surface
161
Potential Energy, Work, and the Electric Field
The potential energy difference can be defined as
the negative of the work
done by a conservative force F on an object moved
from point A to point B.
where dl is a small element of the path from A to
B and l is a vector from A to B.
162
Potential Difference
The electric potential difference from point A to
point B is the potential energy change per unit
charge in moving from A to B.
In the case of a uniform field,
The Volt and the Electron Volt
The unit of potential difference is the volt (V).
1 V 1J/C. To say that a car has a 12V battery
means that the battery does 12 J of work on every
coulomb the moves between its two terminals.
163
The electron volt (eV) is the energy gained by a
particle carrying one elementary charge when it
moves through a potential difference of one
volt. 1eV 1.602 ? 10-19 J.
Calculating Potential Difference
The potential of a point charge. The electric
field of a point charge q is given by
Consider two points A and B at distances rA and
rB from a positive point charge.
164
The distance between them is rB - rA but we
cannot just multiply this distance by the
electric field because the field varies with
position. Instead we must integrate as follows
165
What if the points do not lie on the same radial
line?
The potential difference is independent of path
and one path between A and B consists of a
radial segment and a circular arc. Since E is
perpendicular to the arc, it takes no work to
move a charge along the arc. The potential
difference
therefore arises only from the radial segment.
Defining the potential to be zero at some point
allows us to speak of the potential at a
point, meaning the potential difference from
the reference point to the point in question.
For isolated point charges, a convenient zero is
infinitely far from the charge then the
potential at an arbitrary point a distance r
from the point q is
166
Potential difference in the field of a line charge
We know from before that
for field of a line charge
Hence
167
Finding potential differences using superposition
where the ris are the distances from each of the
charges to the point P.
168
Electric potential is a scalar quantity, so the
sum above is a scalar sum, and there is no need
to consider angles or vector components
Continuous charge distributions
We can calculate the potential of a continuous
charge distribution by considering it to be made
up of infinitely many infinitesimal charge
elements dq. Each acts like a point charge and
therefore contributes to the potential at some
point P an amount dV given by
where the zero potential is at infinity. The
potential at P is the sum of all the
contributions dV from all the charge elements.
169
A charged ring
A total charge Q is uniformly distributed over a
thin ring of radius a. What is the potential on
the axis of the ring?
At the center of the ring,
170
A charged disk
A charged disk of radius a carries a total charge
Q distributed uniformly over its surface. What
is the potential at a point P on the disks
axis, a distance x from the disk?
Divide the disk into charge elements dq. If a
ring shaped element has charge dq and radius r,
then from above,
Then
171
We must relate r and dq.
Unwinding the ring gives a strip of area
The surface density
is the total charge divided by the disks area
is
.
The charge dq on our infinitesimal ring of area
172
then