Quantum Mechanics: An Introduction - PowerPoint PPT Presentation
Title:
Quantum Mechanics: An Introduction
Description:
Quantum Mechanics: An Introduction Electromagnetic radiation exhibits wavelike properties which can be depicted in the following representation below: – PowerPoint PPT presentation
Number of Views:225
Avg rating:3.0/5.0
Title: Quantum Mechanics: An Introduction
1
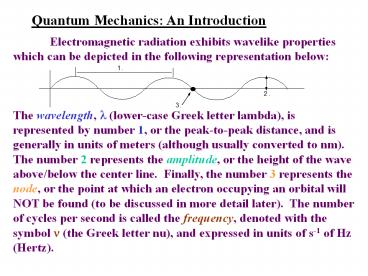
Quantum Mechanics An Introduction Electromagnet
ic radiation exhibits wavelike properties which
can be depicted in the following representation
below The wavelength, ? (lower-case Greek
letter lambda), is represented by number 1, or
the peak-to-peak distance, and is generally in
units of meters (although usually converted to
nm). The number 2 represents the amplitude, or
the height of the wave above/below the center
line. Finally, the number 3 represents the node,
or the point at which an electron occupying an
orbital will NOT be found (to be discussed in
more detail later). The number of cycles per
second is called the frequency, denoted with the
symbol ? (the Greek letter nu), and expressed in
units of s-1 of Hz (Hertz).
2
Both light and waves can be characterized by
wavelength and frequency, and all electromagnetic
radiation moves at the speed of light (c). Max
Planck first analyzed data from the emission of
light from hot, glowing solids. He observed that
the color of the solids varied with temperature.
Furthermore, Planck suggested that a relationship
exists between energy of atoms in the solid and
wavelength. This led to the following equation
?? c
3
?? c This equation has multiple
interpretations. If the wavelength is long,
there will be fewer cycles of the wave passing a
point per second thus, the frequency will be
low. Conversely, for a wave to have a high
frequency, the distance between the peaks of the
wave must be small (short wavelength). In
summary, an inverse relationship exists between
frequency and wavelength of electromagnetic
radiation as the speed of light is always
constant.
4
Energy can be released (or absorbed) by atoms in
discreet chunks (also known as quantum or
photons) of some minimum size that is, energy is
quantized and emitted/absorbed in whole number
units of h?. Collectively, we say that E nh?
nhc/? where n the quantum number, h
Plancks constant (6.626 x 10-34 J s), ?
frequency, ? wavelength, and c speed of light
(3.0 x 108 m/s). Energy (E) is generally
expressed in units of Joules (J), where this
actually implies J/atom.
5
Electromagnetic Spectrum Electromagnetic waves
are produced by a combination of electrical and
magnetic fields which are at right angles to one
another (i.e. perpendicular). The various types
of waves include radiowaves, microwaves,
infrared, visible light, ultraviolet, x-rays,
cosmic rays, and gamma rays. All electromagnetic
waves travel at the speed of light they differ
from one another by their corresponding
wavelengths and frequencies. Increasing
Frequency (?) and Energy (E) Radio Micro
IR Visible UV X-ray Cosmic
Gamma Increasing Wavelength (?)
6
LIGHT ELECTROMAGNETIC RADIATION
VISIBLE LIGHT IS ORDERED AS R O
Y G B
I V red orange yellow
green blue indigo violet
700nm 400nm low energy high
energy long wavelength short wavelength
Example A radiation source has a frequency of
2.35 x 1014 hertz, what is the wavelength
energy associated with this light then identify
the type of radiation. How much energy what
frequency is associated with a wavelength of
488.6 nm? Identify this radiation.
7
RADIATION SOURCE TYPE OF INTERACTION WITH
MATTER X - RAY energy transfer results in an
inner shell electron being ejected causing
other electrons to cascade (emit) down to
lower levels. High energy short
wavelength. UV/Vis energy is transferred to
outershell electron resulting in the
excitation of that electron which eventually
cascades back down to lower(ground) energy
state. Ir energy is transferred to molecule
causing the molecule to vibrate sometimes
even lower energy is emitted back out or the
energy is lost as heat. Microwave energy
is transferred to molecule causing the molecule
to vibrate and rotate the higher initial
energy is lost as vibrational and rotational
energy and as heat. Low energy and long
wavelength.
8
Workshop on EM Radiation 1. Yellow light
exhibits a wavelength of approximately 570 nm.
Determine the frequency of this light and the
total energy of the photon being emitted in units
of J and kJ/mol. 2. When an electron beam
strikes a block of copper, x-rays with a
frequency of 2.0 x 1018 Hz are emitted. How much
energy is emitted at this wavelength by (A) an
excited copper atom when it generates an x-ray
photon (B) 1.00 mol of excited copper atoms and
(C) 1.00 g of copper atoms? 3. A minimum energy
of 495 kJ/mol is required to break the
oxygen-oxygen bond in O2. What is the longest
wavelength of radiation that possesses the
necessary energy to break the bond? 4. Arrange
the following types of photons of radiation in
order of increasing speed infrared radiation,
visible light, x-rays, and microwaves. Which
form of radiation possesses the longest
wavelength? Which form of radiation possesses
the largest amount of energy per photon?
9
Photoelectric Effect Consider incident radiation
which consists of a stream of photons of energy
h?. Once these particles strike the surface of a
metal, the energy is absorbed by an electron. If
the energy of the photon is less than the energy
required to remove an electron from the metal,
then an electron will NOT be ejected. Moreover,
the energy required to remove an electron from
the surface of a metal is called the work
function (?) of the metal. However, if the
energy of the photon is greater than the work
function, then an electron is ejected with a
kinetic energy equal to the difference between
the energy of the incoming photon and the work
function. That is, KEelectron ½ mv2 h? -
h?o where m mass, v velocity, h Plancks
constant, and ? frequency.
10
Photoelectric Effect These results, known as the
photoelectric effect, can be summarized alongside
with Einsteins theory 1. An electron can be
driven out of the metal only if it receives at
least a certain minimum energy, ?, from the
photon during the collision. Therefore, the
frequency of the radiation must have a certain
minimum value if electrons are to be ejected.
This minimum frequency depends on the work
function and hence on the identity of the
metal. 2. Provided a photon has enough energy, a
collision results in the immediate ejection of an
electron. 3. The kinetic energy of the ejected
electron from the metal increases linearly with
the frequency of the incident radiation according
to the equation ½ mv2 h? - h?o.
11
Lecture questions on EM Radiation the
Photoelectric Effect. 1. Yellow light is given
off by a sodium vapor lamp used for public
lighting. If the light has a wavelength of 589
nm, what is the frequency of this radiation?
What is its energy? 2. What type of radiation
is involved if the wavelength is 10 m? What type
of radiation is involved if the frequency is 5 x
1016 Hz? What wavelength is this? 3. Calculate
the ionization energy of an Iridium atom, given
that radiation of wavelength 76.2 nm produces
electrons with a speed of 2980 km/s.
12
Workshop on Photoelectric Effect 1. Calculate
the ionization energy of a rubidium atom, given
that radiation of wavelength 58.4 nm produces
electrons with a speed of 2450 km/s. 2. The
energy required for the ionization of a certain
atom is 3.44 x 10-18 J. The absorption of a
photon of unknown wavelength ionizes the atom and
ejects an electron with velocity 1.03 x 108 m/s.
Calculate the wavelength of the incident of
radiation. 3. The ionization energy of gold is
890.1 kJ/mol. Is light with a wavelength of 225
nm capable of ionizing a gold atom in the gas
phase?
13
de Broglie Relation and the Wave-Particle Duality
of Matter If electromagnetic radiation has a
dual character, could it be that matter, which
has been regarded as consisting of particles,
also have wavelike properties? On the basis of
theoretical observations, Louis de Broglie
suggested that all particles should be regarded
as having wavelike properties. Therefore, it was
proposed that
? h/p h/mv where ? wavelength, h
Plancks constant, p linear momentum (in units
of kg m/s), m mass (kg), and v velocity
(m/s). BE CAREFUL v is velocity and NOT
frequency (?). Beginning chemistry students
often confuse these symbols! Example If an
electron travels at a velocity of 1.000 x 107 m/s
and has a mass of 9.109 x 10-28 g, what is its
wavelength?
14
Heisenberg Uncertainty Principle Heisenberg
determined that there is a fundamental limitation
to just how precisely one can know both the
position and the momentum of a particle at a
given time. That is, ?x ?
?p gt h/4? where x the location of particle, p
linear momentum, and h Plancks constant.
This relationship means that the more precisely
we know a particles motion, the less precisely
we can know its momentum, and vice versa. The
uncertainty principle implies that we cannot know
the exact path of the electron as it moves around
the nucleus. It is therefore not appropriate to
assume that the electron is moving around the
nucleus in a well-defined orbit. Example An
electron with a mass of 9.109 x 10-31 kg is known
to have an uncertainty of 1 pm in its position.
Determine the uncertainty in its speed.
15
NIELS BOHR (1885-1962) A MODEL for THE HYDROGEN
ATOM
Bohr built upon Plancks Einsteins ideas about
quantized energy. States 1. The hydrogen atom
has only specific allowable energy levels
(quantized). 2. The atom does not radiate energy
while within an energy level. 3. Electrons
transition to different energy levels only by
absorbing or emitting a photon whose energy
equals the difference in energy between the
levels. Ephoton Efinal - Einitial
hn Limitations 1. Failed to predict the
spectrum for other atoms. (does not take into
account additional nucleus-electron attractions
and electron- electron repulsion) 2. Electrons
do not move in orbits
16
LINE SPECTRUM
Bohrs model was an attempt to explain how light
was emitted when an element was vaporized and
then thermally or electrically excited (the flame
test or neon sign). Through experimentation it
was discovered that each element has a unique
line spectrum with specific wavelengths that can
be used to identify it. RYDBERG EQUATION An
empirical equation used to predict the position
and wavelength of the lines in a given series in
a specific region of the EM spectrum. 1
R (1 - 1) l n2
n2 R Rydberg constant 1.096776 x 107 m-1
17
BOHRS MODEL RYDBERG Combined 1913
RH 2.179 x 10-18 J - Rydberg
constant The electron in the atom occupies
specific energy levels (hn, 2hn, 3hn, etc.) This
is called QUANTIZATION E RH
n2 The electron may undergo a
transition from one energy level to another.
Remember that the energy of emitted photonhvEi
- Ef hv RH (1 - 1)
ni2 nf2 1 RH (1
- 1) l hc ni2 nf2 These equations
can be used to determine the l or v of the
hydrogen atom.
18
The problem with classical physics of the time
was that an electron orbiting a nucleus would
lose energy eventually collapse into the
nucleus. In Bohrs model, an electron can travel
around a nucleus without radiating energy.
Furthermore, an electron in a given orbit has a
certain definite amount of energy. The only way
an electron can lose energy is by dropping from
one energy level to a lower one. When this
happens, the atom emits a photon of radiation
corresponding to the difference in energy levels.
However, electrons in higher levels cannot drop
to a lower level if that level is filled.
Because no electrons can move to a lower level,
none of them can lose energy. The atom is
energetically stable and is said to be in its
ground state. Atoms can absorb energy from an
outside source (such as heat from a flame or
electrical energy from a source of voltage),
causing one or more of the electrons within the
atom to move to higher energy levels. When
electrons are moved to these higher levels, the
atom is said to be in an excited state. However,
the atom is energetically unstable, so it will
not remain in an excited state for a long period
of time. Eventually, electrons return to lower
levels. As they do so, energy is given off in
the form of quanta.
19
ENERGY STATES OF THE HYDROGEN ATOM
When an electron, in its ground state, absorbs
energy from a photon (called absorption), that
electron is promoted to a higher energy level
(called the excited state). Emission is when an
electron in an excited state loses energy and
returns to a lower energy level.
E -2.18 x 10-18 J (Z2/n2) E
the energy of the atom (derived from classical
physics) Z the charge of the nucleus n the
energy level To find the energy difference
between any two levels and predict the spectral
lines for the hydrogen atom
?E hn hc l ?E Ef
- Ei -2.18 x 10-18 J (nf-2 - ni-2)
R -2.18 x 10-18
J/(hc)
20
If ni ? nf - energy is emitted ________es th
e electron jumps from a higher ?
energy level down to a lower
energy ________gs level If ni ? nf
- energy is absorbed ________es the electron
jumps from a lower ? energy level to a
higher one. ________gs Example Calculate the
wavelength of light that corresponds to the
transition of the electron from n 4 to n 2
state of the hydrogen atom. Is the light
emitted or absorbed? What color is it?
21
Bohr Model Revisited Bohr proposed a model that
included the idea that the electron in a hydrogen
atom moves around the nucleus only in certain
allowed circular orbits. Furthermore, Bohr
concluded the following as applied to the
bright-line spectrum of hydrogen 1. Hydrogen
atoms exist in only specified energy states given
by the Rydberg energy equation
E -2.178 x 10-18 J
(Z2/nfinal2 - Z2/ninitial2) where E Energy
(J), Z atomic number, and n orbital level.
2. Hydrogen atoms can absorb only certain
amounts of energy, and no others. 3. When
excited hydrogen atoms lose energy, they lose
only certain amounts of energy, emitted as
photons. 4. The different photons given off by
hydrogen atoms produce the color lines seen in
the bright-line spectrum of hydrogen. The
greater the energy lost by the atom, the greater
the energy of the photon.
22
Workshop on Bohrs Model 1. Determine the
energy of the line in the spectrum of hydrogen
that represents the movement of an electron from
a Bohr orbit with n 6 to n 4. 2. Determine
the wavelength of light that must be absorbed by
a hydrogen atom in its ground state to excite it
to the n 2 orbit. NOTE At first, Bohrs model
appeared to be very promising. The energy levels
calculated by Bohr closely agreed with the values
obtained from the hydrogen emission spectrum.
However, when Bohrs model was applied to atoms
other than hydrogen, it did not work at all. It
was therefore concluded that Bohrs model is
fundamentally incorrect for atoms with more than
one electron.
23
Schrödinger Equation Erwin Schrödinger proposed
the theory of quantum mechanics which suggested
that an electron (or any other particle)
exhibiting wavelike properties should be
described by a mathematical equation called a
wavefunction (denoted by the Greek letter psi,
?). Specifically, he developed a mathematical
formalism to describe the hydrogen atom as a
wave. This equation (which involves differential
calculus!) gives the probability of finding an
electron at some point in a three-dimensional
space at any given instant but offers no
information about the path the electron follows
(recall Heisenberg). The region in space where
there is a probability of finding an electron is
known as an orbital, in the Schrödinger
equation, the wavefunction is used to calculate
the probability of finding an electron in space.
24
QUANTUM MECHANICS Erwin Schrodinger (1887 -
1961) Schrodinger formulated the theory of wave
mechanics a description of the behavior of the
tiny particles that make up matter in terms of
waves. His wave equation describes the behavior
of electrons in atoms. H Y E Y Y is the wave
function/atomic orbital a mathematical
description of the motion of the electrons
matter-wave in terms of position time. H is
the Hamiltonian operator E is the energy of the
atom d2 Y/dx2 d2Y/dy2 d2Y/dz2
(8p2me/h2)E-V(x,y,z) Y(x,y,z) Y2 is the
probability of an electron being within a volume.
25
Quantum Mechanics
- In quantum mechanics, the electrons occupy
specific energy levels (as in Bohr's model) but
they also exist within specific probability
volumes called orbitals with specific
orientations in space. The electrons within each
orbital has a distinct spin. - n The principle quantum number
- Describes the possible energy levels and
pictorially it describes the orbital size. - n 1, 2, 3. where an orbital with the value of
2 is larger than an orbital with the value of 1.
2s
1s
26
Quantum Mechanics
- l angular momentum quantum number
- Describes the "shape" of the orbital and can have
values from 0 to n - 1 for each n. - l (n-1) to 0 (2 l 1 subshells)
- orbital designation s p d f
- shape
- ml magnetic quantum number
- Related to the orientation of an orbital in space
relative to the other orbitals with the same l
quantum numbers. It can have values between l
and - l . - ms spin quantum number
- An electron has either 1/2 or -1/2 spin values
sometimes referred to as spin up and spin down.
Too hard to draw see text
27
Quantum Mechanics and the Periodic Table 1.
Principle Quantum Number (n) specifies the
energy level of an electron and labels the shell
of an atom. Represented by the period number. 2.
Angular Momentum Quantum Number (l) specifies
the subshell of a given shell in an atom and
determines the shape of the orbitals in the
subshell.
Period 1 2 3 4 5 6 7
28
3. Magnetic Quantum Number (ml) identifies the
individual orbitals of a subshell of an atom and
determines the orientation in space 4a. Spin
Quantum Number (ms) distinguishes the two spin
states of an electron n
l Orbital Designation ml of orbital 1 2
3 4 4b. Pauli Exclusion Principle No two
electrons can have the same four sets of
identical quantum numbers. Moreover, when two
electrons (and NO MORE THAN TWO!) occupy the same
given orbital, their spins must be different.
This is due to the spin number.
29
Lecture Questions on Quantum Mechanics 1.
Determine the quantum numbers associated with the
following energy levels a) n2 b) n 3 n
5 2. Determine the sublevel names quantum
numbers a) n 3, l 2 b) n 4, l 2 c) n
2, l 2 3. What is the maximum number of
electrons in an atom that can have these quantum
numbers? A. n 3 B. n 2, l 0, ml 0 C. n
5, ms ½ D. n 2, l 1
30
Workshop on quantum numbers 1. How many
subshells are there for n 6? How many total
orbitals are there in the shell with n 6? 2.
Write the subshell notation and the number of
electrons that can have the following quantum
numbers if all the orbitals of that subshell are
filled A. n 3 l 2 B. n 5 l 0 C. n
7 l 1 D. n 4 l 3 3. How many electrons
can have the following quantum numbers in an
atom A. n 3, l 1 B. n 5, l 3, ml
-1 C. n 2, l 1, ml 0 4. Which of the
following sets of quantum numbers n, l, ml, ms
are allowed and which are not? For the sets of
quantum numbers that are incorrect, state what is
wrong. A. 2, 2, -1, -½ B. 6, 0, 0, ½ C. 5,
4, 5, ½